Friday Sept. 7, 2007
The Practice Quiz was returned in class today. You'll find
answers to the quiz questions here.
The first optional assignment is due
one week from today.
There is also a new reading assignment.
We will
sometimes "beat material to death" in this class. Here's an
example: we worked through the following figure in great detail in
class.
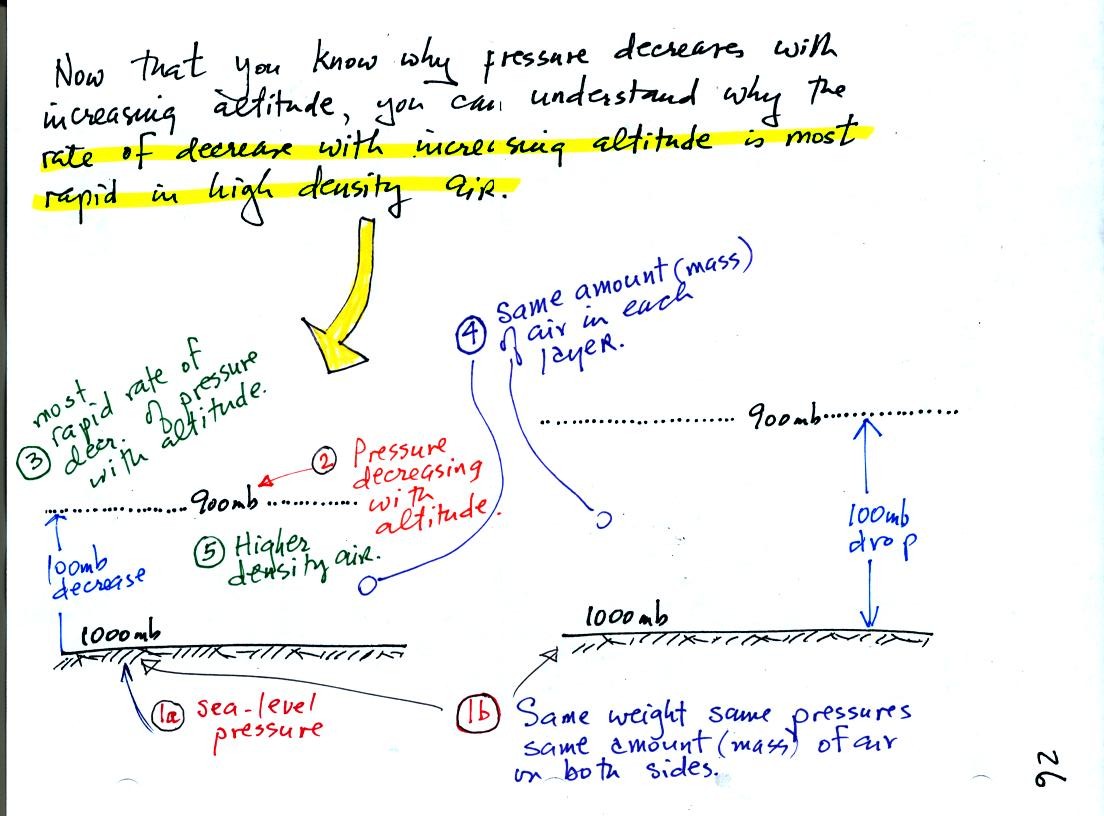
Point 1a - 1000 mb is a reasonable sea level pressure value.
Point 1b - The fact that the pressures are equal at the bottoms of both
pictures means that the weight of the atmosphere at the bottom of the
picture on the left is the same as the weight of the atmosphere at the
bottom of the picture at right. The only way this can be true is
if there is the same total amount (mass) of air in both cases.
Point 2 - Moving upward from the ground we find that pressure decreases
to 900 mb at the level of the dotted line in the picture at left.
This is what you expect, pressure decreases with increasing
altitude.
The pressure decreases to 900 mb in the picture at right, but you have
to go to higher altitude before this occurs.
Point 3 - The most rapid rate of pressure decrease with increasing
altitude is occurring in the picture at left.
Point 4 - Since there is a 100 mb drop in the layer at left and in the
layer at right, both layers must contain the same amount (mass) of air.
Point 5 - The air in the picture at left is squeezed into a thinner
layer than in the picture at right. The air density in the left
layer is higher than in the layer at right.
We used and analyzed this picture to prove to ourselves that the rate of pressure decrease
with altitude is higher in dense air than in lower
density air.
This is a fairly subtle but important concept. We will use this
concept later in the semester when we try to understand the
intensification of hurricanes.
We didn't
work through the following figures at all (except for filling in the
box at the bottom of p. 28 with the English and Metric units for mass
and weight).
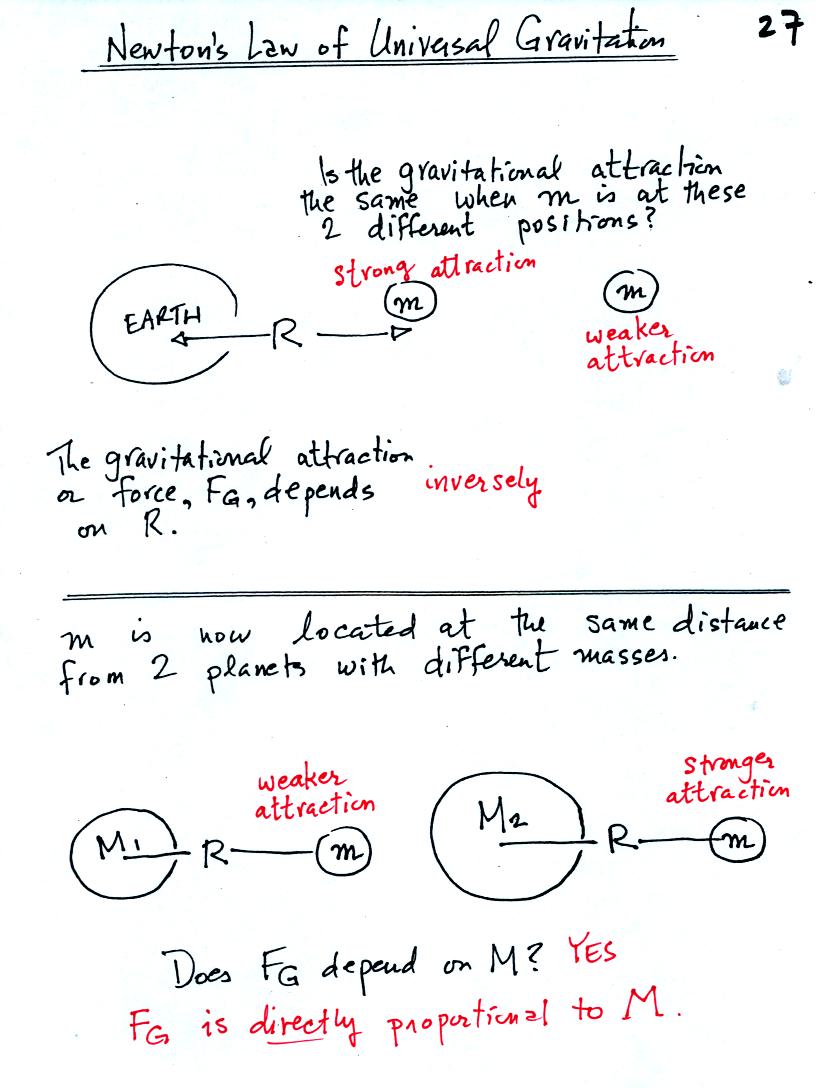
The gravitational attraction between two objects depends
first of
all
on the distance separating the objects. The gravitational
force becomes weaker the further away the two objects are from each
other. In the bottom
picture above and the top figure below we see that the attractive force
also depends on the masses of the two objects.
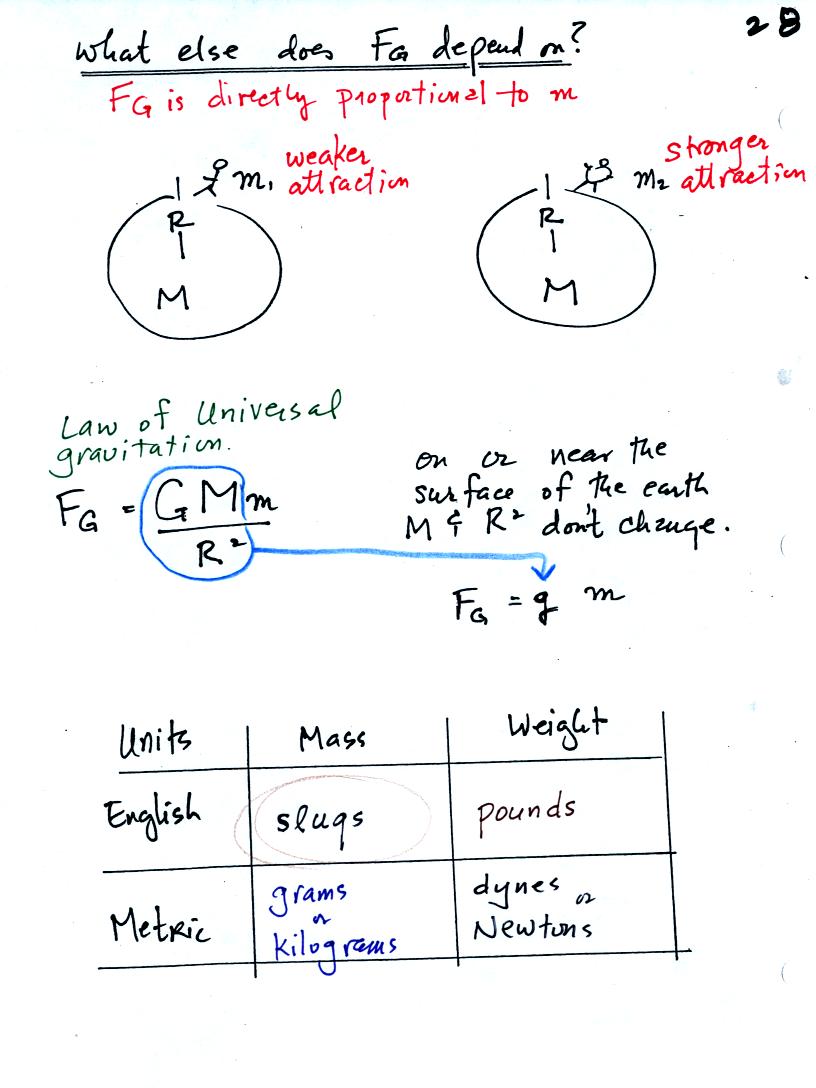
The complete formula is shown in the middle of the page
above. G
is a constant. On the surface of the earth G, M, and R don't
change. The gravitational acceleration, g, is just G times Mearth
divided by ( Rearth )2
. To determine the weight (on the earth's surface) of an object
with mass m you simply multiply m x g.
Down at the bottom of the page are the Metric and English units of mass
and weight.
Here's another page from the
photocopied Class Notes that we didn't cover in class. The
weight of a person on the earth and the moon is calculated in English
and metric units.
The mass of a person would be the same on the earth and on the moon.
The weight of a person depends on the person's mass and on the strength
of gravity (the acceleration of gravity term, the g variable below).
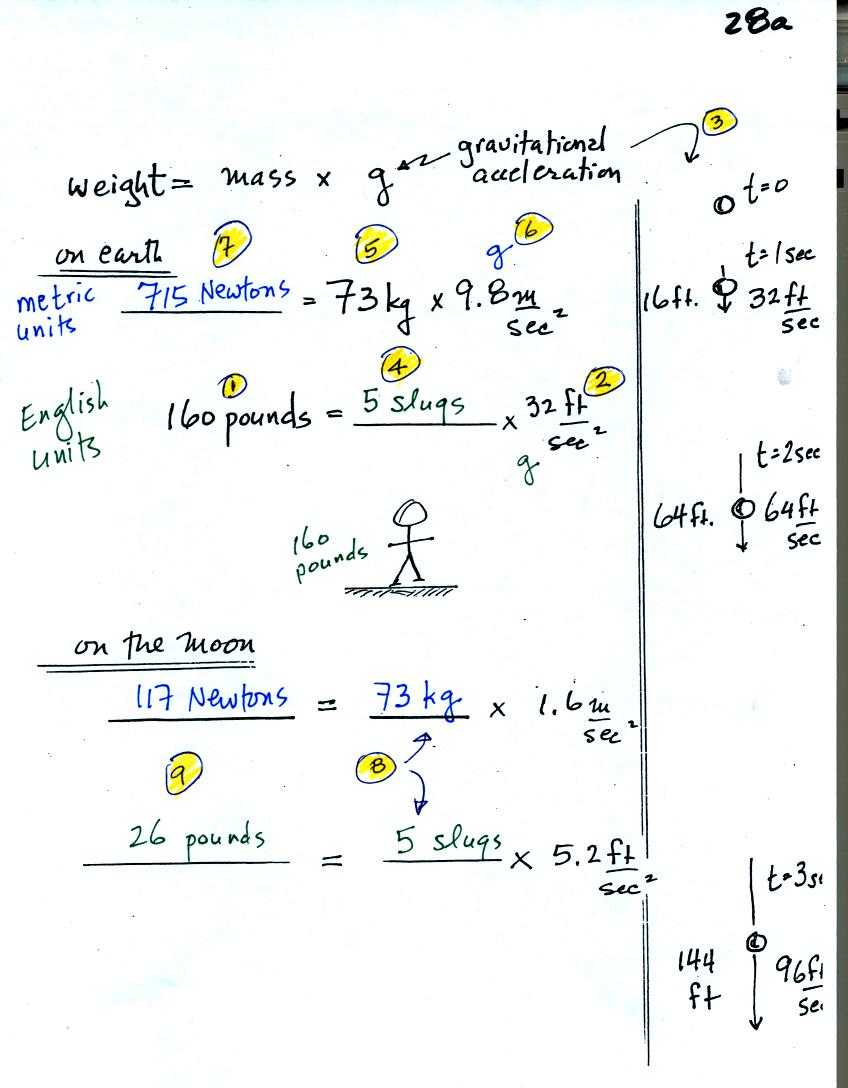
(1) The course instructor weighs about 160 pounds. In
(2) we see that the
gravitational acceleration is 32 ft/sec2 in English units. The
meaning of this value is shown in (3). Gravity will cause a
falling object to fall 32 ft/sec faster with every second it continues
to fall. Dividing the instructor's weight by the gravitation
acceleration in (4) we obtain the instructor's mass, 5 slugs, in
English units.
In metric units, the instructor has a mass of 73 kilograms (5).
The gravitation acceleration is 9.8 m/sec (6). Multiplying these
two values, in (7), we find that the instructor weighs 715 Newtons.
On the moon, the mass stays the same. Gravity is weaker, so the
value of g is smaller. The instructor would weigh quite a bit
less (117 Newtons or 26 pounds) on the moon compared to the earth.
Here's
another topic that we'll beat into submission, trying to understand how
a mercury barometer works (it's used to measure atmospheric
pressure). You'll find most of what follows on p. 29 in the
photocopied Class Notes.
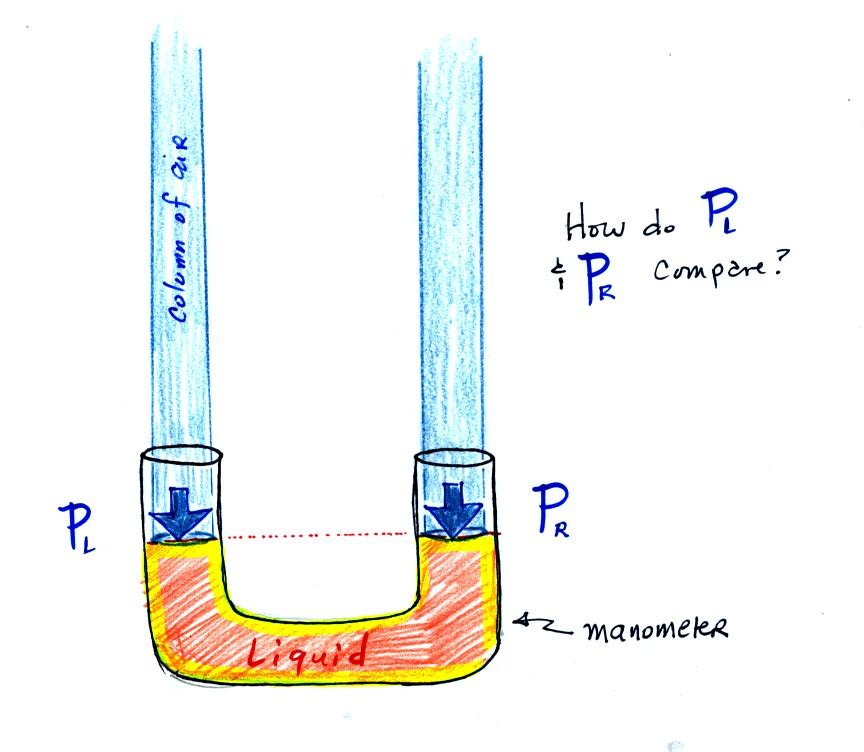
The instrument above ( a u-shaped glass tube filled with a
liquid of some kind) is a manometer and can be used to measure pressure
difference. The
two ends of the tube are open so that air can get inside and air
pressure can press on the liquid. Given that the liquid levels on
the two sides of the manometer
are equal, what could you about PL and PR?
The liquid can slosh back and
forth just like the pans on a balance can move up and down. A
manometer really behaves just like a pan balance. 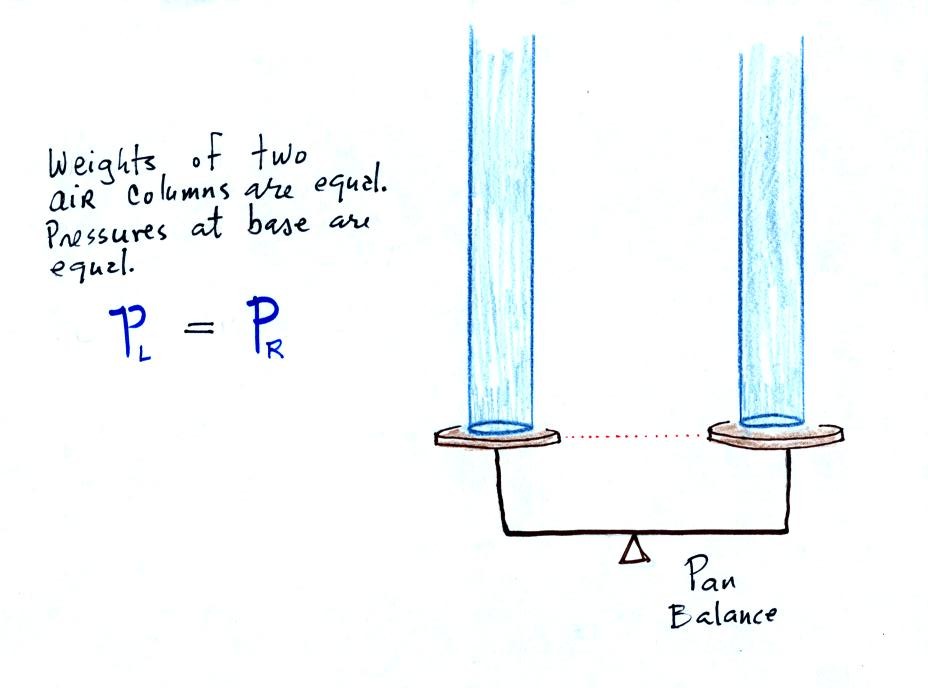
PL and PR are equal (note
you don't really know what either pressure is just that they are equal).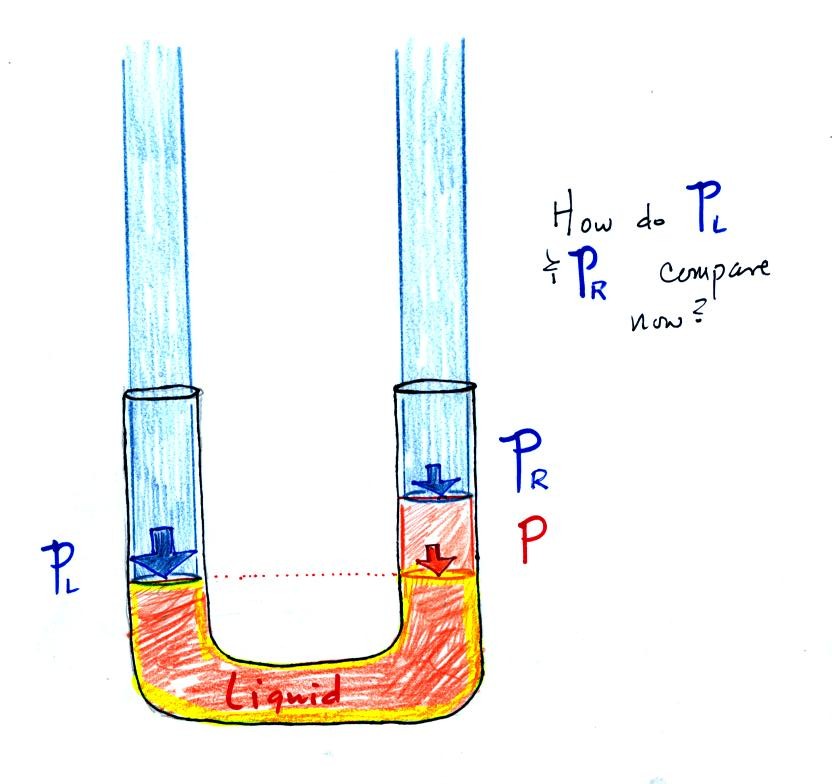
Now the situation is a little different, the
liquid levels
are no
longer equal. You probably realize that the air pressure on the
left, PL, is a little higher than the air pressure on the
right,
PR. PL is now being balanced by PR
+ P acting together. P
is the pressure produced by the extra fluid on the right hand side of
the manometer (the fluid that lies above the dotted line). The
height of the column of extra
liquid provides a measure of the difference between PL and PR.
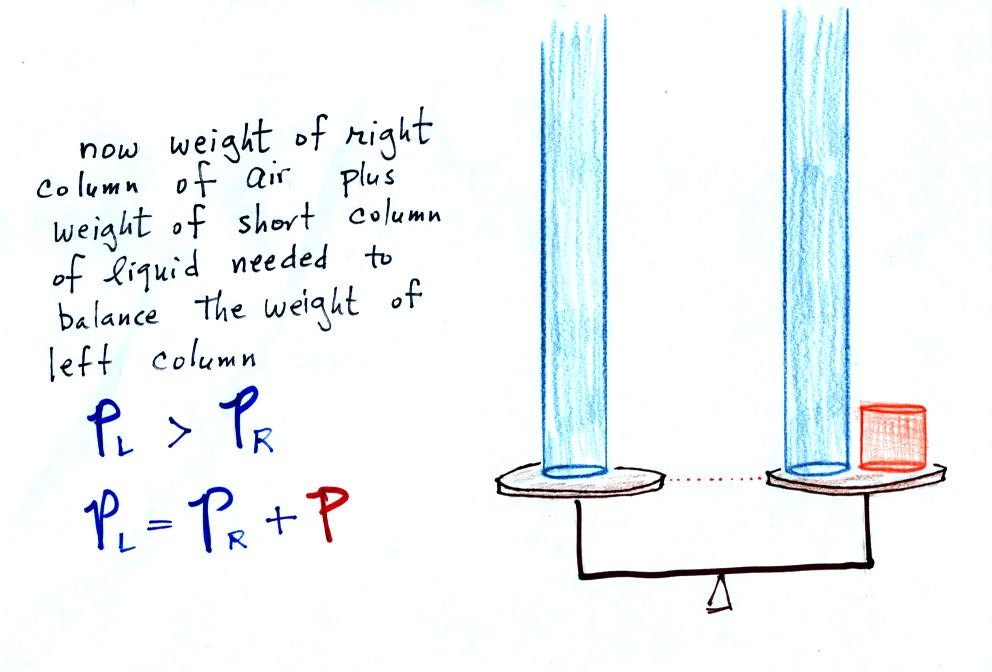
Next we will go an extreme and close off the right hand side of the
manometer.
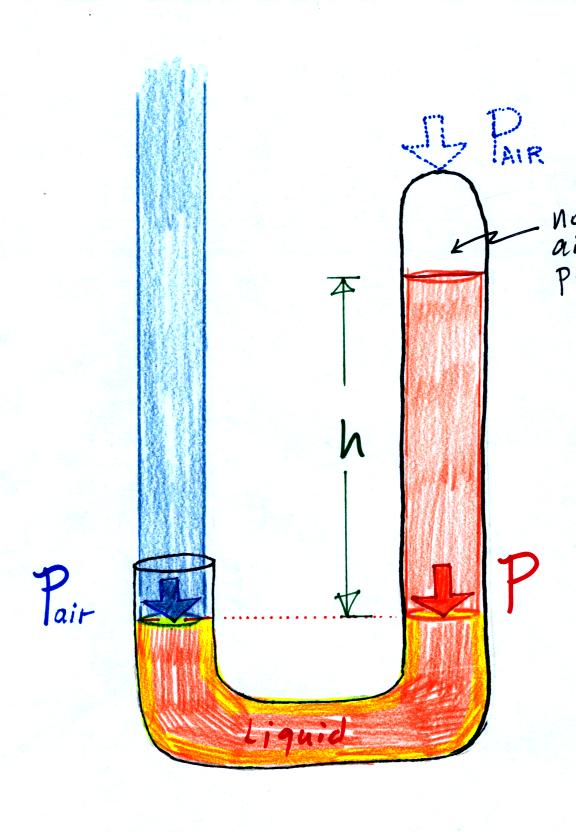
Air pressure can't get into the right tube any
more. Now at the level of the dotted line the balance is between
Pair and P (pressure by the extra liquid on the
right). If
Pair changes, the height of the right column, h, will
change. You now have a barometer, an instrument that can measure
and monitor the atmospheric pressure.
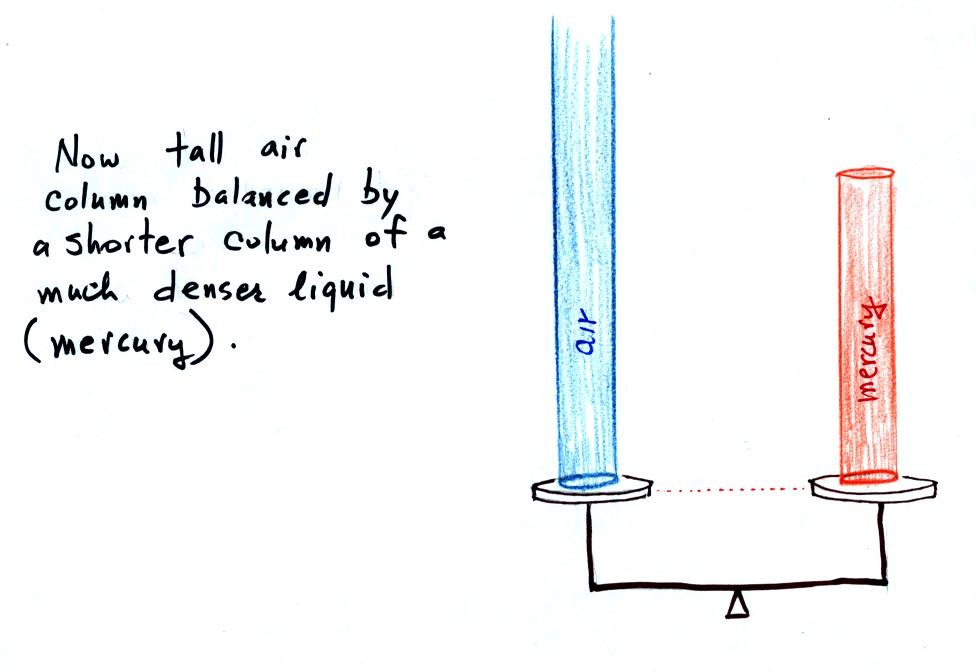
Barometers like this are usually filled with mercury. Mercury is
a liquid. You need a liquid that can slosh back and forth in
response to changes in air pressure. Mercury is also dense which
means the barometer won't need to be as tall as if you used something
like water. A water barometer would need to be over 30 feet
tall. With mercury you will need only a 30 inch tall column to
balance the weight of the atmosphere at sea level under normal
conditions (remember the 30 inches of mercury pressure units mentioned
earlier). Mercury also has a low rate of
evaporation so you don't have much mercury gas at the top of the right
tube.

Finally here is a more conventional barometer design.
The bowl of
mercury is usually covered in such a way that it can sense changes in
pressure but not evaporate and fill the room with poisonous mercury
vapor.
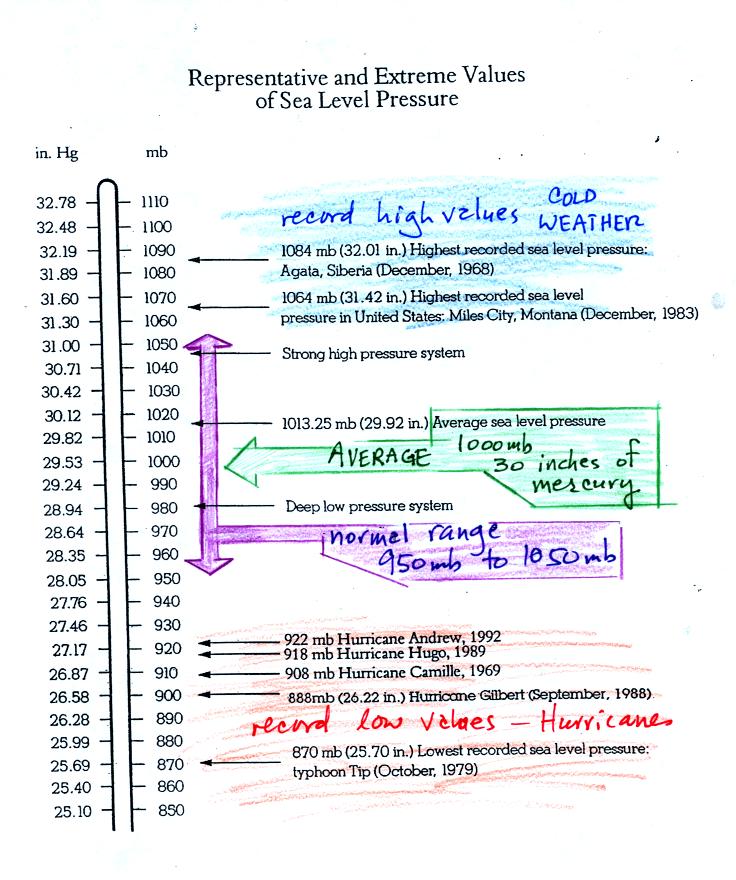
The figure above (p. 30 in the photocopied Class Notes)
first
shows average sea level pressure values (1000 mb or 30 inches of
mercury are close enough in this class). Sea level pressures
usually fall between 950 mb and 1050 mb. Record high sea level
pressure values occur during cold weather. Record low pressure
values have all been set by intense hurricanes (the record setting low
pressure is the reason these storms were so intense)
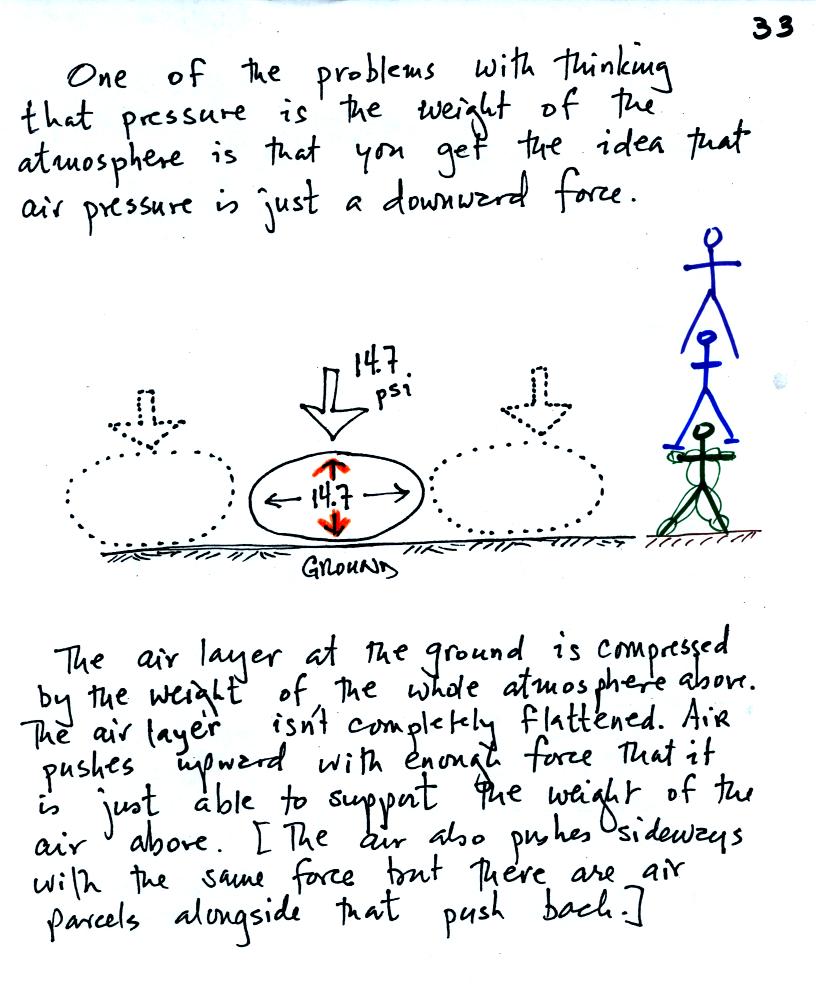
Air pressure is a force that pushes downward, upward, and
sideways.
If you fill a balloon with air and then push downward on it, you can
feel the air in the balloon pushing back (pushing upward). You'd
see the air in the balloon pushing sideways as well.
The bottom person in the people pyramid above must push upward with
enough
force to support the other people. The air in a layer at the
bottom of the atmosphere must do the same thing. It pushes upward
with enough force to support the weight of all the air overhead.
The air
pressure in the four tires on your automobile push down on the road
(that's something you would feel if the car ran over your foot) and
push upward
with enough force to keep the 1000 or 2000 pound vehicle off the
road.
We
finished class with a demonstration of the upward force caused by air
pressure.
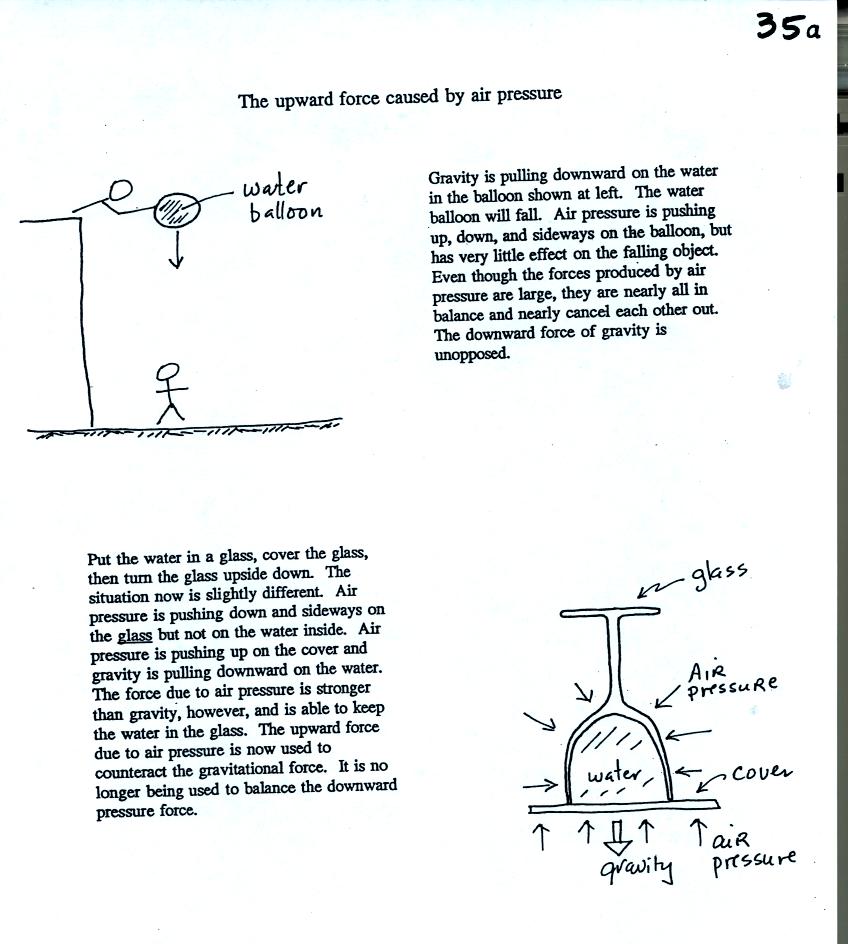
The demonstration is described on p. 35a in
the photocopied Class Notes.
The following more detailed look at
what is going on in the demonstration wasn't covered in class
. First
the case of a water balloon:
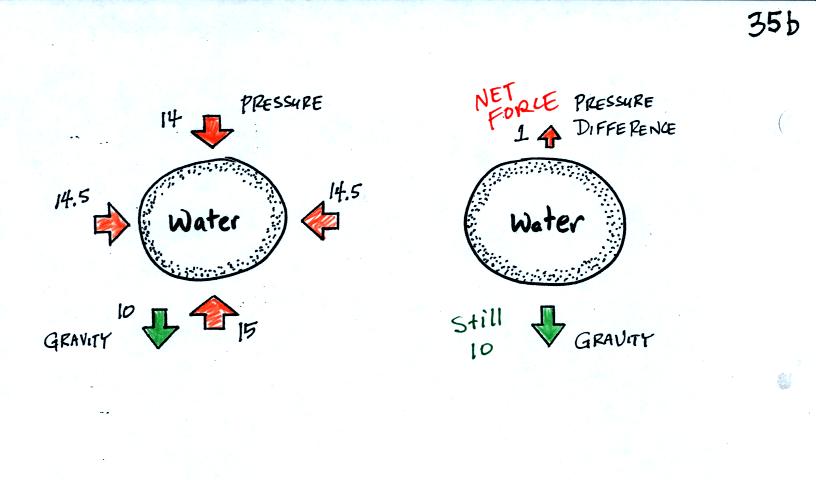
The figure at left shows air pressure (red
arrows)
pushing on all
the
sides of the balloon. Because pressure decreases with increasing
altitude, the pressure pushing downward on the top of the balloon is a
little weaker (strength=14) than the pressure pushing upward at the
bottom of the
balloon (strength=15). The two sideways forces cancel each other
out. The
total effect of the pressure is a weak upward force (shown on the right
figure, you might have heard this called a bouyant force).
Gravity exerts a downward force on the water
balloon. In the figure at right you can see that the gravity
force (strength=10) is stronger than the upward pressure difference
force (strength=1). The
balloon falls as a result.
In the demonstration a wine glass is filled with water. A small
plastic lid is used to cover the wine glass. You can then turn
the glass upside down without the water falling out.
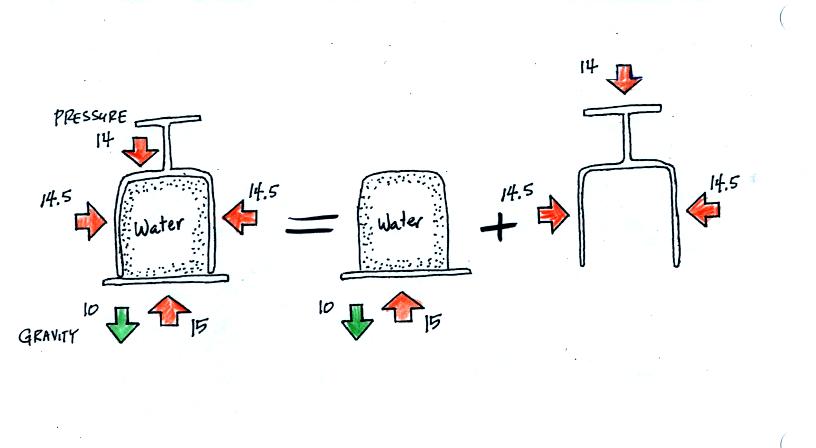
All the same forces are shown again in the left most
figure.
In
the right two figures we separate this into two parts. First
the water inside the glass isn't feeling the downward and sideways
pressure forces (because they're pushing on the glass). Gravity
still pulls downward on the water but the upward pressure force is able
to overcome the downward pull of gravity. The upward pointing
pressure force is used to overcome gravity not to cancel out the
downward pointing pressure force.
The demonstration was repeated using a 4 Liter flash (more than a
gallon of water, more than 8 pounds of water). The upward
pressure force was still able to keep the water in the flask (much of
the weight of the water is pushing against the sides of the flask which
the instructor was supporting with his arms).















