
We'll finish up the section on
composition of air and air
pollutants today by learning about tropospheric ozone. Then we'll
start a new block of material: mass, weight, density and
pressure. Rather
than covering stratopheric ozone in class, I think I will make that one
of the One-Side-of-One-Page report topic choices (the assignment will
be announced in class before the Practice
Quiz).
An iron bar was passed around at the beginning of the period so that everyone would have a chance to handle it and try to guess how much it weighed.
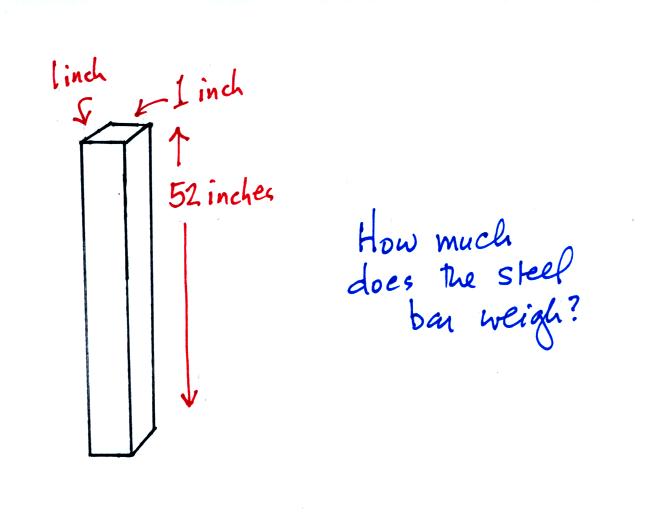
This will relate to something we'll be covering near the end of class today.
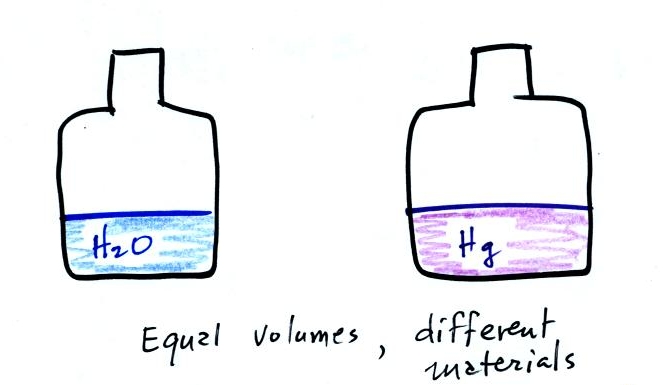
Bottles containing approximately equal volumes of water and mercury were also handed out (thanks for being careful with the mercury). There is a lot more mass in the bottle of mercury than in the bottle of water. Because it has more mass the bottle of mercury also weighs more than the bottle of water (that's something you can feel). Mercury is much denser than water. Again this is material we will be covering this morning.
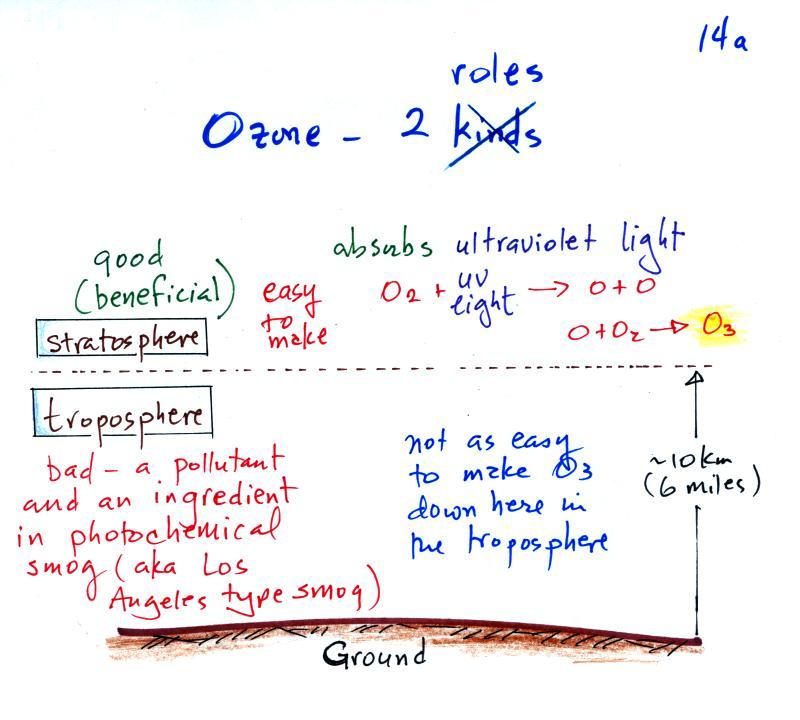
The figure above can be found on p. 14a in the photocopied ClassNotes. Ozone has a Dr. Jekyll (good) and Mr. Hyde (bad) personality. The ozone layer (ozone in the stratosphere) is beneficial, it absorbs dangerous high energy ultraviolet light (which would otherwise reach the ground and cause skin cancer, cataracts, etc. There are some types of UV light that would kill us).
Ozone in the troposphere is bad, it is a pollutant. Tropospheric ozone is a key component of photochemical smog (also known as Los Angeles-type smog)
We'll be making some photochemical smog as a class demonstration. To do this we'll first need some ozone; we'll make use of the simple stratospheric recipe (shown above) for making ozone in the demonstration rather than the more complex tropospheric process (the 4-step process in the figure below).
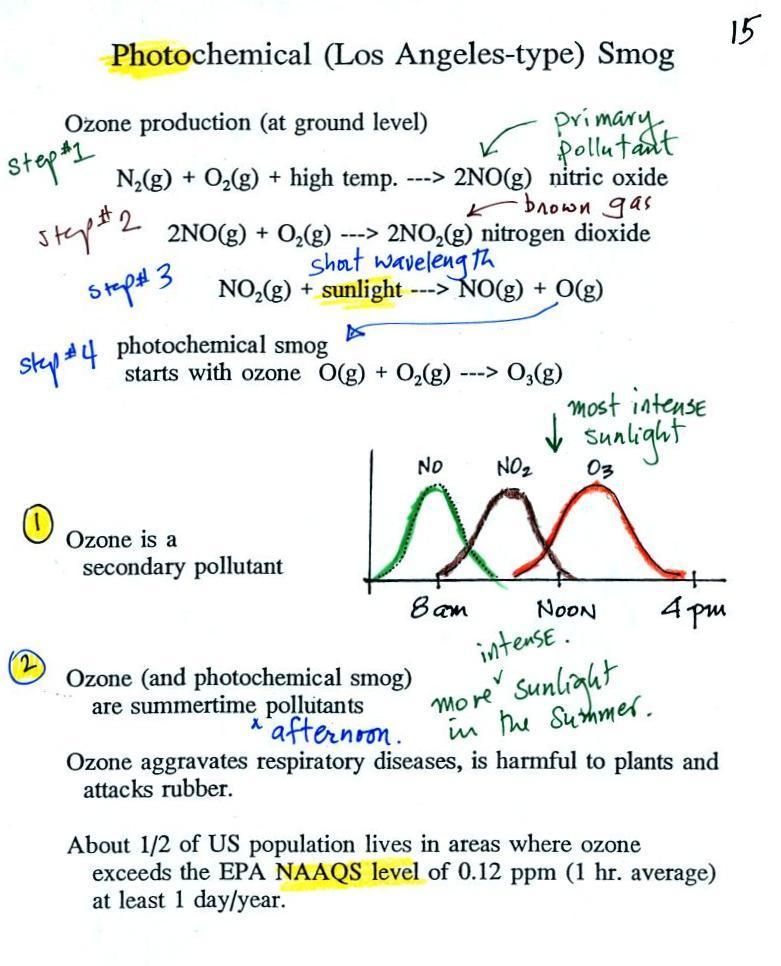
At the top of this figure (p. 15 in the packet of ClassNotes) you see that a more complex series of reactions is responsible for the production of tropospheric ozone. The production of tropospheric ozone begins with nitric oxide (NO). NO is produced when nitrogen and oxygen in air are heated (in an automobile engine for example) and react. The NO can then react with oxygen in the air to make nitrogen dioxide, the poisonous brown-colored gas that I've been thinking about making in class. Sunlight can dissociate (split) the nitrogen dioxide molecule producing atomic oxygen (O) and NO. O and O2 react in a 4th step to make ozone (O3). Because ozone does not come directly from an automobile tailpipe or factory chimney, but only shows up after a series of reactions in the air, it is a secondary pollutant. Nitric oxide would be the primary pollutant in this example.
NO is produced early in the day (during the morning rush hour). The concentration of NO2 peaks somewhat later. Because sunlight is needed in step #3 and because peak sunlight normally occurs at noon, the highest ozone concentrations are usually found in the afternoon. Ozone concentrations are also usually higher in the summer when the sunlight is most intense.
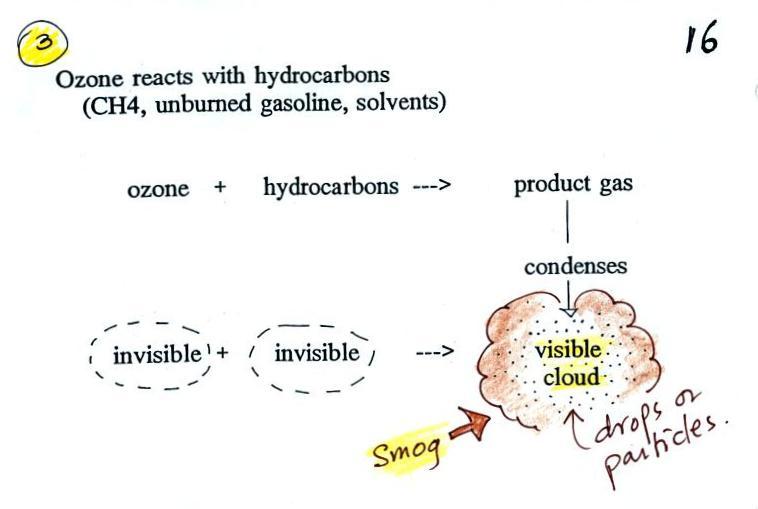
Once ozone is formed, the ozone can react with a hydrocarbon of some kind to make a product gas. The ozone, hydrocarbon, and product gas are all invisible, but the product gas sometimes condenses to make a visible smog cloud or haze. The cloud is composed of very small droplets or solid particles. They're too small to be seen but they are able to scatter light - that's why you can see the cloud.
Here's a pictorial summary of the photochemical smog demonstration.

We started by putting a small "mercury vapor" lamp inside a flash. The bulb produces a lot of ultraviolet light (the bulb produced a dim bluish light that we could see but UV light is invisible so we had no way of really telling how bright it was). The UV light and oxygen in the air produced a lot of ozone (you could easily have smelled it if you took off the cover of the flask).

After a few minutes we turned off
the lamp and put
a few pieces of lemon peel into the flash. Part of the smell that
comes from lemon peel is limonene, a hydrocarbon. The limonene
gas reacted with the ozone to produce a product gas. The product
gas
condensed, producing a visible smog cloud (the cloud was white, not
brown as shown above). I also shined the laser beam through the
smog cloud to reinforce the idea that we are seeing the cloud because
the drops or particles scatter light.
Next one of the brutal breaks and changes of topic that will periodically occur in this class. Now that we've learned about the composition of the atmosphere we will be learning about some of its other physical properties such as temperature, air density, and air pressure. We'll also be interested in how they change with altitude.

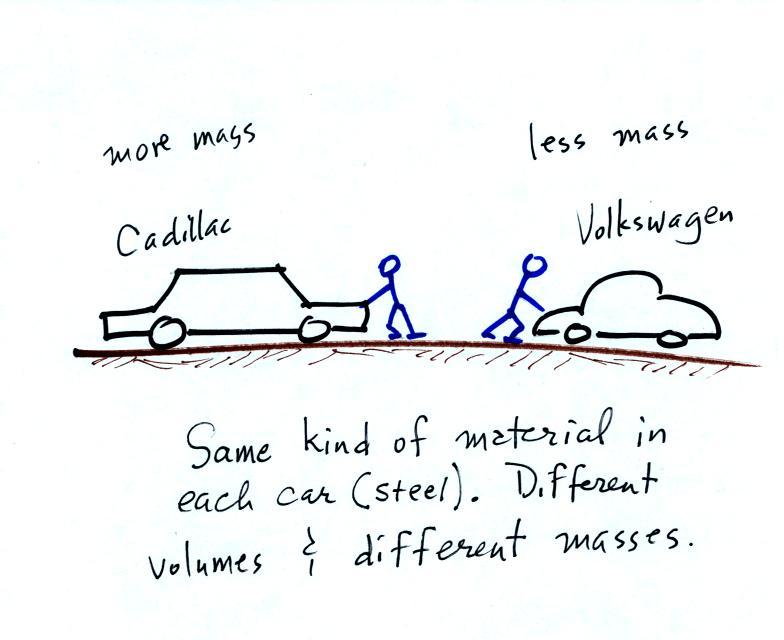
Before we can learn about atmospheric pressure in particular, we need to review the terms mass and weight. In some textbooks you'll find mass defined as "amount of stuff" or "amount of a particular material." Other books will define mass as inertia or as resistance to change in motion (this comes from Newton's 2nd law of motion, we'll cover that later in the semester). The next picture illustrates both definitions.

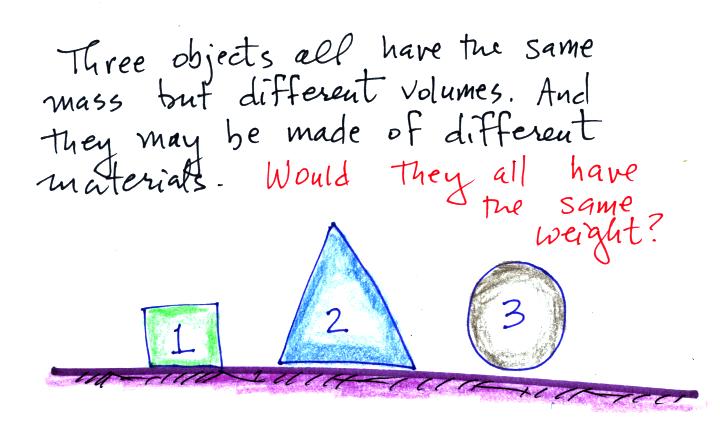


Density is the next term we need to look at.


An iron bar was passed around at the beginning of the period so that everyone would have a chance to handle it and try to guess how much it weighed.

This will relate to something we'll be covering near the end of class today.
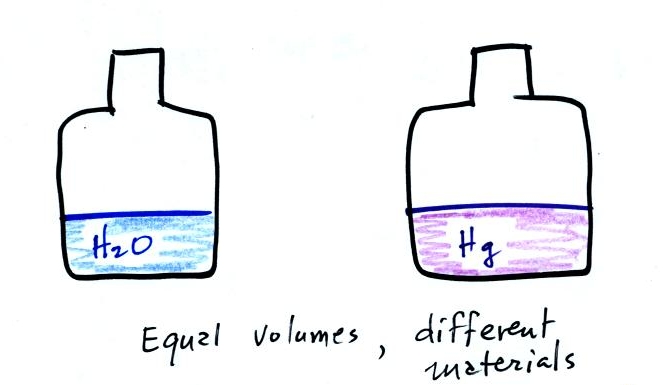
Bottles containing approximately equal volumes of water and mercury were also handed out (thanks for being careful with the mercury). There is a lot more mass in the bottle of mercury than in the bottle of water. Because it has more mass the bottle of mercury also weighs more than the bottle of water (that's something you can feel). Mercury is much denser than water. Again this is material we will be covering this morning.

The figure above can be found on p. 14a in the photocopied ClassNotes. Ozone has a Dr. Jekyll (good) and Mr. Hyde (bad) personality. The ozone layer (ozone in the stratosphere) is beneficial, it absorbs dangerous high energy ultraviolet light (which would otherwise reach the ground and cause skin cancer, cataracts, etc. There are some types of UV light that would kill us).
Ozone in the troposphere is bad, it is a pollutant. Tropospheric ozone is a key component of photochemical smog (also known as Los Angeles-type smog)
We'll be making some photochemical smog as a class demonstration. To do this we'll first need some ozone; we'll make use of the simple stratospheric recipe (shown above) for making ozone in the demonstration rather than the more complex tropospheric process (the 4-step process in the figure below).

At the top of this figure (p. 15 in the packet of ClassNotes) you see that a more complex series of reactions is responsible for the production of tropospheric ozone. The production of tropospheric ozone begins with nitric oxide (NO). NO is produced when nitrogen and oxygen in air are heated (in an automobile engine for example) and react. The NO can then react with oxygen in the air to make nitrogen dioxide, the poisonous brown-colored gas that I've been thinking about making in class. Sunlight can dissociate (split) the nitrogen dioxide molecule producing atomic oxygen (O) and NO. O and O2 react in a 4th step to make ozone (O3). Because ozone does not come directly from an automobile tailpipe or factory chimney, but only shows up after a series of reactions in the air, it is a secondary pollutant. Nitric oxide would be the primary pollutant in this example.
NO is produced early in the day (during the morning rush hour). The concentration of NO2 peaks somewhat later. Because sunlight is needed in step #3 and because peak sunlight normally occurs at noon, the highest ozone concentrations are usually found in the afternoon. Ozone concentrations are also usually higher in the summer when the sunlight is most intense.

Once ozone is formed, the ozone can react with a hydrocarbon of some kind to make a product gas. The ozone, hydrocarbon, and product gas are all invisible, but the product gas sometimes condenses to make a visible smog cloud or haze. The cloud is composed of very small droplets or solid particles. They're too small to be seen but they are able to scatter light - that's why you can see the cloud.
Here's a pictorial summary of the photochemical smog demonstration.

We started by putting a small "mercury vapor" lamp inside a flash. The bulb produces a lot of ultraviolet light (the bulb produced a dim bluish light that we could see but UV light is invisible so we had no way of really telling how bright it was). The UV light and oxygen in the air produced a lot of ozone (you could easily have smelled it if you took off the cover of the flask).

Next one of the brutal breaks and changes of topic that will periodically occur in this class. Now that we've learned about the composition of the atmosphere we will be learning about some of its other physical properties such as temperature, air density, and air pressure. We'll also be interested in how they change with altitude.

Before we can learn about atmospheric pressure in particular, we need to review the terms mass and weight. In some textbooks you'll find mass defined as "amount of stuff" or "amount of a particular material." Other books will define mass as inertia or as resistance to change in motion (this comes from Newton's 2nd law of motion, we'll cover that later in the semester). The next picture illustrates both definitions.

A Cadillac and a volkswagen
have both stalled in an intersection. Both cars are made of
steel. The Cadillac is larger and has more steel, more stuff,
more mass. The Cadillac would be much harder to get moving than
the VW, it has
a larger inertia (it would also be harder to slow down than the
Volkswagen once it is
moving).
As long as we're talking about cars, here's a picture of my vehicle. It's a 1980 Toyota Celica.
As long as we're talking about cars, here's a picture of my vehicle. It's a 1980 Toyota Celica.

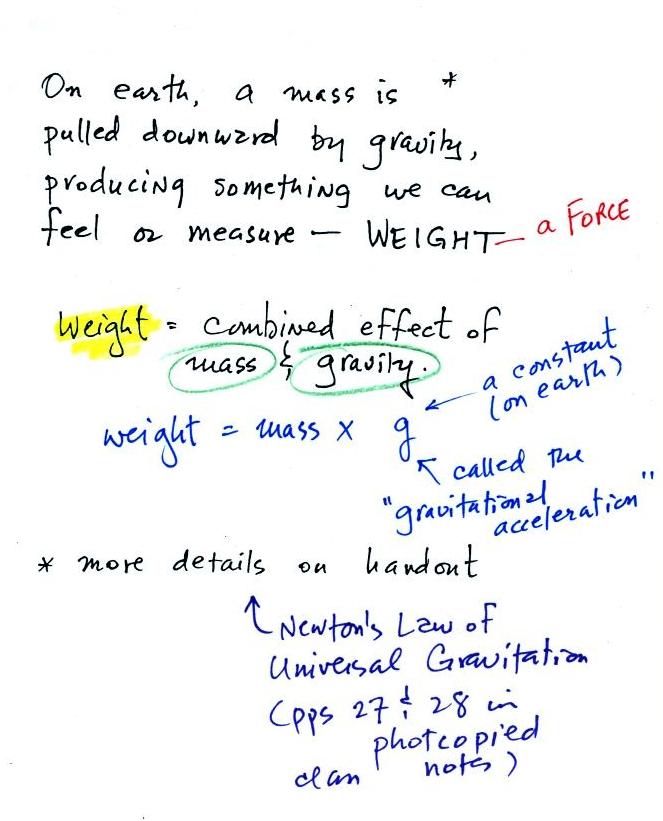
Weight
is a force and depends on
both the mass of an object and the
strength of gravity. We tend to use
weight and mass
interchangeably
because we spend all our
lives on earth where gravity never changes. On the earth's
surface you determine the weight of an object by multiplying the
object's mass by g. As long as you're on the surface of the earth
g has a constant value; it's called the gravitational
acceleration.
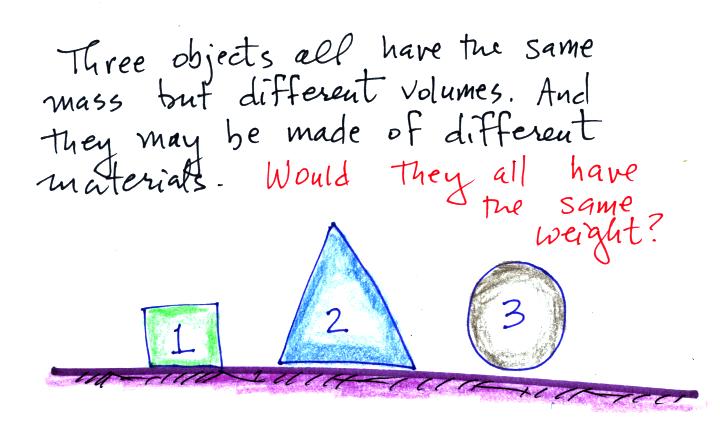
I asked a question at
this point. I would say
the people that responded to the question were about evenly
divided. Equal numbers answered yes (the objects would have the
same weight) and no (the weights would be different).

To determine the weight
you multiply the mass by the gravitational
acceleration. Since all three objects have the same mass and g is
a constant you get the same weight for each object. Here was a
follow up question:

A student responded
that the two objects would have
different weights if they were on different planets.
That's
correct.

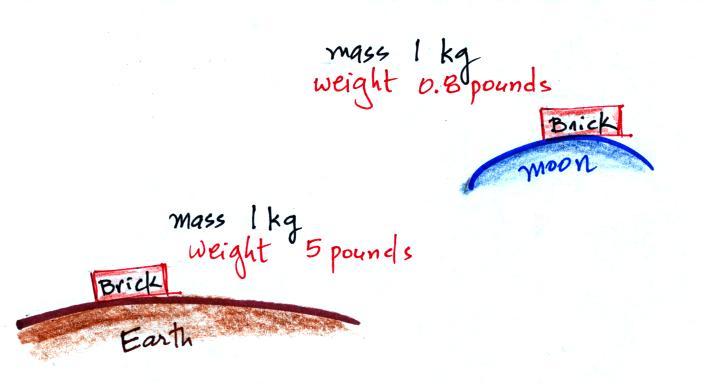
The example above looks
at bricks on the earth and the
moon. The value of the gravitational acceleration on the moon is
about 1/6th the value on the earth. The brick would weigh a lot
more than 5 pounds if you were to take it to Jupiter.
Here's a little more information (not covered in class) about what determines the value of the gravitational acceleration (Newton's Law of Universal Gravitation).
Here's a little more information (not covered in class) about what determines the value of the gravitational acceleration (Newton's Law of Universal Gravitation).
Density is the next term we need to look at.

In
the first example there is more mass (more dots, which symbolize air
molecules) in the right box than
in the left box. Since the two volumes are equal the box at right
has higher density. Equal masses are squeezed into different
volumes in the bottom example. The box with smaller volume has
higher density.
Now we're ready to
define (and hopefully understand)
pressure.
It's a pretty important concept. A lot of what happens in the
atmosphere is caused by pressure differences. Pressure
differences cause wind. Large pressure difference (such as you
might find in a tornado or a hurricane) create powerful and destructive
storms.

The air
that
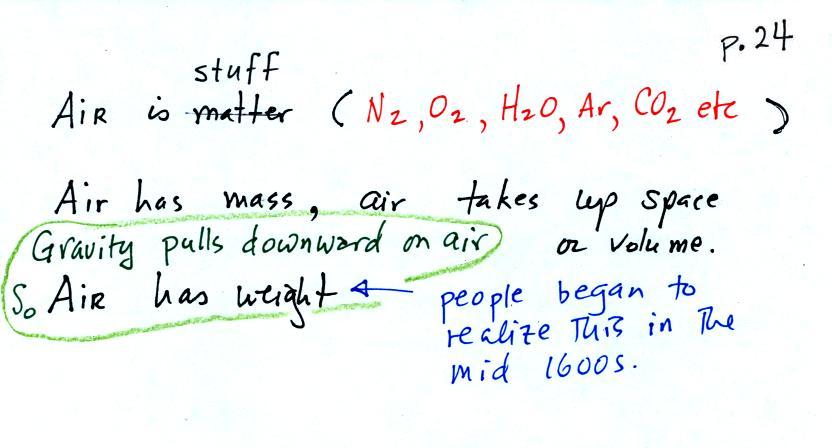
surrounds the earth has mass. Gravity pulls downward on the
atmosphere giving it weight. Galileo conducted (in the 1600s) a
simple
experiment
to
prove
that
air
has
weight. The experiment
wasn't mentioned
in class.
Atmospheric pressure is determined by the weight of the air overhead. This is 1 of 2 ways, a sort of large scale representation, of understanding air pressure.
Atmospheric pressure is determined by the weight of the air overhead. This is 1 of 2 ways, a sort of large scale representation, of understanding air pressure.

Pressure is defined as force
divided by area. In the case of
atmospheric pressure the weight of a column of air divided by the area
at the bottom of the column (as illustrated above).
Under normal conditions a 1 inch by 1 inch column of air stretching from sea level to the top of the atmosphere will weigh 14.7 pounds. Normal atmospheric pressure at sea level is 14.7 pounds per square inch (psi). These are the units you use when you fill up your car or bike tires with air. A car usually takes around 30 psi, I put about 90 psi in the thin tires on my bicycle.
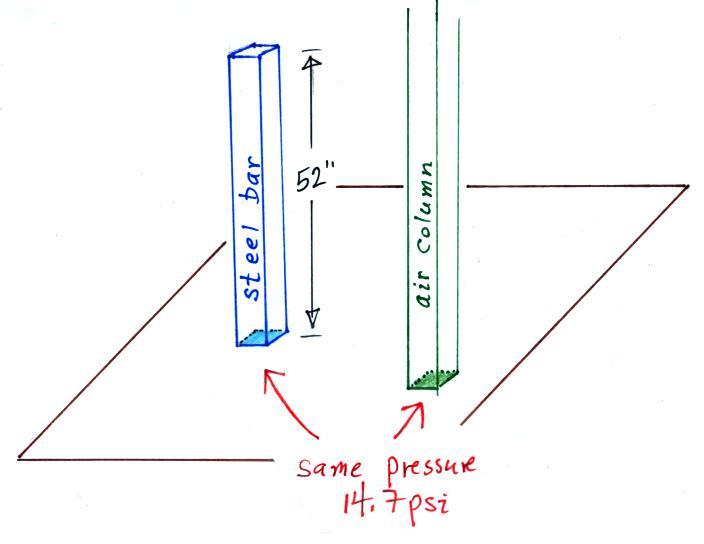
This is where the steel bar
comes in. The steel bar also weighs exactly 14.7 pounds (many
people think it is heavier than that). Steel is a lot denser
than air, so a steel bar only needs to be
52 inches tall to have the same weight as an air column that is 100 or
200 miles tall.
14.7 psi is one weigh of expressing average sea level pressure. Here are average sea level pressure values in different units.
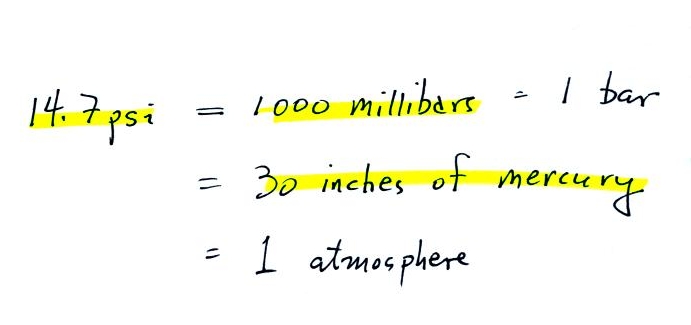
Under normal conditions a 1 inch by 1 inch column of air stretching from sea level to the top of the atmosphere will weigh 14.7 pounds. Normal atmospheric pressure at sea level is 14.7 pounds per square inch (psi). These are the units you use when you fill up your car or bike tires with air. A car usually takes around 30 psi, I put about 90 psi in the thin tires on my bicycle.

14.7 psi is one weigh of expressing average sea level pressure. Here are average sea level pressure values in different units.
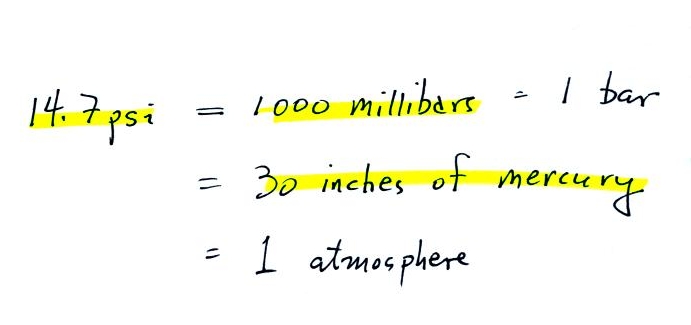
Typical sea level
pressure is 14.7 psi or about 1000 millibars
(the
units used by meterologists and the units that we will use in this
class most of the time) or about 30 inches of mercury (refers to
the reading on a mercury barometer).
We'll talk briefly about mercury barometers next week. They're used to measure atmospheric pressure.
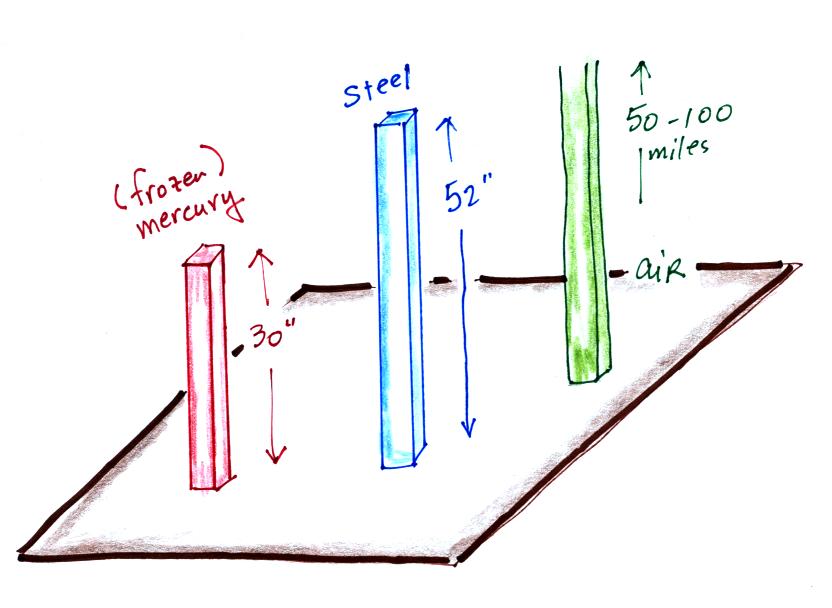
Mercury is more dense than steel and you only need a 30 inch column of mercury to balance the weight of a column of air. And that's basically what a mercury barometer does. It basically balances the weight of a tall column of air with a shorter column of mercury.

Here's a picture of a car I used to own. It's A French Peugeot 404 (mine was in much worse condition than this one). One of the first things I did after buying it was to fill the tank with gasoline and to check the tire pressure. The scale on the air compressor ran from 0 to 3 and it took me 15 or 20 minutes to realize that it was calibrated in bars instead of psi. So I put about 2 bars into each tire and proudly drove off.
One last figure, not shown in class.

Bar means pressure; you'll find it in millibars, barometer, and
isobars. Isobars are contours of pressure
drawn on weather maps.
We'll talk briefly about mercury barometers next week. They're used to measure atmospheric pressure.

Mercury is more dense than steel and you only need a 30 inch column of mercury to balance the weight of a column of air. And that's basically what a mercury barometer does. It basically balances the weight of a tall column of air with a shorter column of mercury.

Here's a picture of a car I used to own. It's A French Peugeot 404 (mine was in much worse condition than this one). One of the first things I did after buying it was to fill the tank with gasoline and to check the tire pressure. The scale on the air compressor ran from 0 to 3 and it took me 15 or 20 minutes to realize that it was calibrated in bars instead of psi. So I put about 2 bars into each tire and proudly drove off.
One last figure, not shown in class.
