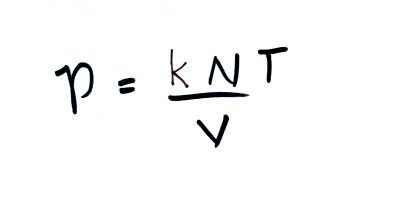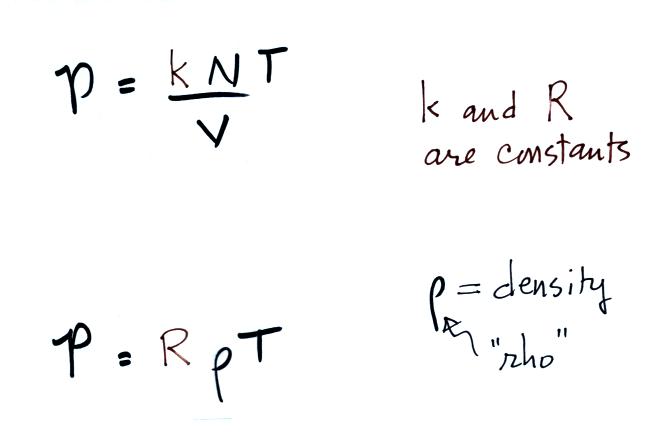Thursday Sept. 15, 2016
La Santa Cecilia: "Losing Game"
(3:15), "La
Negra" (3:16), "I Won't Cry
for You (English)" (3:40), "No Te Lloro
Mas (Espanol)" (3:21), "Tainted Love"
(2:53), "Nunca
Mas" (3:45)
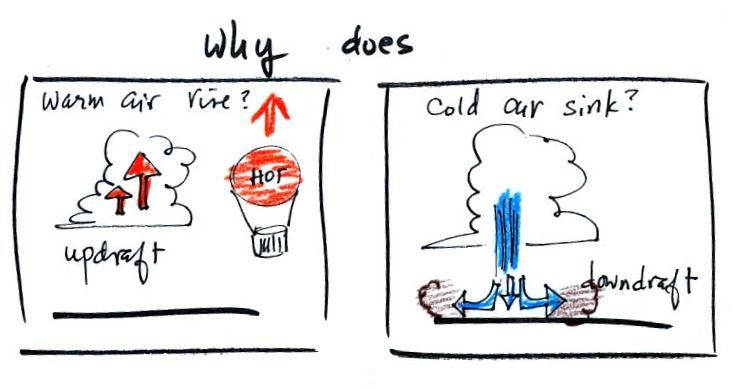
An ambitious goal today:
trying to understand why warm air rises & cold air sinks

|

|
Hot air balloons floating over the Rio
Grande river during the Albuquerque Balloon Fiesta (source
of the photo)
|
Photograph of a microburst, a localized
intense thunderstorm downdraft, that hit Wittmann Arizona
in July 2015. Surface winds of 55 MPH were measured.
(source
of the photo)
|
A full understanding of these rising and sinking motions is a
3-step process (the following is from the bottom part of p. 49 in
the photocopied ClassNotes).
We will first learn about the
ideal gas law. It's is an equation that tells you which
properties of the air inside a balloon work to determine the
air's pressure. Then we will look at Charles' Law, a
special situation involving the ideal gas law (air temperature
volume, and density change together in a way that keeps the
pressure inside a balloon constant). Then we'll learn
about the 2 vertical forces that act on air. I'm pretty
sure you know what the downward force is and am about equally
sure you don't remember what the upward force is (even though
it is something that has come up before in this class this
semester, on Tuesday as a matter of fact).
The ideal gas law - a microscopic scale explanation of
air pressure
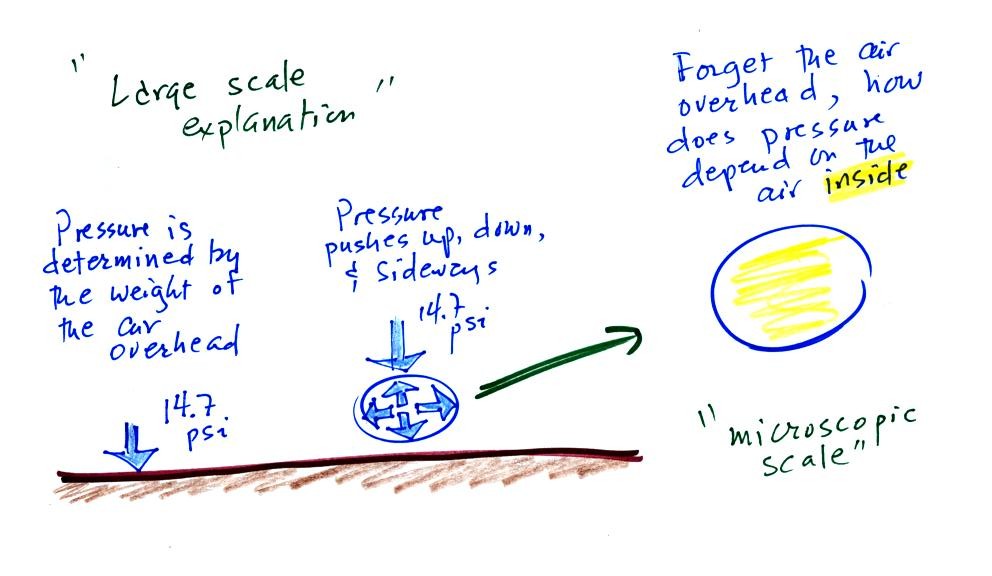
We've spent a fair amount of time
learning about pressure. We first began thinking of
pressure as being determined by the weight of the air
overhead. Air pressure pushes down against the ground at
sea level with 14.7 pounds of force per square inch.
That's a perfectly sound explanation.
We then went a bit further and tried to imagine the weight of
the atmosphere pushing down on a balloon sitting on the
ground. If you actually do push on a balloon you realize
that the air in the balloon pushes back with the same
force. Air pressure everywhere in the atmosphere pushes
upwards, downwards, and sideways.
These are large scale, atmosphere size, ways of thinking about
pressure. Next we are going to concentrate on just the
air in the balloon pictured above. This is more of a
microscopic view of pressure.
Imagine filling a balloon with air. If you could
look inside which picture below would be more realistic?
The view on the left is
incorrect.
The air molecules actually do not fill the balloon and
take up all the available space.
|
This is the correct
representation.
The air molecules are moving
around at 100s of MPH but actually take up little or no
space in the balloon.
|
The air molecules are continually colliding
with the walls of the balloon and pushing outward (this force
divided by area is the pressure). Wikipedia
has a
nice animation. An individual molecule doesn't
exert a very strong force, but there are so many molecules
that the combined effect is significant.
We want to identify the properties or characteristics of the
air inside the balloon that determine the pressure and then put
them together into an equation called the ideal gas law
(actually there'll be two equations).
The ideal gas law equations
You're not going to have to be able to figure out or remember
the ideal gas law equation. I'll give it to you. Here
is is:
You should know what the symbols in the equation
represent. Probably the most obvious variable is N the
number of air molecules. It's
the motions of the air molecules that produce pressure. No
air molecules (N = 0) means no pressure. The more air
molecules there are the higher the pressure.
Number of gas molecules or atoms
Pressure (P) is
directly proportional to Number of air molecules (N). If N
increases P increases and vice versa.
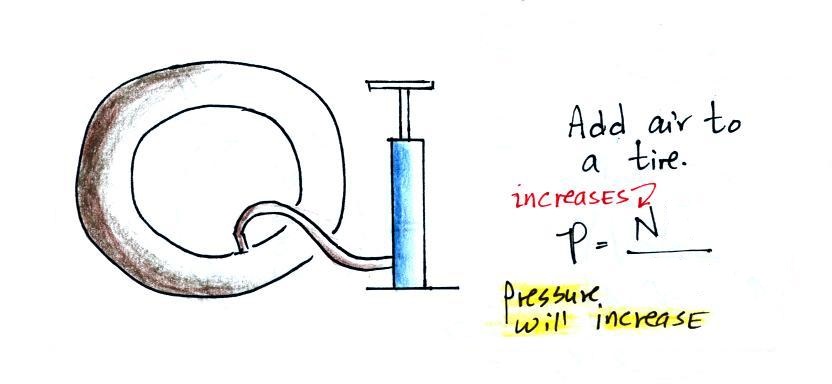
Here's an example. You're adding air
to a tire. As you add more and more air to something like a
bicycle tire, the pressure increases. Pressure is directly
proportional to N; an increase in N causes an increase in P.
If N doubles, P also doubles (as long as the other variables in
the equation don't change).
Temperature
Here's what I think is the next most obvious variable.
You shouldn't throw a
can of spray paint into a fire because the temperature
will cause the pressure of the gas (propellant) inside
the can to increase and the can could explode.
So T (temperature) belongs in the ideal gas law equation
Increasing the
temperature of the gas in a balloon will cause the gas
molecules to move more quickly (kind of like "Mexican
jumping beans"). They'll collide with the
walls of the balloon more frequently and rebound with
greater force - that will increase the pressure.
We've gotten a little bit ahead of the story. The
variable V (volume) has appeared in the equation and it's in the
denominator. A metal can is rigid. It's
volume can't change. When we start talking about volumes of
air in balloons or in the atmosphere volume can change. A
change in temperature or a change in number of air molecules might
be accompanied by a change in volume.
At this point we did a quick demonstration to show the effect
of temperature on the pressure of the gas in a rigid sealed
container (N and V in the ideal gas law equation stay constant,
just as in a can of spray paint).
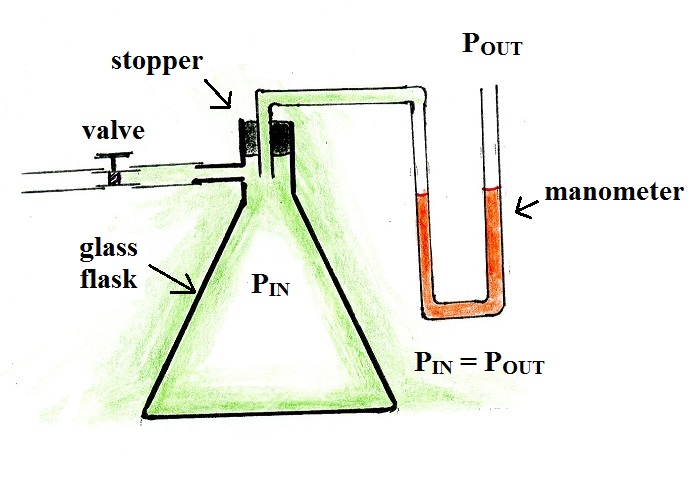
The container was a glass flask, sealed with a rubber
stopper. A piece of tubing with a valve was connected to the
flask. The valve was opened at the start of the
demonstration to be sure the pressures inside and outside the
flask were equal. The valve was then closed. The
manometer is a U-shaped tube filled with a liquid (transmission
oil) that can detect differences in pressure. Pressure from
the air inside the flask could enter one end of the manometer
tube. The other end was exposed to the pressure of the air
outside the flask.
Green in the figure indicates that the temperatures of the air
inside and outside the flask were equal. The manometer is
showing that the pressure of the air inside and outside the flask
were equal.

I wrapped my hands around the flask to warm the air inside very
slightly. The increase in air temperature caused a slight
increase in the pressure of the air inside the flask. The
air outside didn't change. Note the change in the levels of
the liquid in the manometer indicating the increase of the air
pressure inside the flask.
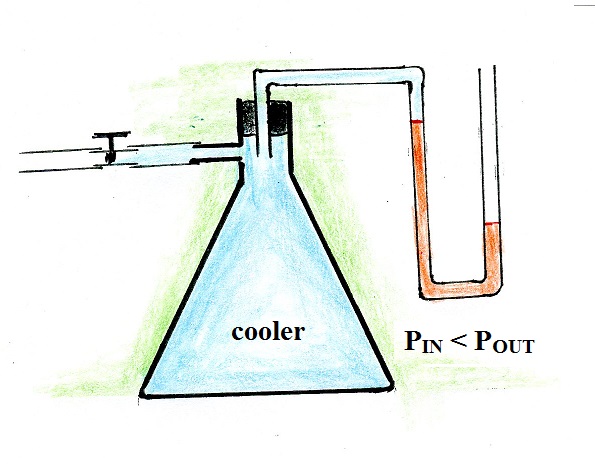
The valve was opened momentarily so that the pressures inside
and out would again be equal. The valve was then closed and
some isopropyl alcohol (rubbing alcohol) was dribbled on the
outside of the flask. As the alcohol evaporated it cooled
the flask and the air inside the flask. This caused the air
pressure inside the flask to drop. This change in air
pressure was again indicated by the liquid levels in the
manometer.
Volume
The effect of volume on pressure might be a little harder to
understand. Just barely fill a balloon with air, wrap your
hands around it, and squeeze it. It's hard and you don't
compress the balloon very much at all.
Or think of the bottom layer of the atmosphere
being squished by the weight of the air above. As the
bottom layer is compressed and its volume shrinks it pushes
back with enough force to eventually support the air above.
A decrease in volume
causes an increase in pressure, that's an inverse
proportionality.
It might take three or four
breaths of air to fill a balloon. Think about
that. You add some air (N increases) and the balloon
starts to inflate (V increases). Then you add another
breath of air. N increases some more and the balloon
gets a little bigger, V has increased again. As you
fill a balloon N and V are both increasing. What is
happening in this case is that the pressure of the air in
the balloon is staying constant. The
pressure inside the balloon pushing outward and trying to
expand the balloon is staying
equal to (in balance with) the pressure of the air outside
pushing inward and trying to compress the balloon.
Here's the same picture again except N and V
are decreasing together in a way that keeps pressure
constant. This is exactly what is happening in Experiment
#1.
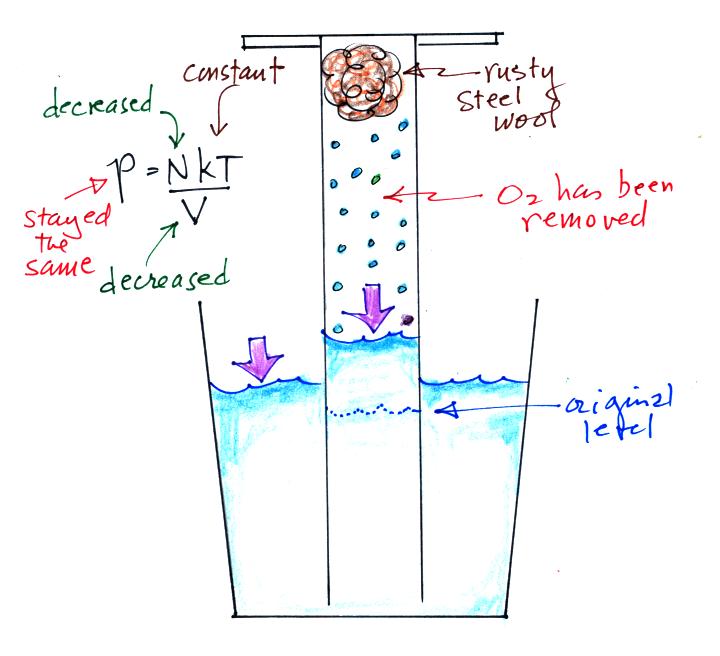
Experiment #1
- P stays constant, N & V both decrease
Here's a little more detailed explanation of Expt. #1
|
|
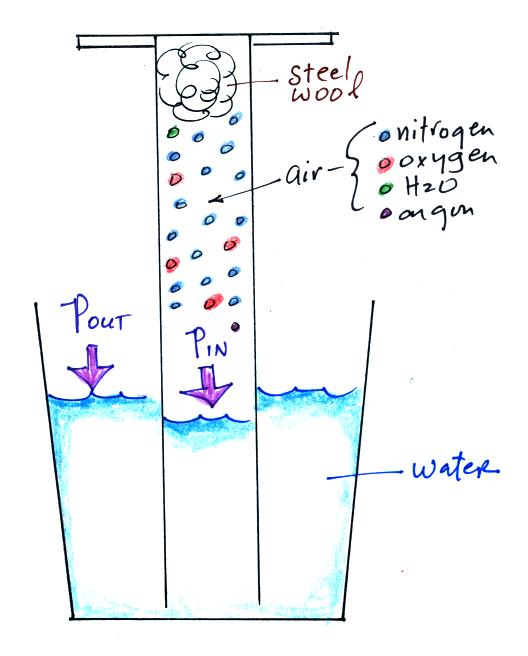
The object of Experiment
#1 is to measure the percentage
concentration of oxygen in the air.
An air sample is
trapped together with some steel wool inside a graduated
cylinder. The cylinder is turned upside down and
the open end is stuck into a glass of water sealing off
the air sample from the rest of the atmosphere.
This is shown at left above. The pressure of air
outside the cylinder tries to push water into the
cylinder, the pressure of the air inside keeps the water
out.
Oxygen in the cylinder reacts with
steel wool to form rust. Oxygen is removed from
the air sample which causes N (the total number of air
molecules) to decrease. Removal of oxygen would
ordinarily cause a drop in Pin
and
upset the balance between Pin
and Pout
. But, as oxygen
is removed, water rises up into the cylinder decreasing
the air sample volume. The decrease in V is what
keeps Pin
equal to Pout
.
N and V both decrease together in the same relative
amounts and the air sample pressure remains constant.
If you were to remove 20% of the air molecules, V would
decrease to 20% of its original value and pressure would stay
constant. It is the change in V that you can see, measure,
and use to determine the oxygen percentage concentration in
air. You should try to explain this in your experiment
report.
You might think that the mass of the gas molecules inside a
balloon might affect the pressure (big atoms or molecules might
hit the walls of the balloon harder and cause higher pressure
and vice versa).
The mass of the air molecules doesn't matter. The big ones
move relatively slowly, the smaller ones more quickly.
They both hit the walls of the balloon with the same
force. A variable for mass doesn't appear in
the ideal gas law equation.
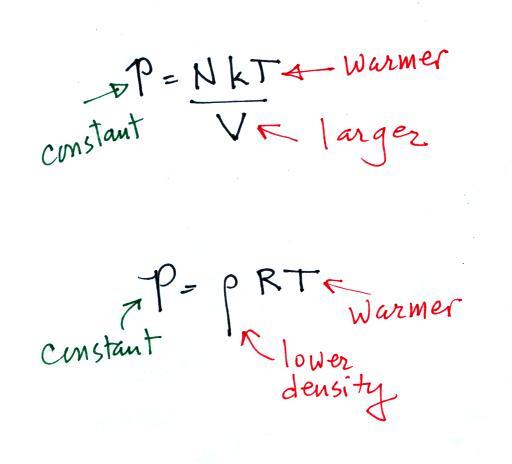
The figure below shows two forms of the ideal
gas law. The top equation is the one we've been looking at
and the bottom is a second slightly different version. You
can ignore the constants k and R if you are just trying to
understand how a change in one of the variables would affect the
pressure. You only need the constants when you are doing a
calculation involving numbers and units (which we won't be
doing).
The ratio N/V is similar to density
(mass/volume). That's where the ρ (density)
term in the second equation comes from.
Step #2 Charles Law
Charles Law means that P (pressure) in the ideal gas law
stays constant. Changing the temperature
of a volume of air will cause a change in density and volume;
pressure will stay constant. This is an important situation
because this is how volumes of air in the atmosphere behave.
This is probably the most difficult part of today's class and is
worked out in lots of detail.
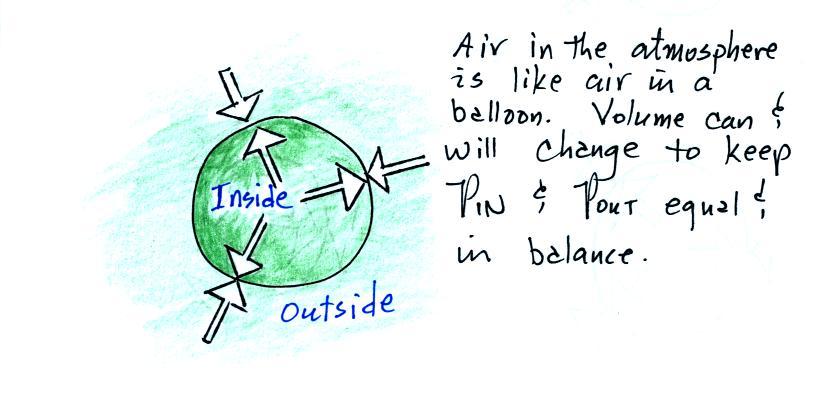
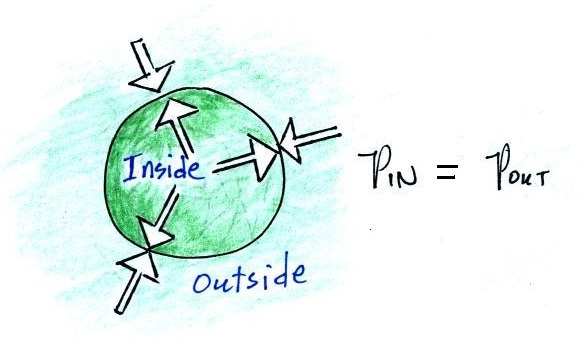
We will use a balloon of air. The air inside and
outside the balloon (or parcel) are exactly the same.
The pressure of the air surrounding the balloon pushing inward is
balanced by the pressure of the air inside the balloon that is
pushing outward. If we change something inside the balloon
that upsets this balance, the balloon would expand or shrink until
the pressures are again in balance.
Volumes of air in the atmosphere will behave the same
way.
First let's imagine warming the air inside a balloon. We'll
won't change the temperature of the air outside the balloon.
Increasing the temperature will momentarily increase the
pressure. This creates an imbalance. Now that P inside
is greater than P outside the balloon will expand.
Increasing the volume causes the pressure to start to
decrease. The balloon will keep expanding until P inside is
back in balance with P outside.
We're left with a balloon that is larger, warmer, and filled with
lower density air than it was originally.
The pressures inside and outside are again the same. The
pressure inside is back to what it was before we warmed the air in
the balloon. You can increase the temperature and volume of
a parcel together in a way that keeps pressure constant (which is
what Charles' law requires). This is equivalent to
increasing the temperature and decreasing the density together and
keeping the pressure constant.
In nature the change in temperature and volume occur
simultaneously. It's like jumping from the first to the last
step above.
Warming a parcel of
atmospheric air will cause the parcel volume to
increase, the density of the air in the parcel to
decrease, while pressure remains constant.
We can go through the same kind of reasoning and see what happens
if we cool the air in a parcel. Actually you should see if
you can figure it yourself. I've included all the steps
below. We'll just skip to the last step in class.
We'll start with a parcel of air that has the same temperature
and density as the air around it.
We'll cool the air inside the parcel. The air outside
stays the same.
Reducing the air temperature causes the pressure of the air
inside the balloon to momentarily decrease. Because the
outside air pressure is greater than the pressure inside the
balloon the parcel is compressed.
The balloon will get smaller and smaller (and the pressure
inside will get bigger and bigger) until the pressures inside and
outside the balloon are again equal. The pressure inside is
back to the value it had before you cooled the air in the parcel.
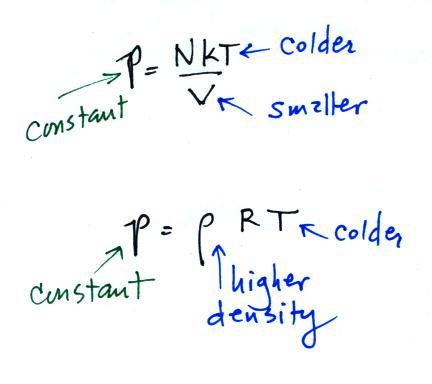
The first and last steps, without all the intermediate and
momentary details, are shown below.
Cooling some air will cause volume to decrease and density to
increase while pressure stays constant.
If you want to skip all the details and just remember one
thing, here's what I'd recommend
Demonstration of Charles Law in action
Parcels of atmospheric air and air in balloons behave the same
way, they both obey Charles' Law. Charles Law
can be demonstrated by dipping a balloon in liquid nitrogen.
You'll find an explanation on the top of p. 54 in the photocopied
ClassNotes.
A balloon shrinks down to practically zero volume when dunked
in the liquid nitrogen. When pulled from the liquid nitrogen
the balloon is filled with very cold, very high density air.
Then the balloon starts to warm up.
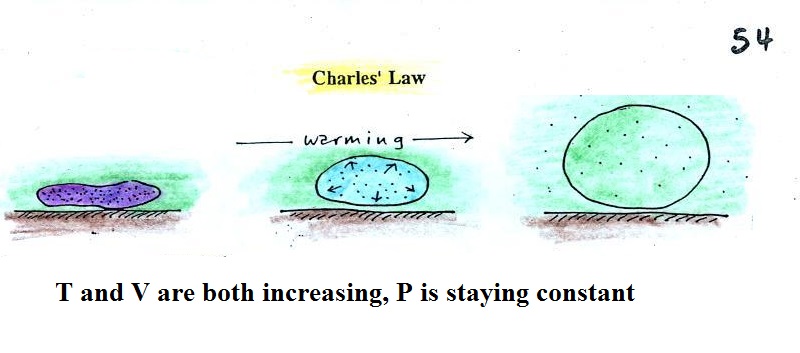
The volume and temperature both increasing
together in a way that kept pressure constant (pressure inside
the balloon is staying equal to the air pressure outside the
balloon). Eventually the balloon ends up back at room
temperature (unless it pops while warming up).
All of that was just Step #2,
we've still got Step #3
Step #3 Two vertical forces acting on a parcel of air
in the atmosphere
(see p. 53 in the ClassNotes)
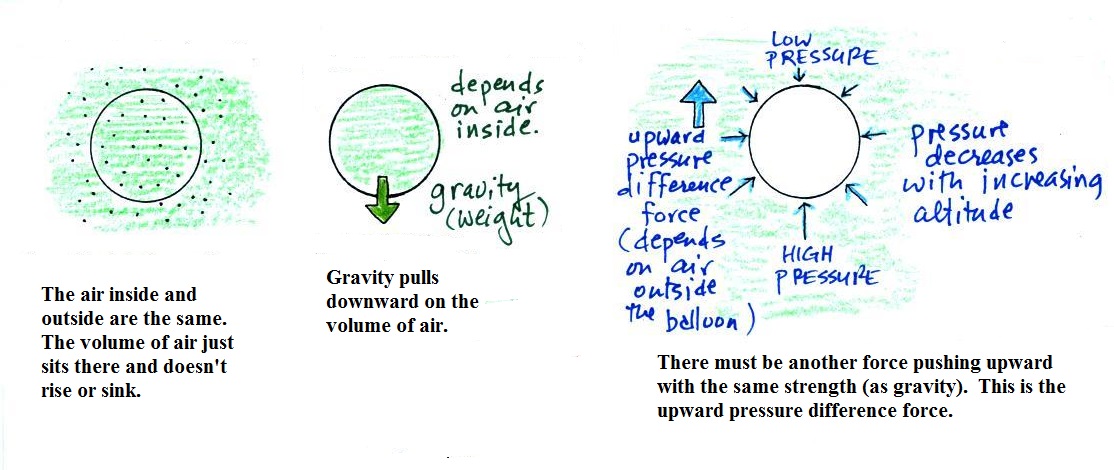
Basically it comes down to this - there
are two forces acting on a parcel of air in the atmosphere. They
are shown above.
The first force is gravity, it pulls downward. Most
everyone knows about this force. The strength of the
gravity force (the weight of the air in the parcel) depends on
the mass of the air inside
the parcel.
Second there is an upward pointing pressure difference
force. Not too many people know about this one. This
force is caused by the air outside (surrounding) the parcel.
Pressure decreases with increasing altitude. The pressure
of the air at the bottom of a parcel pushing upward is slightly
stronger than the pressure of the air at the top of the balloon
that is pushing downward. The overall effect is an upward
pointing force.
When the air inside a parcel is exactly the same as the air
outside (same densities), the two forces are equal in strength
and cancel out. The parcel is neutrally buoyant and it
wouldn't rise or sink, it would just hover.
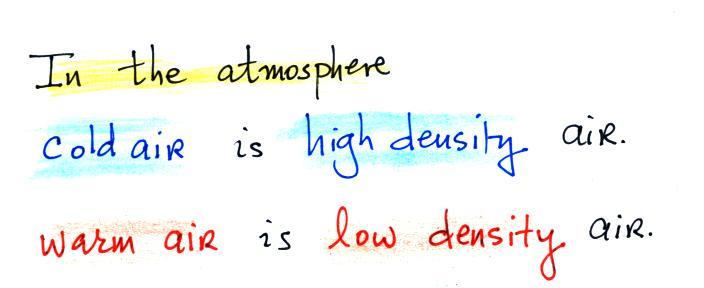
We'll replace the air inside the balloon
with either warm low density air or cold high density
air.
In the first case, a balloon with warm low density air won't
weigh as much. The gravity force is weaker. The
upward pressure difference force doesn't change (because it is
determined by the air outside the balloon which hasn't changed)
and ends up stronger than the gravity force. The balloon
will rise.
Conversely if the air inside is cold high density air, it
weighs more. Gravity is stronger than the upward pressure
difference force and the balloon sinks.
It all comes down to a question of how the density of
the air in a parcel compares to the density of the air
surrounding the parcel. If the parcel is filled
with low density air it will rise. A parcel full of high
density air will sink. That's true of things other than
air. Wood floats in water because it is less dense than
water.
Here's a short demonstration of the role
that density plays in determining whether a balloon will rise or
sink (or hover)
Convection demonstration
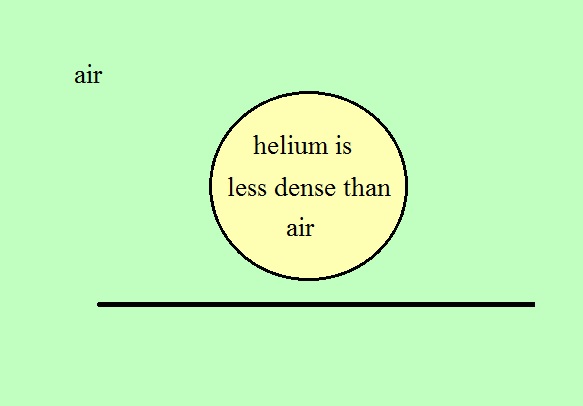
We used balloons filled with helium (see
bottom of p. 54 in the photocopied Class Notes).
Helium is less dense than air even when it has the same
temperature as the surrounding air. The downward
gravity force (weight of the helium filled balloon) is
weaker than the upward pressure difference force. You
don't need to warm a helium-filled balloon to make it rise.
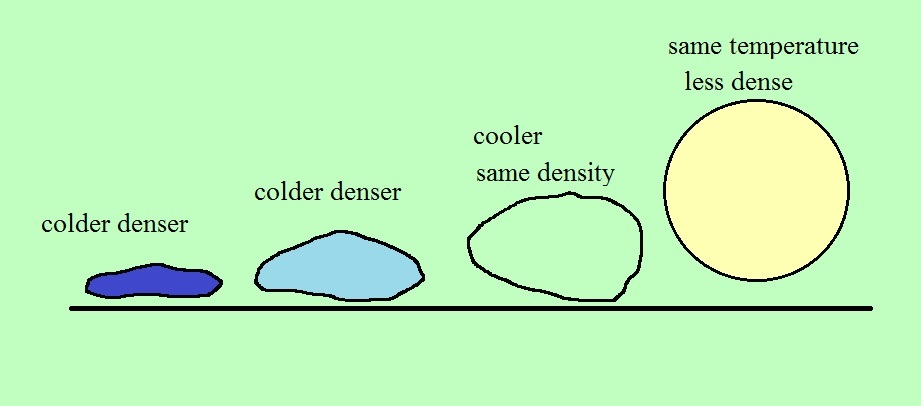
We dunk the helium filled balloon in
liquid nitrogen to cool it off. When you pull the balloon
out of the liquid nitrogen it has shrunk. The helium is
denser than the surrounding air. I set it on the table (dark
blue above) and it just sat there.
As the balloon of helium warms and expands its density decreases
(light blue). For a brief moment it has the same density as
the surrounding air (green). It's neutrally buoyant at this
point. Then it warms back to near room temperature where it
is again less dense than the air and lifts off the table (yellow).
Free convection
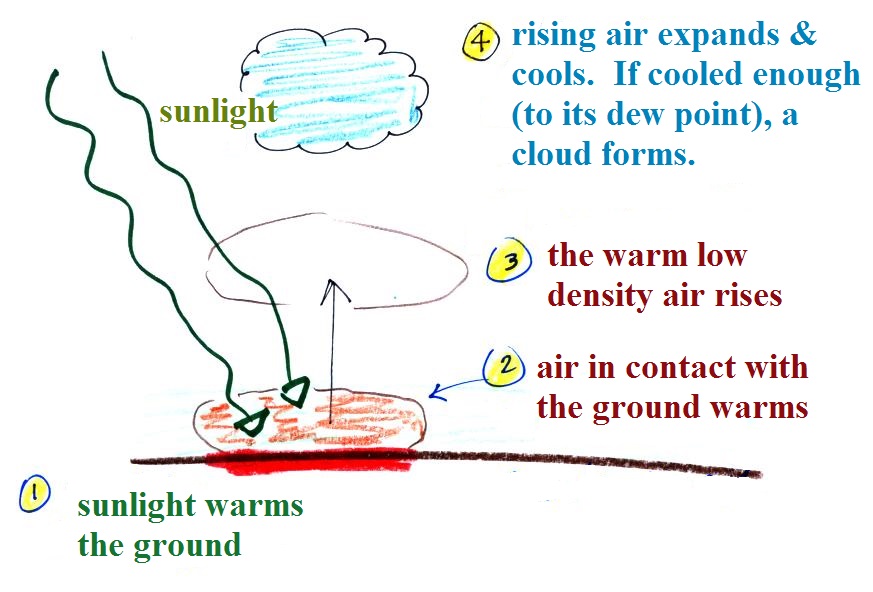
Something like this happens in the atmosphere.
Sunlight shines through the atmosphere. Once it reaches the
ground at (1) it is absorbed and warms the ground. This in
turns warms air in contact with the ground (2) As this air
warms, its density starts to decrease (pressure is staying
constant). When the density of the warm air is low enough,
small "blobs" of air separate from the air layer at the ground and
begin to rise, these are called "thermals." (3) Rising air
expands and cools (we've haven't covered this yet and it might
sound a little contradictory). If it cools enough (to the
dew point) a cloud will become visible as shown at Point 4.
This whole process is called convection; many of our summer
thunderstorms start this way.