Monday Jan. 30, 2006
The Assignment #1 1S1P reports were collected in class today. It
takes
a while to grade these. You should expect to start receiving your
graded reports back sometime next week.
Photocopies of the Practice Quiz Study Guide
were distributed in class
(the Practice Quiz is this coming Wednesday). Note the location
of the
reviews (Mon. and Tue. afternoon 4 - 5 pm) will be Chavez (Econ)
301.
There won't be any questions from the Stratospheric Ozone and the Ozone
Hole section at the end of the study guide.
The Experiment #1 reports are due next Monday. You should bring back
your materials this week so that you can pick up the supplementary
information sheet (which I inadvertently forgot to bring to class on
Mon.)
The Optional Assignment is due on Wednesday.
You'll
find the following material discussed on p. 29 in the photocopied
notes. In 3 simple steps you can understand how a barometer works. It
is probably worth mentioning that barometers are used to measure
pressure (atmospheric pressure). It is not coincidence that the
word bar that appears in barometer
is the same bar that appears in millibars. We will be
learning about weather maps next week and will come across isobars, contours of pressure.
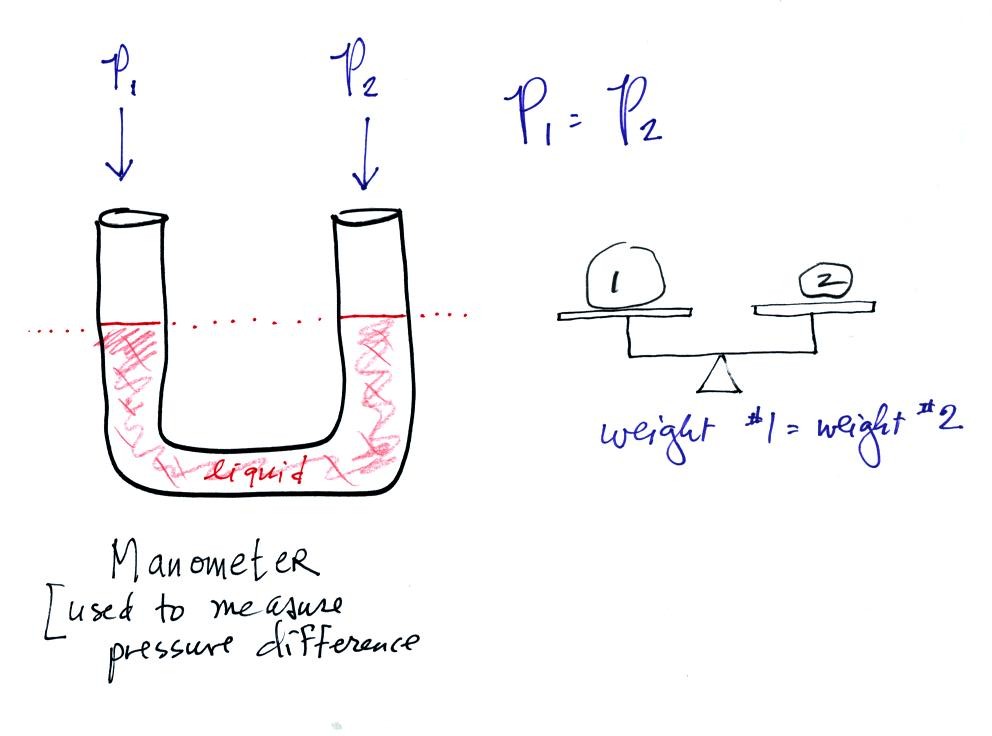
A manometer can be used to measure pressure difference. The
manometer is just a u-shaped tube usually made of glass so that you can
see the liquid that is inside. The liquid can slosh back and
forth just like the pans on a balance can move up and down. A
manometer really behaves just like a pan balance.
In this picture the fact that the liquid levels are the same in the
right and left tubes means P1 and P2 are the same (note you really
don't know what P1 and P2 are, just that they are equal).
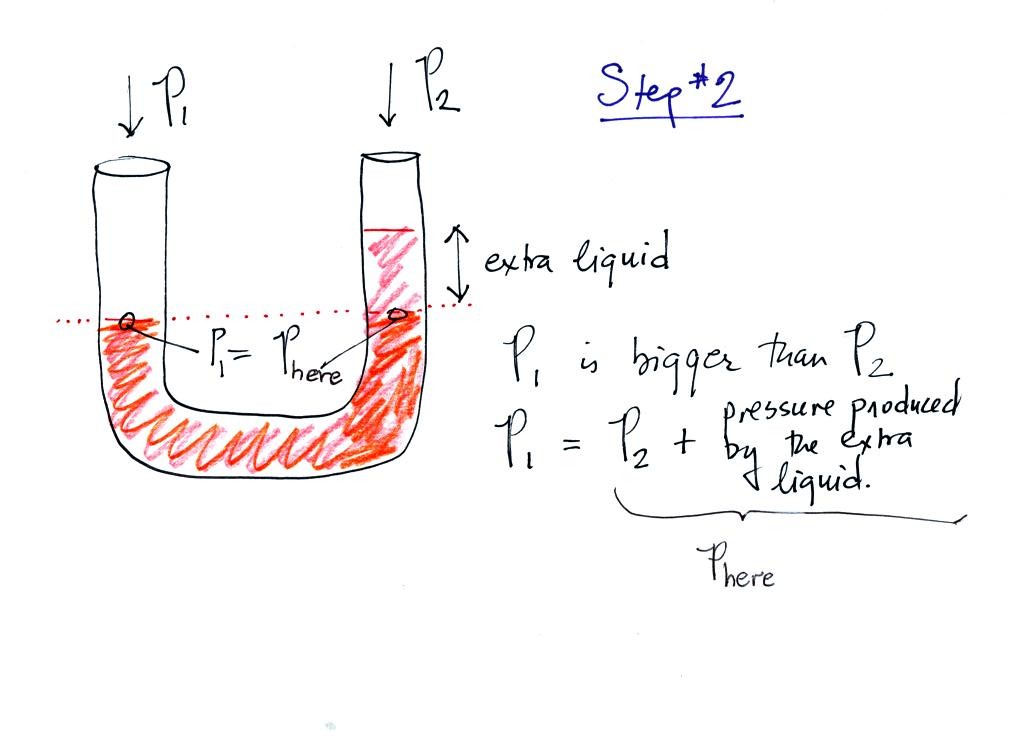
Now the situation is a little different, the liquid levels are no
longer equal. The orange shaded portion of the liquid is the
balance that we had in the previous picture. The pressure P1 is
equal to Phere (the word here was
added after class), the pressure part way up the right
column. P2 is not able by itself to balance P1, P2 is lower than
P1. P1 plus the pressure produced by the column of extra liquid
on the right balances P1. The height of the column of extra
liquid provides a measure of the difference between P1 and P2.
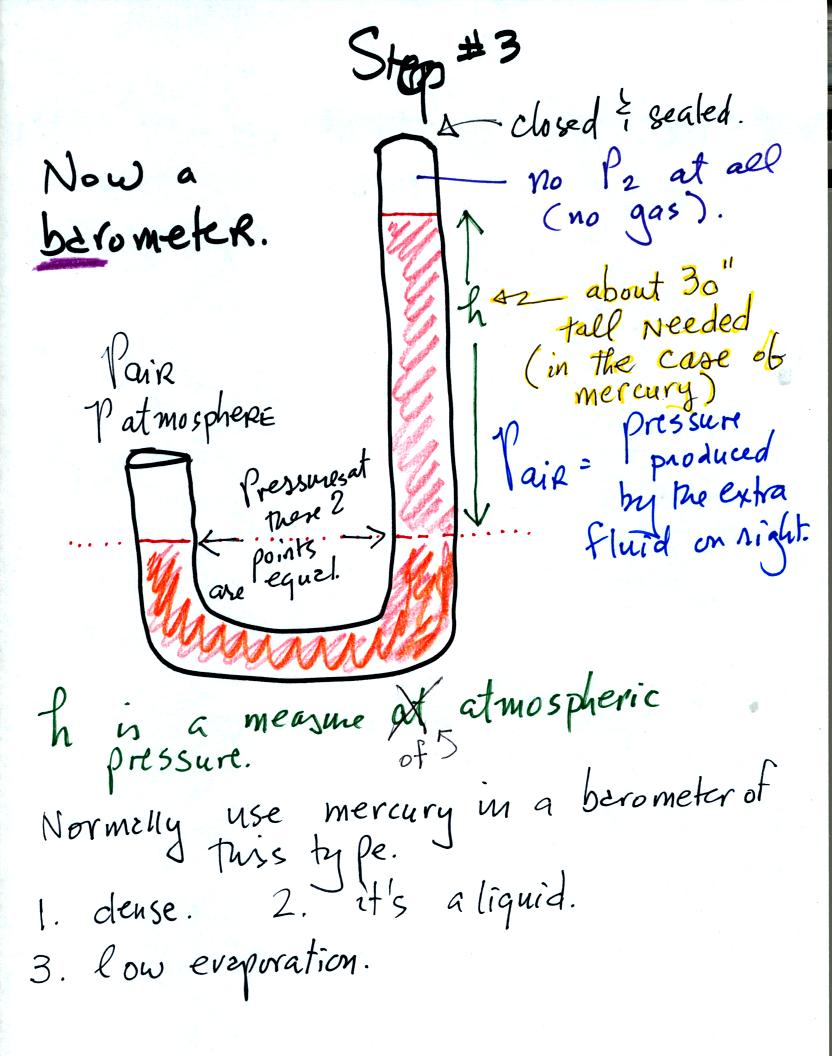
We have changed the manometer by lengthening the right tube and sealing
it off at the top. Air pressure can't get into the right tube any
more. The balance is again shaded in orange at the bottom of the
barometer. Pressures at the two points indicated are equal.
Pair is equal to the pressure produced by the column h inches tall on
the right. If Pair changes, h will change. You now a way of
measuring and monitoring the atmospheric pressure.
Barometers like this are usually filled with mercury. Mercury is
a liquid. You need a liquid that can slosh back and forth in
response to changes in air pressure. Mercury is also dense which
means the barometer won't need to be as tall as if you used something
like water. A water barometer would need to be over 30 feet
tall. With mercury you will need only a 30 inch tall column to
balance the weight of the atmosphere at sea level under normal
conditions (remember the 30 inches of mercury pressure units mentioned
in class a week or so ago). Mercury also has a low rate of
evaporation so you don't have much mercury gas at the top of the right
tube.

Galileo invented the thermometer (I believe). The barometer was
invented in 1643. Not long after that a couple of French
researchers verified that pressure decreased with increasing
altitude.
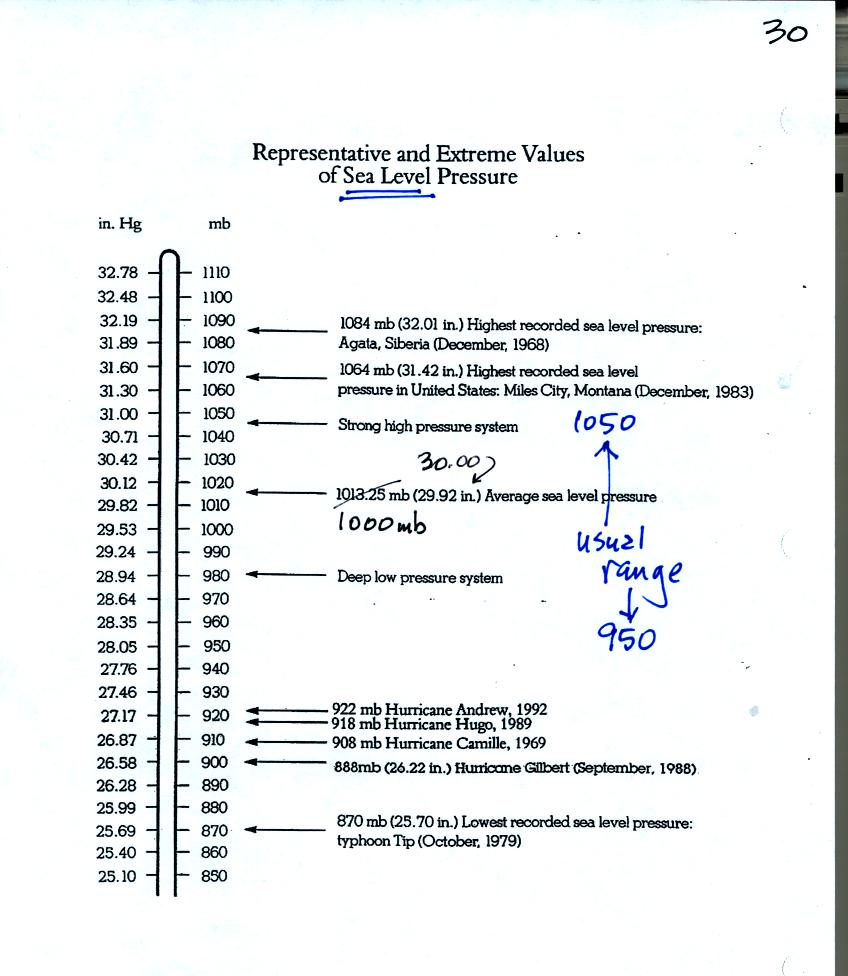
Under normal conditions sea level pressure is about 1000 mb (about 30
inches of mercury). It can be higher and lower than this but
usually falls in the range 950 mb to 1050 mb. Record high and low
sea level pressure values are shown in the chart. Note the record
low values have all be observed in hurricanes.
Now on to
a second way of thinking about air pressure, a sort of microscopic
view. But first (the
picture below was not shown in class)
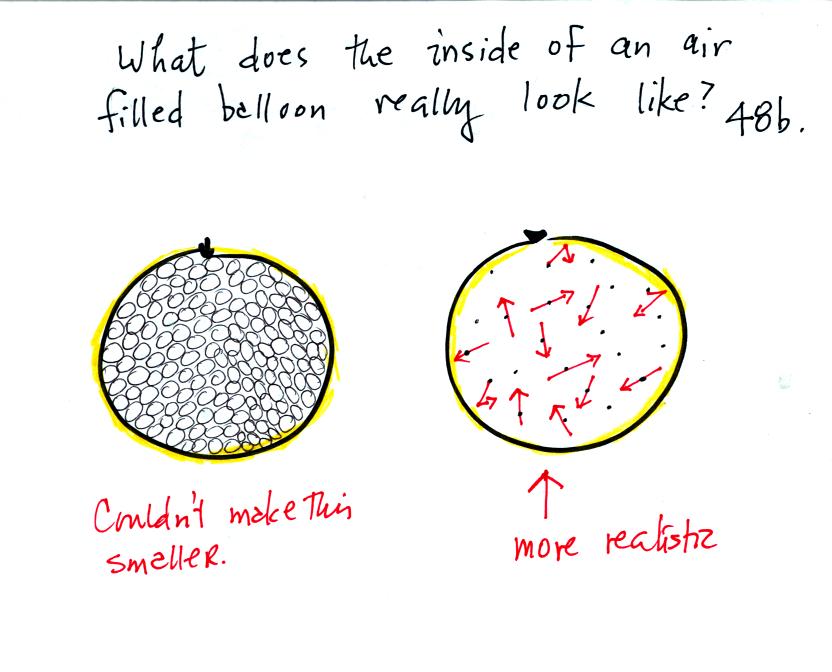
What keeps an air filled balloon inflated? Is it the fact that
the air molecules are like clear glass marbles that just take up all of
the room inside the balloon? If that were true it would be hard
to reduce the volume of the balloon without taking out any of the air
(and we will reduce the volume of a balloon without removing any air
later in the class). Or is the picture on the right, miniscule
air molecules moving around inside the balloon at 100s of MPH and
occasionally colliding with the walls of the balloon and pushing
outward, more accurate?
Click on this molecules
in a gas animation link to see a computer graphics
representation of molecules moving around inside a sealed box.
Next we will see what factors affect the strength of the pressure
produced by moving air molecules inside a balloon.
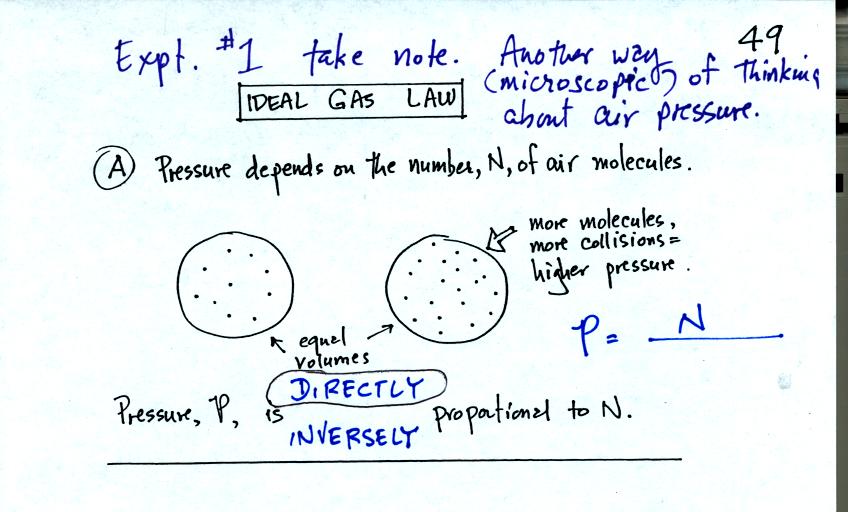
Pressure first depends on the number of air molecules, N.
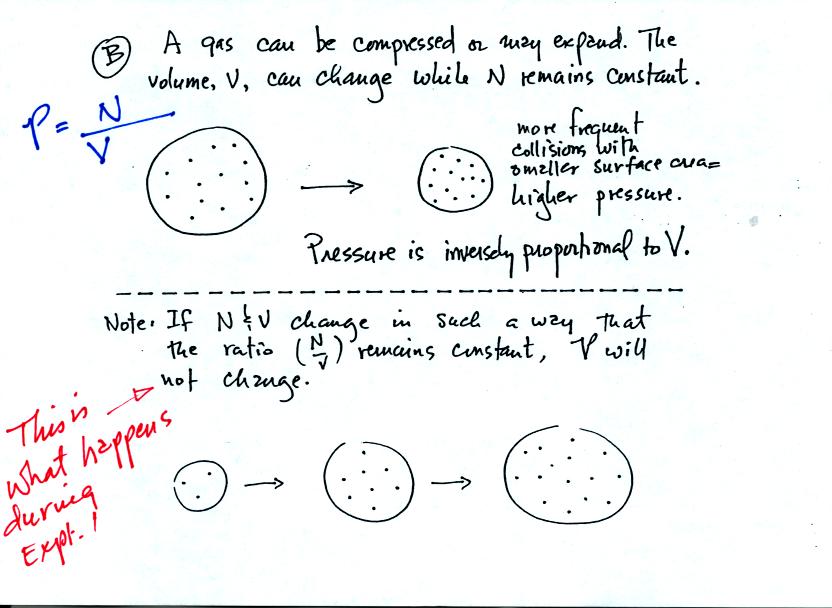
Pressure depends (inversely) on the volume of the balloon. Note the comment in red added after class.
In the Oxygen concentration of the air experiment, the Number of air
molecules in an air sample and the Volume of the air sample will change
together in a way that keeps the air sample Pressure constant.

Pressure
depends on the air's temperature. You shouldn't thrown an aerosol
can into a fire, the fire would heat the gas inside the can, increase
the pressure, and possibly cause the can to explode.

Surprisingly the pressure does not depend on the mass of the
molecules. Pressure doesn't depend on the composition of the
gas. The massive slow moving molecules hit the walls of the
balloon with the same force as the lighter but faster moving molecules.
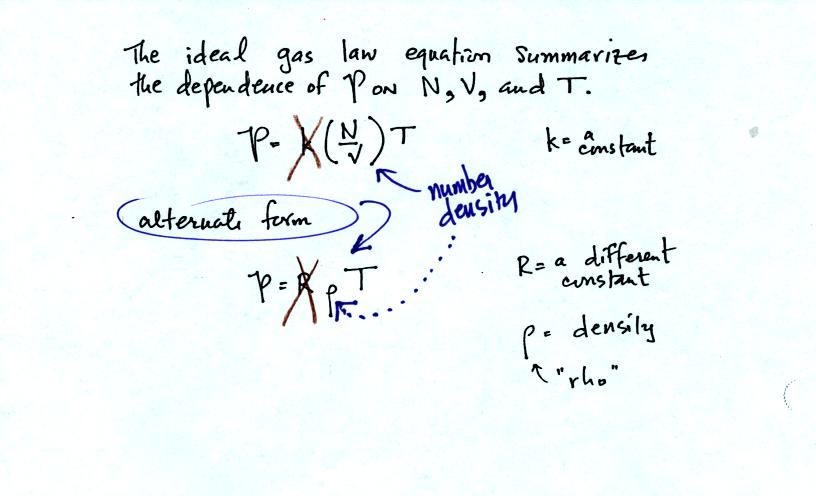
Here are the two ideal gas law equations. You can ignore the
constants k and R if you are just trying to understand how a change in
one of the variables would affect the pressure. You only need the
constants when you are doing a calculation involving numbers.
(1) Pressure = (Number of air molecules) multiplied by temperature divided by volume
or
(2) Pressure = (density) multiplied
by (temperature)
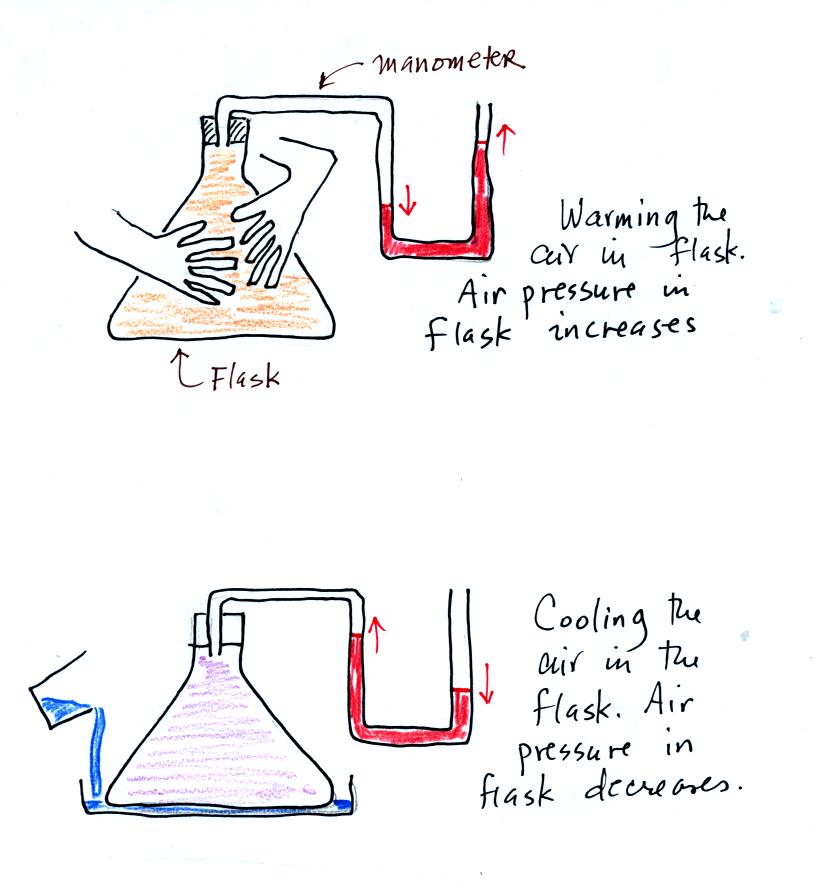
We used a manometer to show how warming the air in a flash (by touching
it with your hands) caused the air pressure in the flask to
increase. Conversely by cooling the air with a little
liquid nitrogen causes the air pressure in the flask to decrease.
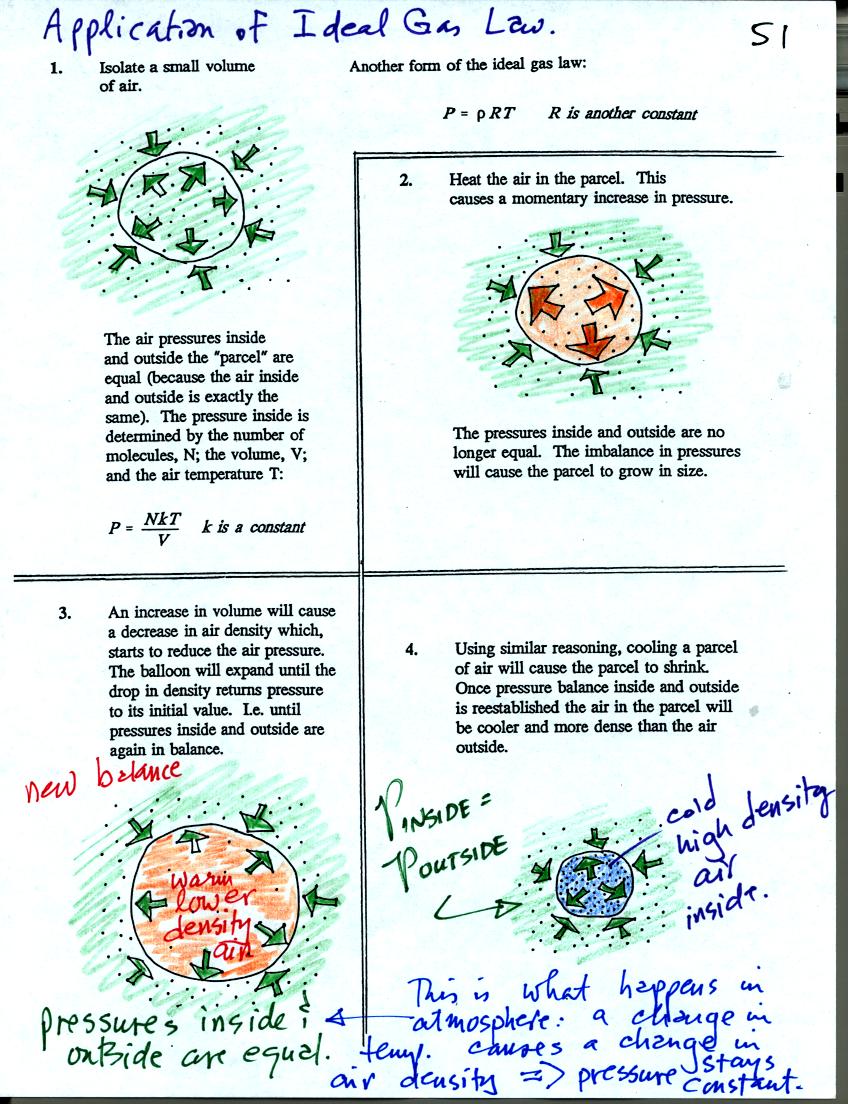
Some of the colors in these
figures have been changed slightly from those in class.
Air in the atmosphere behaves more like the air in a balloon than air
in a flask. A flask doesn't change volume, a balloon can.
We start in the upper left hand corner with air inside a balloon that
is exactly the same as the air outside. The air inside and
outside have been colored green. The arrows show that the
pressure of the air inside pushing outward and the pressure of
the air surrounding the balloon pushing inward are all the same.
Next week warm the air in the balloon. The pressure of the air
increases (just like it did in the flash). The increase is
momentary though. Because the pressure inside is now greater than
the pressure outside the balloon will expand. An increase in
volume will reduce the pressure of the air inside however.
Eventually the balloon will expand enough that the pressures inside and
outside are again in balance. You end up with a balloon of warm
low density air that has the same pressure as the air surrounding it.
You can use the same reasoning to understand that cooling a balloon
will cause its volume to decrease. You will end up with a balloon
filled with cold high density air. The pressures inside and
outside the balloon will be the same.
These associations: warm air
= low density air and cold air = high density air are important and
will come up a lot during the remainder of the semester.
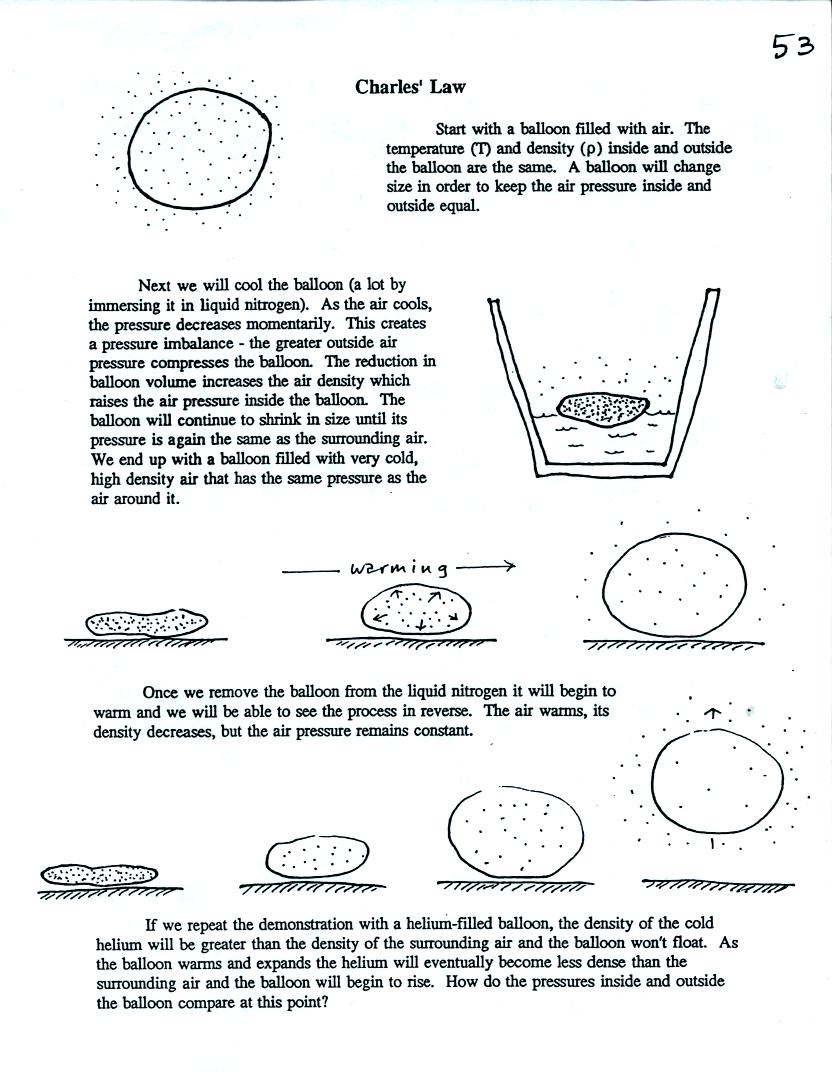
In the
atmosphere air temperature and air density change together in a way
that keeps pressure constant. This is Charles's Law and was
demonstrated at the end of class. The demonstration is
illlustrated and described at the top of p. 53 in the photocopied
notes. A slight modification of the demonstration will be shown
in class on Friday.
I













