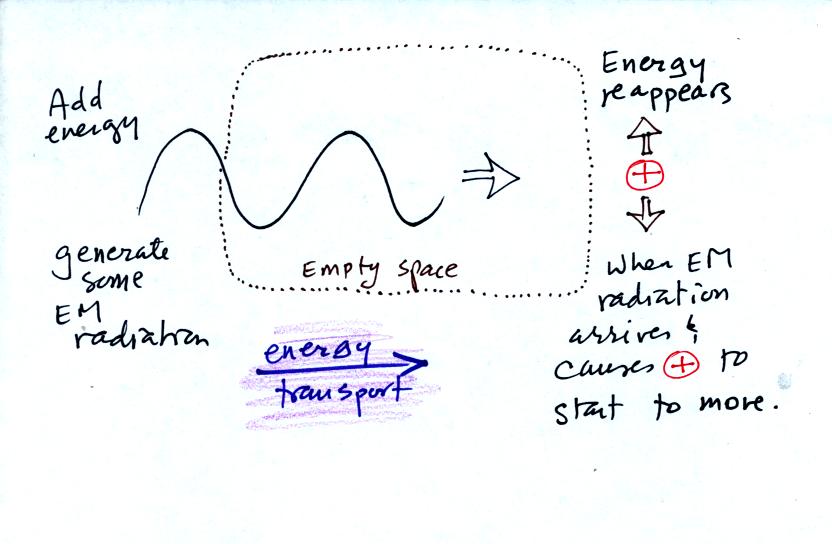
Here's another way of looking at the amount and kind rules that govern the emission of EM radiation.
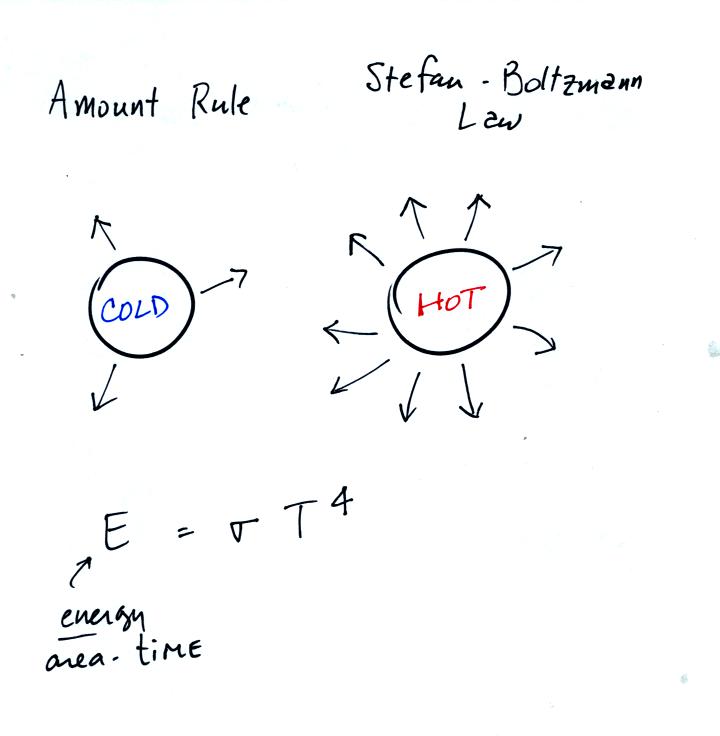
The amount rule is actually called the Stefan-Boltzmann law.
It allows you to calculate the energy emitted per second by a square meter of an object's surface.
(the energy emitted by a square meter of the earth or energy emitted by a square meter of the sun).
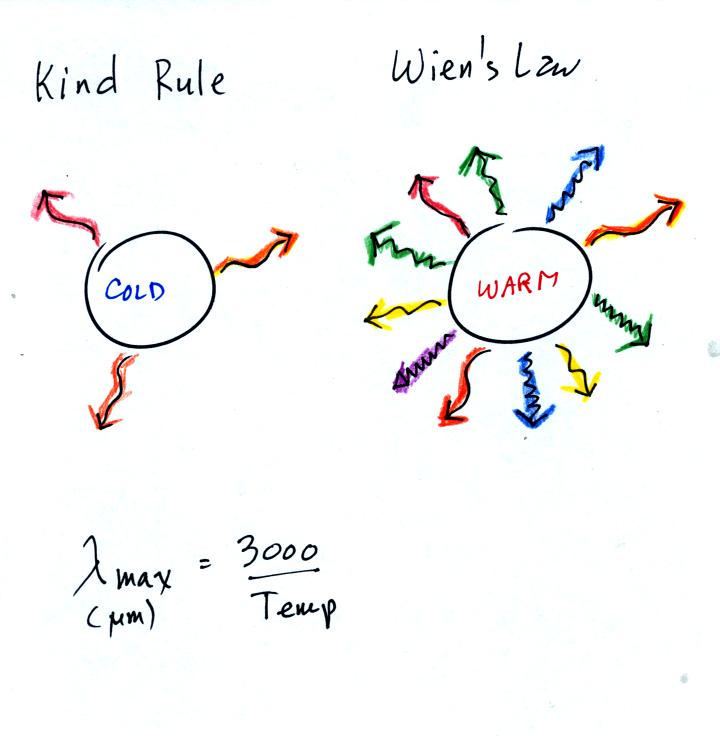
The kind rule (which tells you something about the wavelength of the EM radiation emitted by an object) is actually known as Wien's Law. As an object warms it will begin to emit shorter and shorter wavelengths of light.
The cold object is emitting long wavelength radiation in the example above.
The warm object is emitting some long wavelength radiation but also some shorter wavelength radiation.
The following graphs (at the bottom of p. 65 in the photocopied Class Notes) also help to illustrate the Stefan-Boltzmann law and Wien's law.
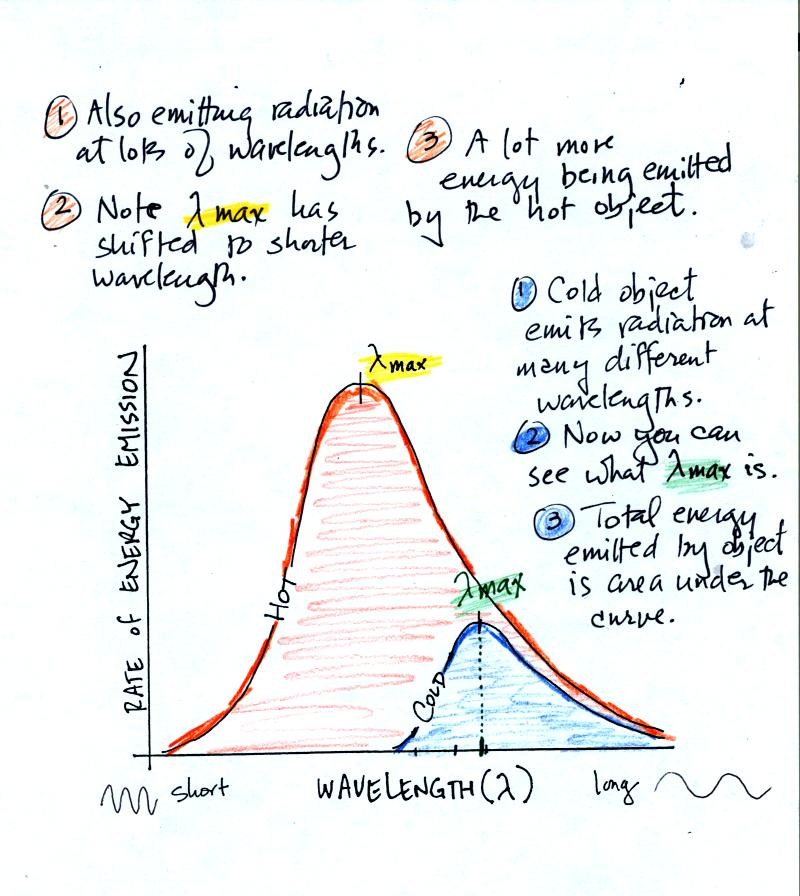
A hot object emits a lot more radiant energy than a cold object. The hot object also emits a lot of shorter wavelength radiation.
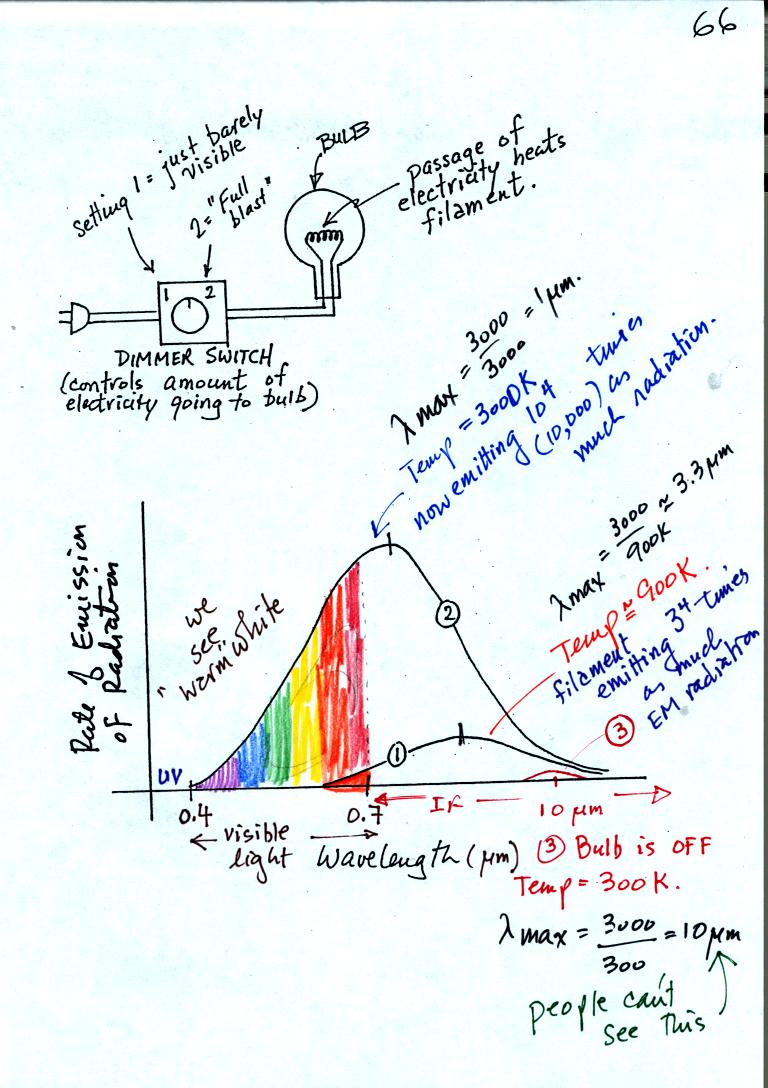
A bulb connected to a dimmer switch can be used to demonstrate the rules above. We'll be interested in the EM radiation emitted by the tungsten filament in the bulb.
We start with the bulb turned off (Point 3 near the bottom right part of the figure above). The filament will be at room temperature which we will assume is around 300 K. The bulb will be emitting radiation (note the small curve above the 10 micrometer mark). The radiation is very weak so we can't feel it. It is also long wavelength far IR radiation so we can't see it. But it is there.
Next we use the dimmer switch to just barely turn the bulb on (the temperature of the filament is now about 900 K). The bulb wasn't very bright at all and had an orange color. This is curve 1 in the figure. Note the far left end of the curve has moved left of the 0.7 micrometer mark - into the visible portion of the spectrum. That is what you are able to see the small, the portion of the radiation emitted by the bulb that is visible light (but just long wavelength red and orange light). Most of the radiation emitted by the bulb is to the right of the 0.7 micrometer mark and is invisible IR radiation (it is strong enough now that you could feel it though).
Finally we turn on the bulb completely. The filament temperature is now about 3000K. The bulb is emitting a lot more visible light, all the colors, though not all in equal amounts. The bulb was also much brighter. The mixture of the colors produces a warm white light. It is warm because it is a mixture that contains a lot more red, orange, and yellow than blue, green, and violet light. It is interesting that most of the radiation emitted by the bulb is still in the IR portion of the spectrum (lambda max is 1 micrometer). This is invisible light. A tungsten bulb like this is not especially efficient, at least not as a source of visible light.
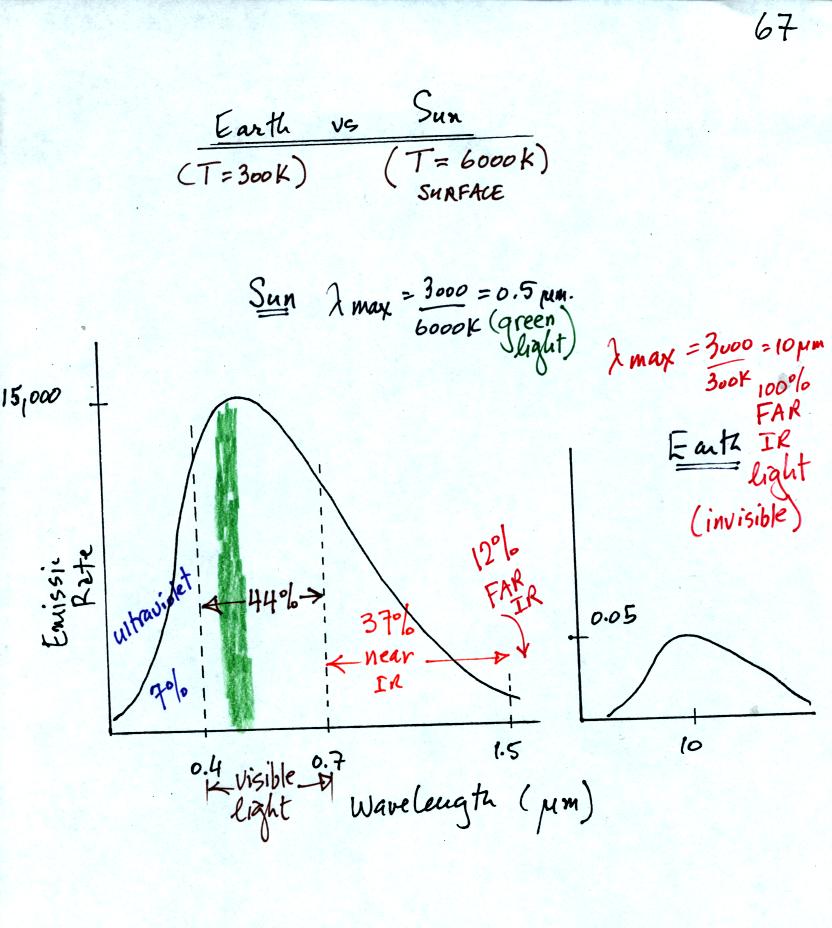
This figures compares the EM radiation emitted by the earth and the sun. First because the sun (surface of the sun) is 20 times hotter than the earth a square meter of the sun's surface emits energy at a rate that is 160,000 times higher than the earth. Lambda max for the sun is 0.5 micrometers, green light. The sun emits more green light than any other kind of light. The sun doesn't appear green because it is also emitting lesser amounts of violet, blue, yellow, orange, and red - together this mix of colors appears white. 44% of the radiation emitted by the sun is visible light, 49% is IR light (37% near IR + 12% far IR), and 7% is ultraviolet light.
100% of the light emitted by the earth is invisible IR light. The wavelength of peak emission for the earth is 10 micrometers. Note the vertical scale on the earth curve is different than on the sun graph. If both the earth and sun were plotted with the same vertical scale, the earth curve would be too small to be seen.
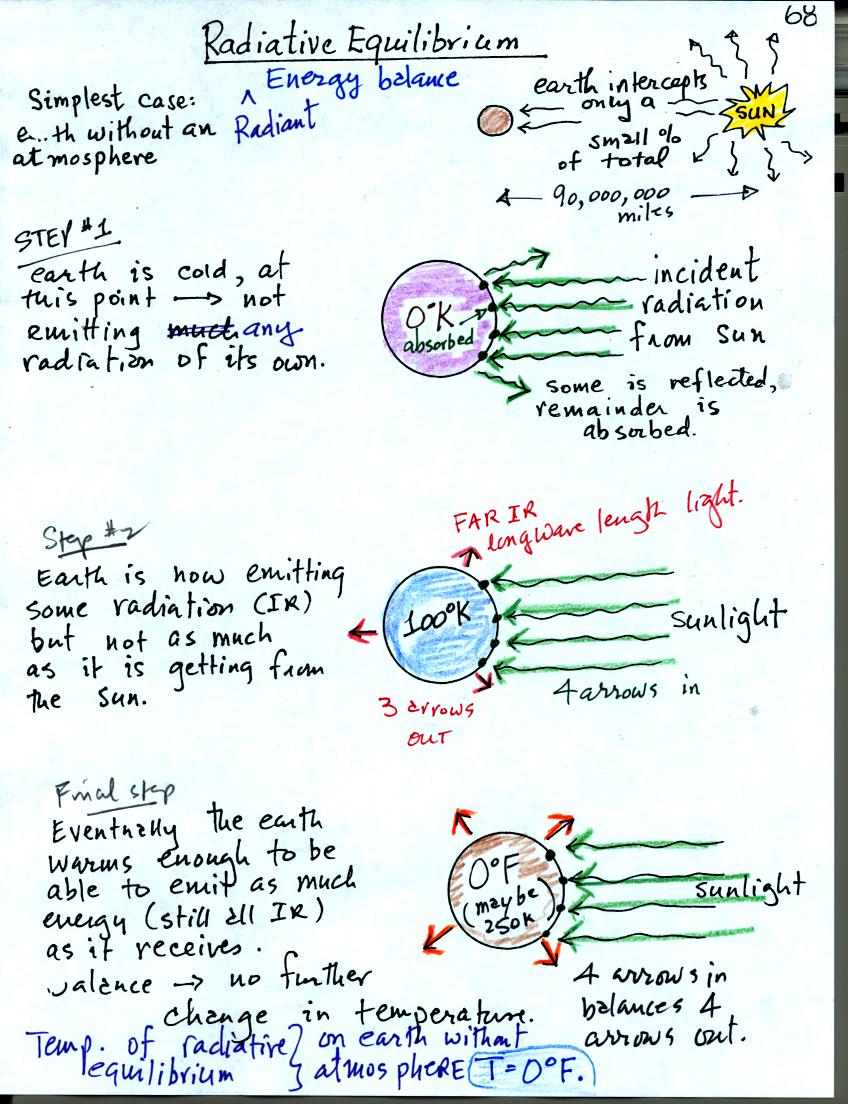
Energy balance on the earth without an atmosphere. The earth (shaded violet) starts out very cold and is not emitting any EM radiation at all. It is absorbing sunlight however so it will warm. Once the earth starts to warm it will begin to emit EM radiation, though not as much as it is getting from the sun (the slightly warmer earth is now colored blue). Eventually it will warm enough that the earth (now shaded brown) will emit the same amount of energy (though not the same wavelength energy) as it absorbs from the sun. This is radiative equilibrium. The temperature at which this occurs is 0 F (on the earth without any atmosphere). That is called the temperature of radiative equilibrium.
Before we move to the more complex situation of radiative equilibrium on the earth with an atmosphere. We'll see how the atmospheric greenhouse effect works. Before doing that we will need to learn something about how gases in the atmosphere affects or filters different kinds of light that passes through the atmosphere.