Thursday Oct. 24, 2013
Portland Oregon seems to turn out a lot of good musical
groups. Black Prairie was featured today and you heard some
of the following: "Little Song
bird", "Dirty
River Stomp", "A Prairie
Musette", "Taraffa",
"How Do You
Ruin Me"
The Experiment #2 reports
have been graded and were returned in class today. You
may revise your report if you want to (a revision isn't
required). Revised reports are due in two weeks (Thu., Nov.
7). Please return your original report with your revised
report.
1S1P Assignment #2 now
contains 3 topics. If you write a report on either or both
of the first two topics, your report(s) are due by next
Tuesday, Oct. 29. The 3rd topic will take a little more work
and isn't due until Tue., Nov. 5. Remember you can write a
total of 2 reports as part of Assignment #2.
A new Optional
Assignment on The Controls of
Temperature (Climate) is now available. The assignment
is due Thu., Oct. 31. Watch out for another humidity example
problems Optional Assignment that will appear later today or
sometime on Friday. It will probably also be due on Oct. 31.
There are still a few sets of Expt. #3 materials and one or two
sets of Expt. #4 materials. You should now have completed or
being working on either an experiment, book report or scientific
paper report.
We needed to finish up a few loose ends from the section
on the Greenhouse Effect before moving onto something new.
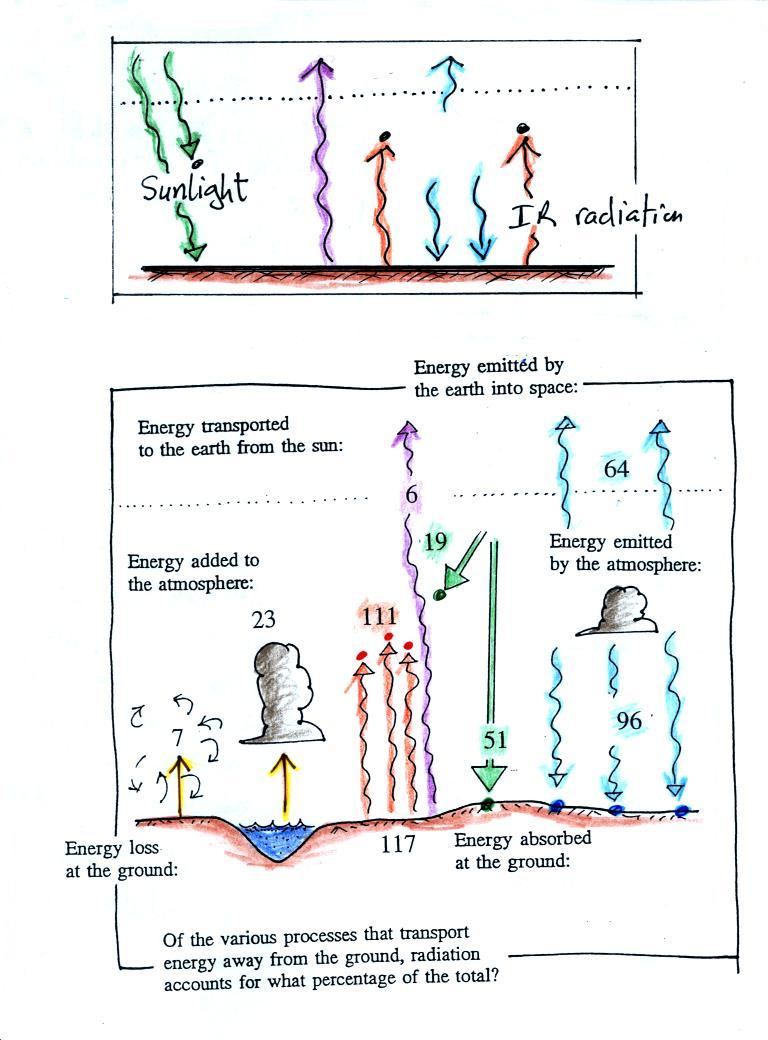
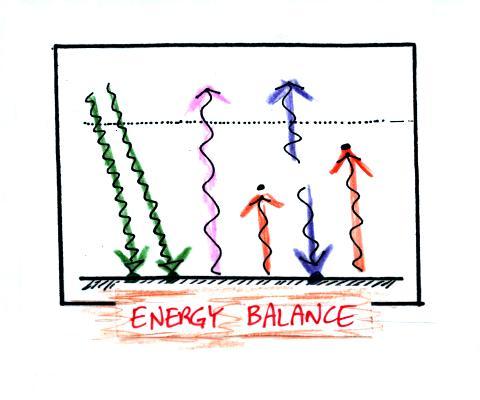
A more realistic picture of energy balance on the earth is shown
below (the bottom figure below). The simplified version that
we just worked out earlier in the class is also shown for
comparison (top figure). Incidentally the top figure is the
answer to the Optional Assignment assigned at the end of class on
Tuesday (you needed to add the 3 bluish arrows being emitted by
the atmosphere: one went upward & into space, the other two
downward to the ground).
In the top figure (the simplified representation of energy
balance) you should recognize the incoming sunlight (green), IR
emitted by the ground that passes through the atmosphere (violet),
IR radiation emitted by the ground that is absorbed by greenhouse
gases in the atmosphere (orange) and IR radiation emitted by the
atmosphere (blue).
The lower part of the figure is pretty complicated.
It would be difficult to start with this figure and find the
greenhouse effect in it. That's why we used a simplified
version. Once you understand the upper figure, you should be
able to find and understand the corresponding parts in the lower
figure (especially since I've tried to use the same colors for
each of the corresponding parts).
Some of the incoming sunlight (51 units in green) reaches the
ground and is absorbed. 19 units of sunlight are absorbed by
gases in the atmosphere. The 30 units of reflected sunlight
weren't included in the figure.
The ground emits a total of 117 units of IR light. Only 6
shine through the atmosphere and go into space. The
remaining 111 units are absorbed by greenhouse gases.
There were a few things I wanted you to notice in the bottom
figure.
1. Note that the ground is
emitting more energy (117 units) than it gets from the sun (51
units). It is able to achieve energy balance because it also
gets energy from the atmosphere (96 units). That's thanks to
the greenhouse effect. If you're really paying attention you
would notice that 117 units emitted doesn't balance 96 + 51 = 147
units absorbed. The surface is emitting 117 units but an
additional 30 units are being carried from the ground to the
atmosphere by conduction, convection, and latent heat (at the far
left of the figure). That brings everything into balance
(117 + 30 = 147).
2. The ground is actually receiving
more energy from the atmosphere (96 units) than it gets from the
sun (51 units)! Doesn't that seem odd? I think the
main reason for this is that the sun just shines for part of the
day. We receive energy from the atmosphere 24 hours per day.
3. The atmosphere is emitting energy upward into
space (64 units) and downward toward the ground (96 units).
Why are the amounts different? One reason might be
that the lower atmosphere is warmer than the upper atmosphere
(warm objects emit more energy than cold objects). But I
think a bigger part of the explanation is probably that there is
more air in the bottom of the atmosphere (the air is denser) than
near the top of the atmosphere. It is the air in the
atmosphere that is emitting radiation. More air = more
emission.
Last. The amount of energy transported by
conduction, convection, and latent heat (the 7 + 23 units at left)
is pretty small compared to what is transported in the form of EM
radiation.
We can use our simplified representation of
the greenhouse effect to understand the effects of clouds on
daytime and nighttime temperatures. The following can be
found on pps. 72a & 72b in the ClassNotes (I've rearranged
things slightly to try to make it clearer)
Here's the simplified picture of
radiative equilibrium again (that you're probably getting pretty
tired of seeing). You should be able to say
something about every arrow in the picture. The two
pictures below show what happens at night when you remove
the two green rays of incoming sunlight.
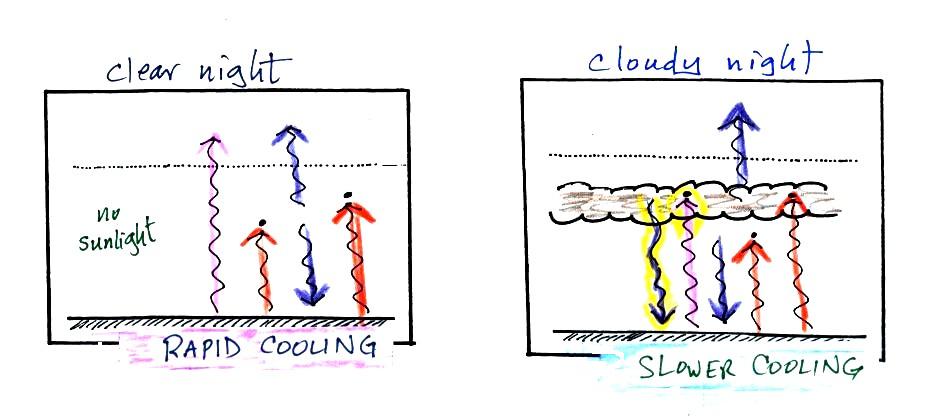
Note first of all that neither picture is in radiative
equilibrium. The picture on the left shows a clear
night. The ground is losing 3 arrows of energy and getting
one back from the atmosphere. That's a net loss of 2
arrows. The ground cools rapidly and gets cold during the
night.
A cloudy night is shown at right. Notice the effect of
the clouds. Clouds are good absorbers of far infrared radiation (10 μm
wavelength). They reflect near IR light (1 μm wavelength)
just like they do visible light. If we could see 10
μm far IR light, clouds would appear black, very different from
what we are used to (because clouds also emit IR light, the
clouds might also glow). Because of the clouds none of the
IR radiation emitted by the ground passes through the atmosphere
into space. It is all absorbed either by greenhouse gases
or by the clouds. Because the clouds and atmosphere are
now absorbing 3 units of radiation they must emit 3 units: we'll
draw 1 going upward into space, the other 2 downward to the
ground.
There is still a net loss of energy at the ground on the cloudy
night but it's smaller, only 1 arrow. The
ground won't cool as quickly and won't get as cold on a cloudy
night as it does on a clear night. That makes for somewhat
warmer early morning bicycle rides this time of the year.
The next two figures compare clear and cloudy days.

Clouds are good reflectors
of visible light
(we see visible light and clouds appear white). The effect
of this is to reduce the amount of sunlight energy reaching the
ground in the right picture. With less sunlight being
absorbed at the ground, the ground doesn't need to get as warm to
be in energy balance.
It is generally cooler during the day on a cloudy day than on a
clear day.
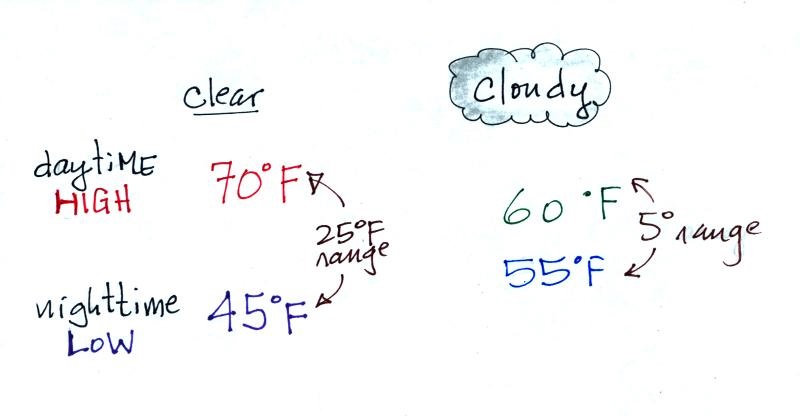
Clouds raise the nighttime minimum temperature and lower the
daytime maximum temperature. Here are some typical daytime
high and nighttime low temperature values on clear and cloudy days
for this time of the year.
We can use our simplified representation of radiative
equilibrium to understand enhancement of the greenhouse effect and
global warming.
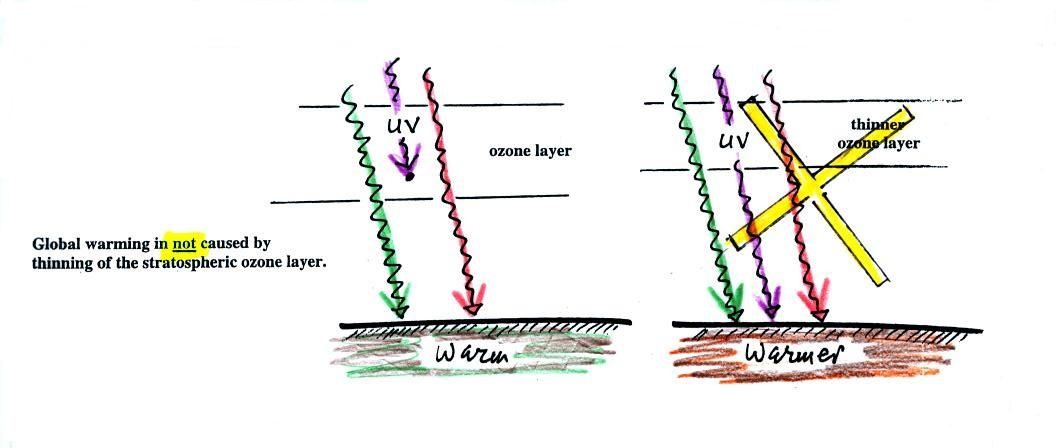
But first, a common misconception about the cause of global
warming.
Many people know that sunlight contains UV light and that the
ozone absorbs much of this dangerous type of high energy
radiation. People also know that release of chemicals such
as CFCs are destroying stratospheric ozone and letting some of
this UV light reach the ground. That is all correct.
They then conclude that it is this additional UV energy
reaching the ground that is causing the globe to warm. This is not correct.
There isn't much UV light in sunlight in the first place (only
about 7% of sunlight is UV) and just a portion of that would reach
the ground. The small amount of additional UV light reaching
the ground won't be enough to cause global warming. It will
cause cataracts and skin cancer and those kinds of problems but
not global warming.
If all 7% of the UV light in sunlight were to reach the ground
it probably would cause some warming. But it probably
wouldn't matter because some of the shortest wavelength and most
energetic forms of UV light would probably kill us and most other
forms of life on earth.
Here's the cause of global warming and the reason for concern
The figure (p. 72c in the photocopied Class Notes) on the left
shows energy balance on the earth without an atmosphere (or with
an atmosphere that doesn't contain greenhouse gases). The
ground achieves energy balance by emitting only 2 units of energy
to balance out what it is getting from the sun. The ground
wouldn't need to be very warm to do this, only 0 F.
If you add an atmosphere and greenhouse gases, the atmosphere
will begin to absorb some of the outgoing IR radiation. The
atmosphere will also begin to emit IR radiation, upward into space
and downward toward the ground. After a period of adjustment
you end up with a new energy balance. The ground is warmer
and is now emitting 3 units of energy even though it is only
getting 2 units from the sun. It can do this because it gets
a unit of energy from the atmosphere. This is what I refer
to as the beneficial greenhouse effect. It makes the earth
more habitable (average surface temperature of 60 F versus the 0 F
without a greenhouse effect).
In the right figure the concentration of greenhouse gases has
increased even more (due to human activities). The earth
might find a new energy balance. In this case the ground
would be warmer and would be emitting 4 units of energy, but still
only getting 2 units from the sun. With more greenhouse
gases, the atmosphere is now able to absorb 3 units of the IR
emitted by the ground. The atmosphere sends 2 back to the
ground and 1 up into space.
Now a new block of material - something we should have started
last Tuesday. Here's an introduction to the first portion:
humidity (moisture in the air). This topic and
the terms that we will be learning are probably new and might be
confusing. That's the reason for this introduction. We
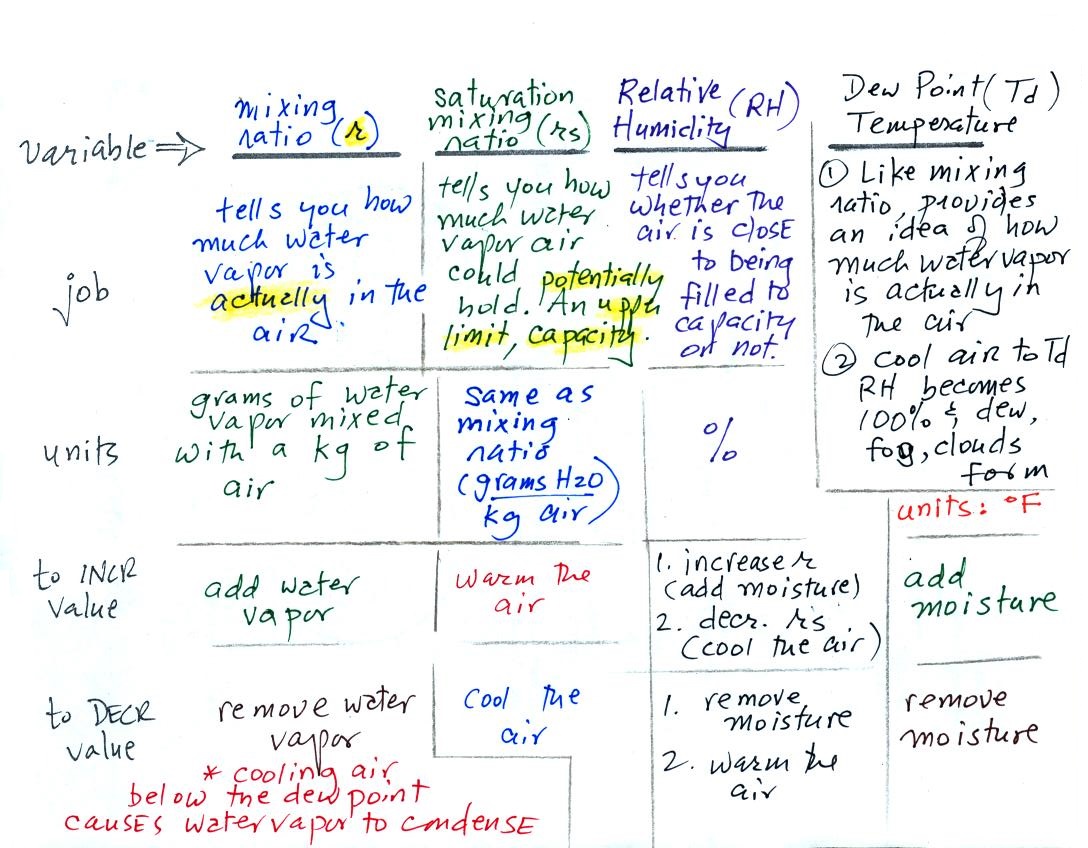
will be mainly be interested in 4 variables:
Your task will be to learn the "jobs" of these variables, what
they're good for, and what can cause them to change value.
A handout was distributed in class. On one side was room to
add or organize some of the new material we'll be covering.
You'll find a copy of that at the end of today's notes. An In-Class
Optional Assignment was on the back of the handout.
You were supposed to complete and turn in the assignment at the
end of class. If you weren't in
class and want to download and complete the assignment,
then turn it in at the beginning of class next Tuesday you can
receive at least partial (and probably full) credit for the
assignment.
The bottom half of the figure below can be found on p. 83 in
the ClassNotes.
Mixing ratio tells you how much water vapor is actually
in the air. You can think of it as just a number: when the
value is large there's more water vapor in the air than when the
value is small. Mixing ratio has units of grams of water
vapor per kilogram of dry air (the amount of water vapor in grams
mixed with a kilogram of dry air). A kilogram of air is
about one cubic meter of air (about one cubic yard of air).
Mixing ratio is basically the same
idea as teaspoons of sugar mixed in a cup of tea.

The value of the mixing
ratio won't change unless you add water vapor to or remove
water vapor from the air. Warming the air won't change
the mixing ratio. Cooling the air won't change the
mixing ratio (with
one exception - when the air is cooled below its
dew point temperature and water vapor starts to
condense). Since the mixing ratio's job is to tell you
how much water vapor is in the air, you don't want it to
change unless water vapor is actually added to or removed
from the air.
Saturation mixing ratio is just an upper
limit to how much water vapor can be found in air,
the air's capacity for water vapor.
It's a property of air and depends on the air's temperature; warm
air
can potentially hold more water vapor
than cold air. It doesn't say anything about
how much water vapor is actually in the air (that's the mixing
ratio's job). This variable has the same
units: grams of water vapor per kilogram of dry air.
Saturation mixing ratio values for different air temperatures
are listed and graphed on p. 86 in the ClassNotes.
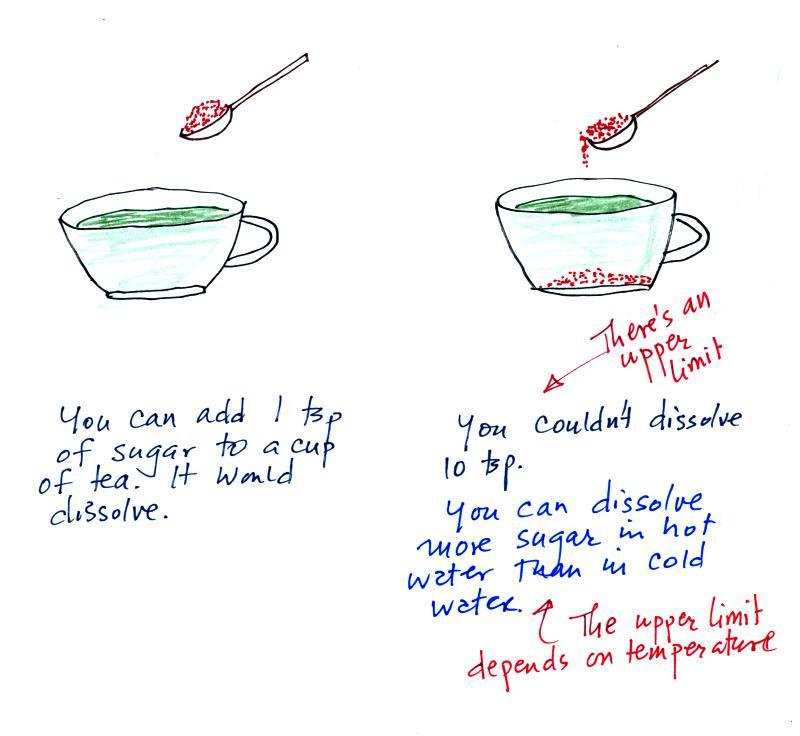
The sugar dissolved in tea
analogy is still helpful. Just as is the case with water
vapor in air, there's a limit to how much sugar can be
dissolved in a cup of hot water. And not only that, the
amount depends on temperature: you can dissolve more sugar in hot
water than in cold water.
The dependence of saturation mixing ratio on air
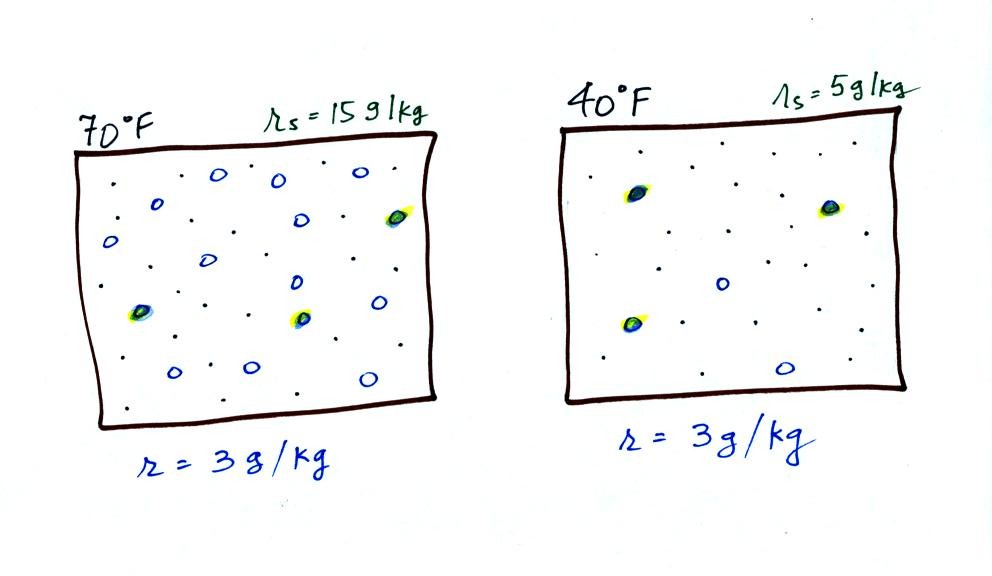
temperature is illustrated below:
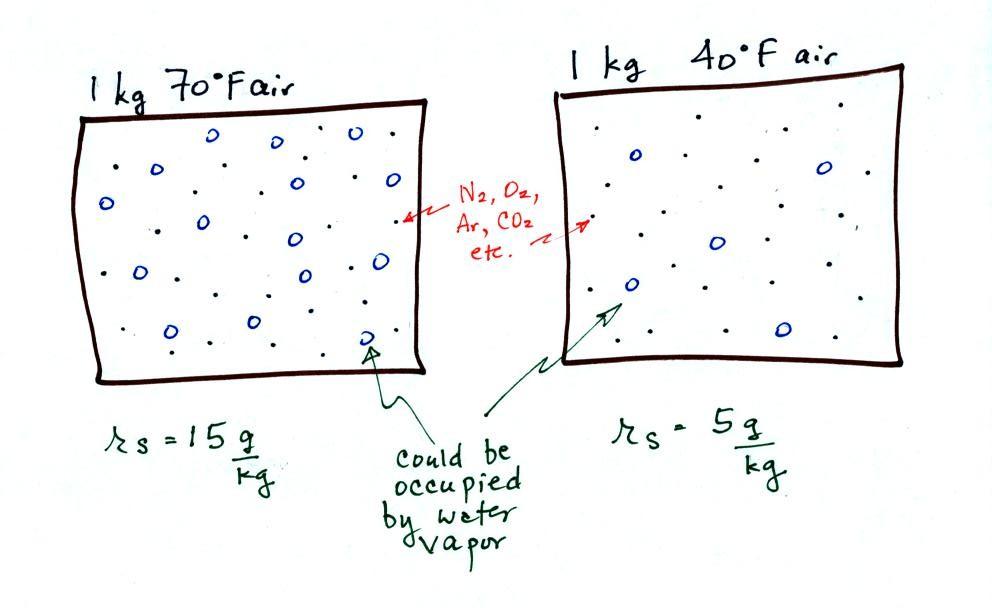
The small specks represent all
of the gases in air except for the water vapor. Each of
the open circles represents 1 gram of water vapor
that the air could potentially hold. There are 15 open
circles drawn in the 1 kg of 70 F air; each 1 kg of 70 F air
could hold up to 15 grams of water vapor. The 40 F air
only has 5 open circles; this cooler air can only
hold up to 5 grams of water vapor per kilogram of dry air.
The numbers 15 and 5 came from the table on p. 86.
Now we have gone and actually put some water vapor into
the volumes of 70 F and 40 F air (the open circles are colored
in). The same amount, 3 grams of water vapor, has been added
to each volume of air. Three of the open circles have been
colored in. The mixing ratio, r, is 3 g/kg in both cases.
After looking at the figure above you might start to guess at what
relative humidity might mean.
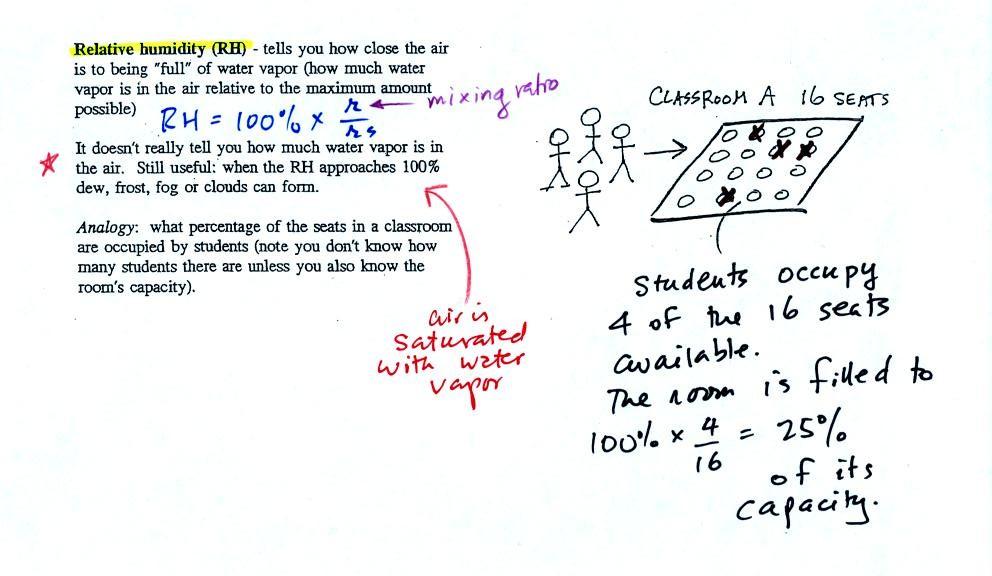
The relative humidity is the variable most people are
familiar with. It tells you how "full" the air is with water
vapor, how close it is to being filled to capacity with
water vapor, how close the air is to being "saturated" with
water vapor.
In the analogy (sketched on the right hand side of p. 83 in the
photocopied notes) 4 students wander into Classroom A which has 16
empty seats. Classroom A is filled to 25% of its
capacity. You can think of 4, the actual number
of students, as being analogous to the mixing ratio. The
classroom capacity is analogous to the saturation mixing
ratio. How full the room is is analogous to the relative
humidity.
The figure below goes back to the volumes (1 kg each) of 70 F
and 40 F air that could potentially hold 15 grams or 5 grams of
water vapor.
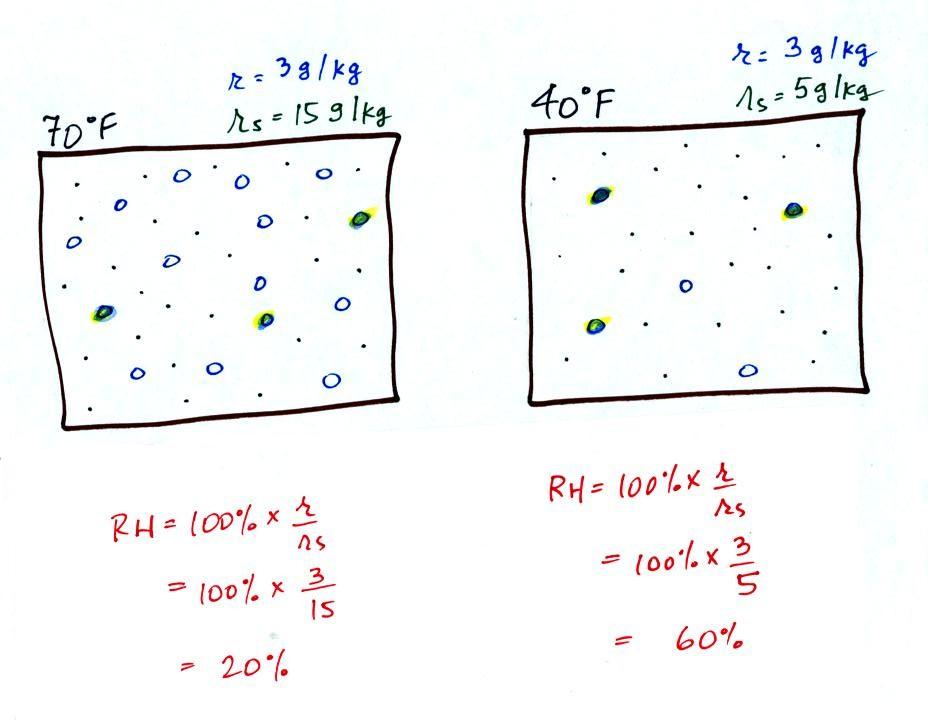
Something important to note: RH
doesn't really tell you how much water vapor is actually in the
air. The two volumes of air above contain the same
amount of water vapor (3 grams per kilogram) but have very
different values of relative humidity. You could just as
easily have two volumes of air with the same relative humidity but
different actual amounts of water vapor.
What is the RH good for if it doesn't tell you how much moisture
is in the air? When the RH reaches 100% dew, fog, and clouds
form. RH tells you whether clouds or fog are about to form
or not.

|
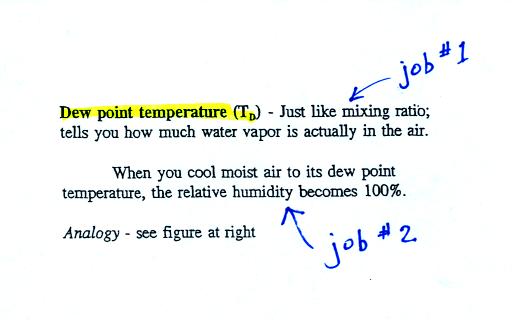 |
The dew point temperature has two jobs. First it
gives you an idea of the actual
amount of water vapor in the air. In this respect
it is just like the mixing ratio. If the dew point
temperature is low the air doesn't contain much water vapor.
If it is high the air contains more water vapor. This is
something we learned early in the semester.
Second the dew point tells you
how much you must cool the air in order to cause the RH to
increase to 100% (at which point a cloud, or dew or
frost, or fog would form). This idea of cooling the air
until the RH increases to 100% is important and is something we
will use a lot.
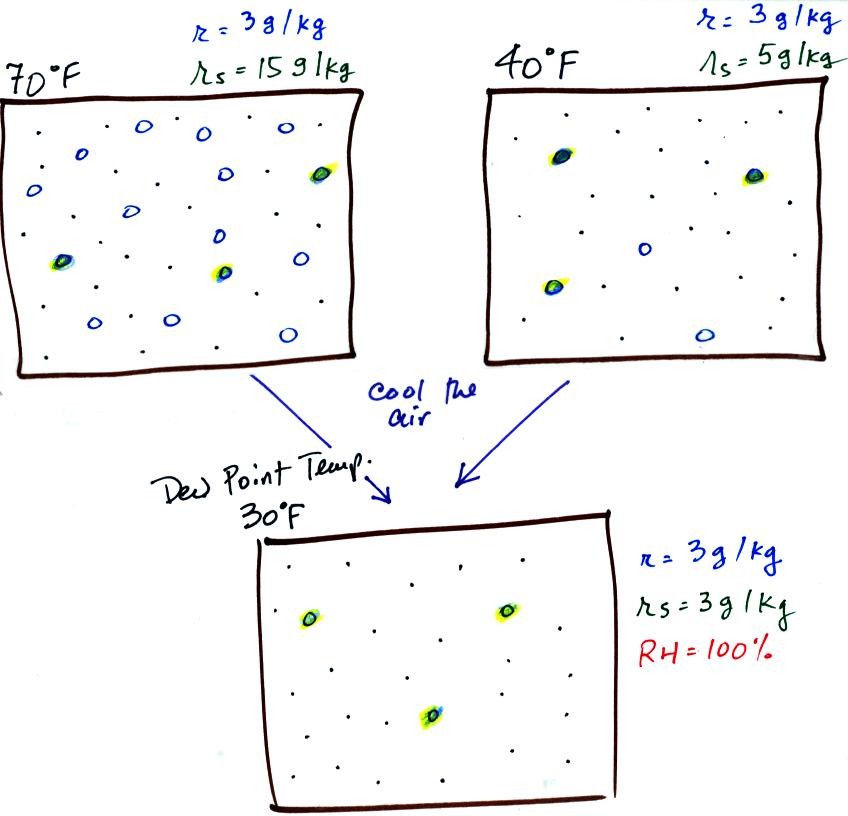
I don't think I showed this figure in
class. If we cool the 70 F air or the 40 F
air to 30 F we would find that the saturation mixing ratio would
decrease to 3 grams/kilogram. Since the air actually
contains 3 g/kg, the RH of the 30 F air would become 100%.
The 30 F air would be saturated, it would be filled to capacity
with water vapor. 30 F is the dew point temperature for 70 F
air that contains 3 grams of water vapor per kilogram of dry
air. It is also the dew point temperature for 40 F air that
contains 3 grams of water vapor per kilogram of dry air.
Because both volumes of air had the same amount of water
vapor, they both also have the same dew point
temperature.
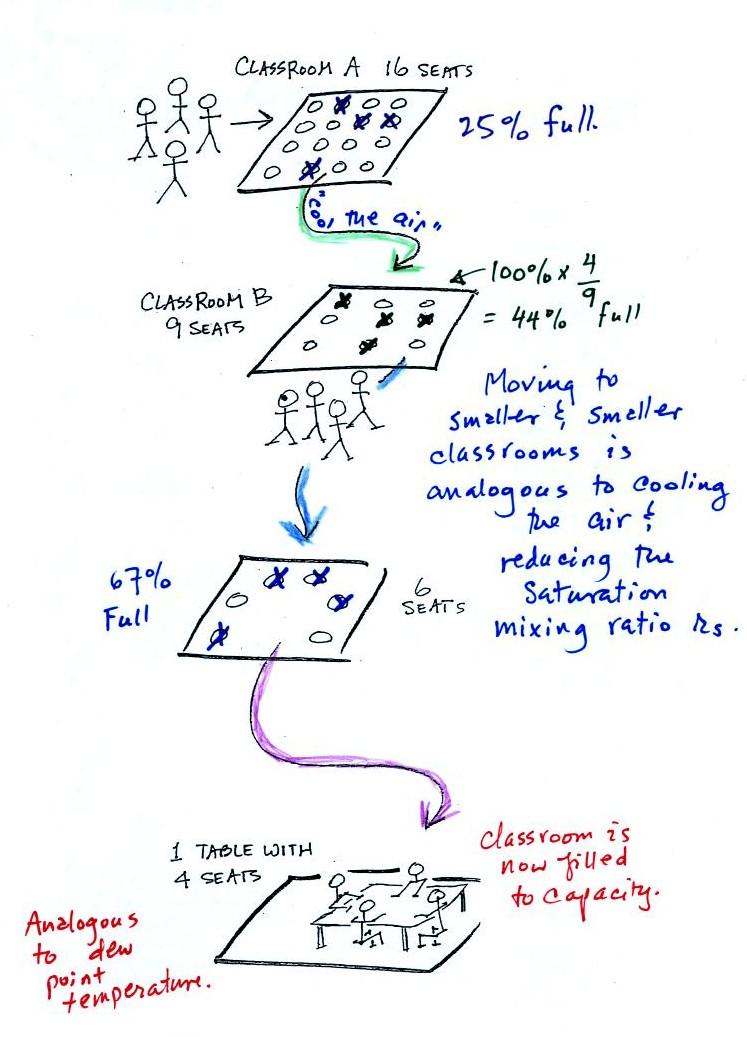
Now back to the
student/classroom analogy (I'm
sure I didn't show this figure in class)
The 4 students move into classrooms of smaller and smaller
capacity. The decreasing capacity of the classrooms is
analogous to the decrease in saturation mixing ratio that occurs
when you cool air. Eventually the students move into a
classroom that they just fill to capacity. This is
analogous to cooling the air to the dew point.
We're going to work 4 example problems. It's by doing
these problems that you start to understand how these various
variables work and what can cause them to change value.
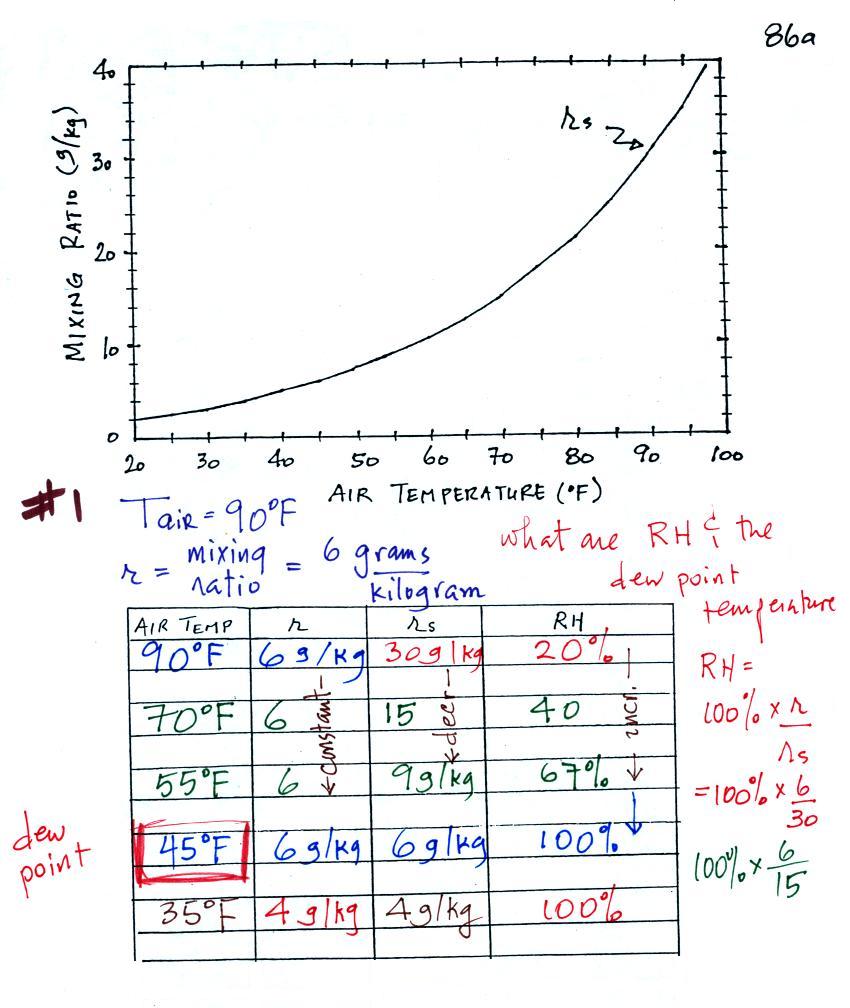
Example #1
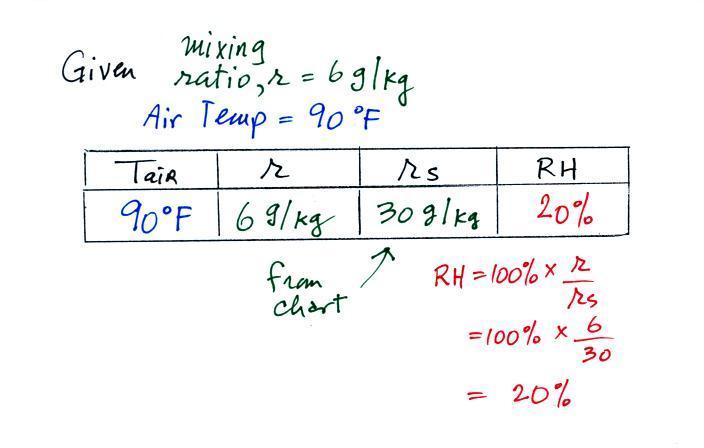
In this example you are given an air temperature
of 90 F and a mixing ratio value of 6 g/kg. You are supposed
to determine the mixing ratio and the dew point temperature.
Here's something close to what we ended up with. This would
be hard enough to sort out even if you were in class. So
we'll work through this problem in a more detailed, step-by-step
manner.
We're given an air temperature of 90 F and a mixing ratio (r) of 6
g/kg. We're supposed to find the relative humidity
(RH) and the dew point temperature. We start by
entering this data in the table.
Anytime you know the air's temperature you can look up
the saturation mixing ratio value on a chart (such as the one on
p. 86 in the ClassNotes); the saturation mixing ratio is 30 g/kg
for 90 F air. 90 F air could potentially hold 30 grams of
water vapor per kilogram of dry air (it actually contains 6 grams
per kilogram in this example).
Once you know mixing ratio and saturation mixing ratio you can
calculate the relative humidity (you divide the mixing ratio by
the saturation mixing ratio, 6/30, and multiply the result by
100%). You ought to be able to work out the ratio 6/30 in
your head (6/30 = 1/5 = 0.2). The RH is 20%.
The numbers we just figured out are shown on the top line above.
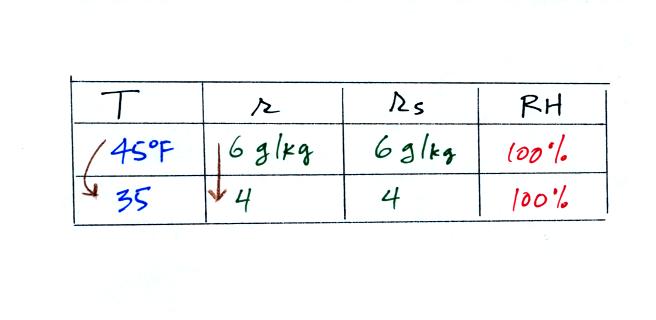
(A) We imagined cooling the air from 90F to 70F, then to 55F, and
finally to 45F.
(B) At each step we looked up the saturation mixing ratio and
entered it on the chart. Note that the saturation mixing
ratio values decrease
as the air is cooling.
(C) The mixing ratio
(r) doesn't change as we cool the air. The only
thing that changes r is adding or removing water vapor and we
aren't doing either. This is probably the most difficult
concept to grasp.
(D) Note how the relative humidity is increasing as we cool the
air. The air still contains the same amount of water vapor
it is just that the air's capacity is decreasing.
Finally at 45 F the RH becomes 100%. This is kind of a
special point. You have cooled the air until it has become
saturated. The dew point temperature in this
problem is 45 F.
What would happen if we cooled the air further still,
below the dew point temperature?
35 F air can't hold the 6 grams of water vapor that 45 F air
can. You can only "fit" 4 grams of water vapor into the 35 F
air. The remaining 2 grams would condense. If this
happened at ground level the ground would get wet with dew.
If it happens above the ground, the water vapor condenses onto
small particles in the air and forms fog or a cloud. Because
water vapor is being taken out of the air (the water vapor is
turning into water), the mixing ratio will decrease from 6 g/kg to
4 g/kg. As you cool air below the dew point, the RH stays
constant at 100% and the mixing ratio decreases.
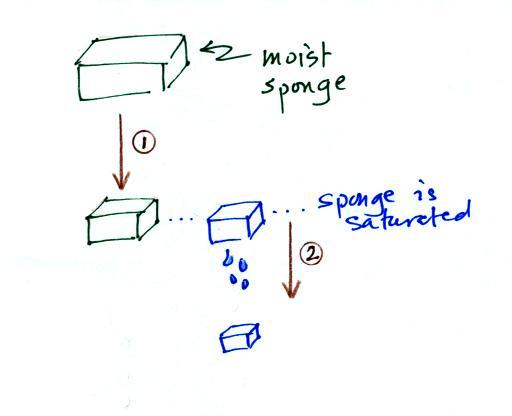
In many ways cooling moist air is liking squeezing a
moist sponge (this figure wasn't shown in class)
Squeezing the sponge and reducing its volume is like cooling moist
air and reducing the saturation mixing ratio. At first (Path
1 in the figure) when you squeeze the sponge nothing happens, no
water drips out. Eventually you get to a point where the
sponge is saturated. This is like reaching the dew
point. If you squeeze the sponge any further (Path 2) water
will begin to drip out of the sponge (water vapor will condense
from the air).
Here's something close to the chart we filled in during class.
You should be able to start with a blank sheet of paper, write
in the names of the 4 variables across the top; write down "job",
"units", "to increase value", and "to decrease value" along the
left edge; and then fill in the rest of the information shown