Monday, Mar. 11, 2019
Pokey Lafarge "Sadie Green"
(3:24), "Something
in the Water" (5:03), "St. Louis
Crawl" (3:08), "Garbage Man
Blues" (4:21)
We'll be using page
83, page 84 and page 85a from the
ClassNotes today.
In the next 2 weeks or so we'll learn a
little bit about how clouds form and will learn how to
identify and name the 10 main cloud types. Only 2 of
these are able to produce significant amounts of
precipitation. It's not as easy to produce
precipitation as you might think. That is something
else we'll be looking at and at the variety of types of
precipitation that result.
Clouds form (and precipitation may begin to fall) once the
relative humidity reaches 100%. We need to spend a
little time trying to better understand humidity variables
like relative humidity and what can cause them to
change. None of this humidity material will be on
this week's quiz.
Introduction to humidity variables
This
topic and the terms that we will be learning are probably
new and might be confusing. So here's an
introduction. We will be mainly be
interested in 4 variables:
Your task will be to learn the
"job" that each of these variables has, the variable's units,
and what can cause each of the variables to change value.
Mixing ratio ( r )
The bottom half of the figure below can
be found on page
83 in the ClassNotes.
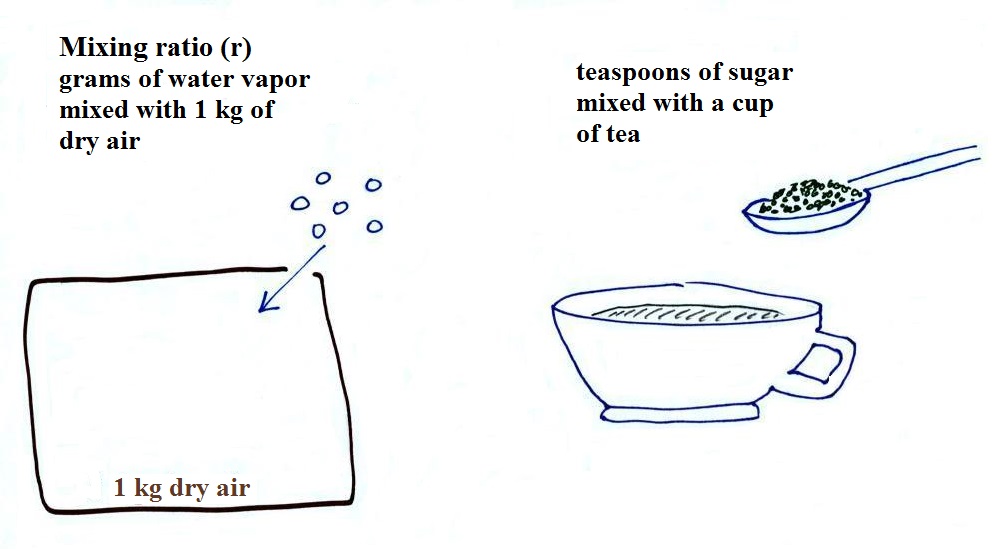
Mixing ratio tells you how much water vapor
is actually
in the air. Mixing ratio has units of grams of
water vapor per kilogram of dry air (the amount of water vapor
in grams mixed with a kilogram of dry air). A kilogram
of air is about one cubic meter of air (about one cubic yard
of air). Mixing ratio is basically the same idea as teaspoons
of sugar mixed in a cup of tea.
The value of the mixing ratio won't change
unless you add water vapor to or remove water vapor from the
air. Warming the air won't change the mixing
ratio. Cooling the air won't change the mixing ratio (with one exception
- when the air is cooled below its dew point temperature and
water vapor starts to condense). Since the mixing
ratio's job is to tell you how much water vapor is in the air,
you don't want it to change unless water vapor is actually
added to or removed from the air.
Saturation mixing ratio ( rS )
Saturation mixing ratio is just an upper limit
to how much water vapor can be found in air, the air's capacity
for water vapor. It's a property of air and depends on
the air's temperature; warm air can potentially hold
more water vapor than cold air. It doesn't
say anything about how much water vapor is actually in the air
(that's the mixing ratio's job). This
variable has the same units: grams of water vapor per kilogram
of dry air. Saturation mixing ratio values for different
air temperatures are listed and graphed on page
84 in the ClassNotes.

The sugar dissolved in tea analogy is still
helpful. Just as is the case with water vapor in air,
there's a limit to how much sugar can be dissolved in a cup of
hot water. And not only that, the amount depends on
temperature: you can dissolve more sugar in hot water than in cold
water.
The dependence of saturation mixing ratio on air
temperature is illustrated below:
The small specks represent all
of the gases in air except for the water vapor. Each of
the open circles represents 1 gram of water vapor
that the air could potentially hold. There are 15
open circles drawn in the 1 kg of 70 F air; each 1 kg of 70 F
air could hold up to 15 grams of water vapor. The 45 F air
only has 6 open circles; this cooler air can only
hold up to 6 grams of water vapor per kilogram of dry air.
The numbers 15 and 6 came from the table on p. 86.
Now we have gone and actually
put some water vapor into the volumes of 70 F and 45 F air (the
open circles are colored in). The same amount, 3 grams of
water vapor, has been added to each volume of air. Three
of the open circles have been colored in. The mixing
ratio, r, is 3 g/kg in both cases. One of the
figures is almost filled to capacity, with water vapor the other
is not. That's basically what the 3rd humidity variable,
relative humidity, tells us
Relative humidity (RH)
The relative humidity is the
variable most people are familiar with. It tells you how
"full" the air is with water vapor, how close it is to
being filled to capacity with water vapor, how
close the air is to being "saturated" with water
vapor. RH has units of %.
In the analogy (sketched on the right hand side of p. 83 in
the photocopied notes) 4 students wander into Classroom A which
has 16 empty seats. Classroom A is filled to
25% of its capacity. You can think of 4, the
actual number of students, as being analogous to the mixing
ratio. The classroom capacity is analogous to the
saturation mixing ratio. How full the room is is analogous
to the relative humidity.
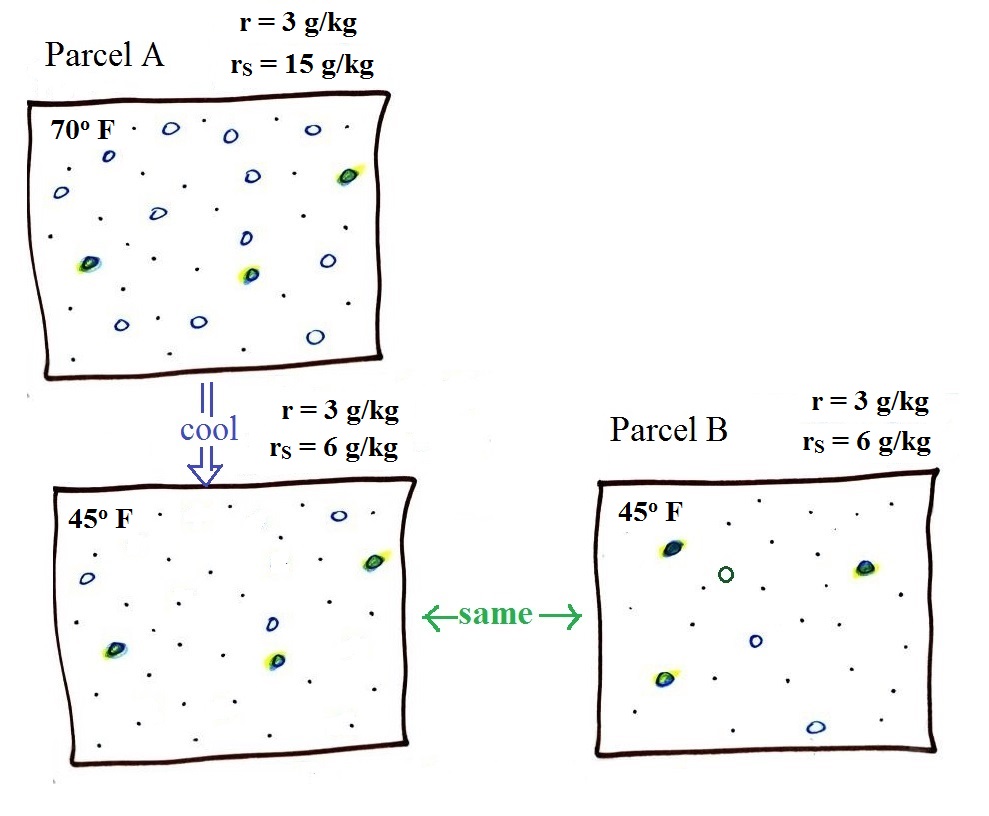
The figure below goes back to the volumes (1 kg each) of 70 F
and 40 F air that could potentially hold 15 grams or 5 grams of
water vapor.

Both the 70 F and the 45 F air each contain 3 grams of water
vapor. The 70 F air is only filled to 20% of capacity (3 of
the 15 open circles is colored in) because this warm air's
capacity, the saturation mixing ratio, is large. The RH in
the 45 F is 50% even though it has the same actual amount of water
vapor because the 45 F air can't hold as much water
vapor and is closer to being saturated.
Something important to note: RH doesn't
really tell you how much water vapor is actually in the air.
The two volumes of air above contain the same amount of water
vapor (3 grams per kilogram) but have very different values of
relative humidity. You could just as easily have two volumes
of air with the same relative humidity but different actual
amounts of water vapor.
What is the RH good for if it doesn't tell you how much
moisture is in the air? When the RH reaches 100% dew, fog,
and clouds form. RH tells you whether clouds or fog are
about to form or not.
Dew point temperature
The dew point temperature has
two jobs. First it gives you an idea of the actual amount
of water vapor in the air. In this respect it is
just like the mixing ratio. If the dew point temperature
is low the air doesn't contain much water vapor. If it is
high the air contains more water vapor. This is something
we learned early in the semester.
The dew point is a temperature and has units of
oF or oC
Second the dew point tells you how much you
must cool the air in order to raise the RH to 100% (at which
point a cloud, or dew or frost, or fog would form). This
idea of cooling the air until the RH increases to 100% is
important and is something we will use a lot.
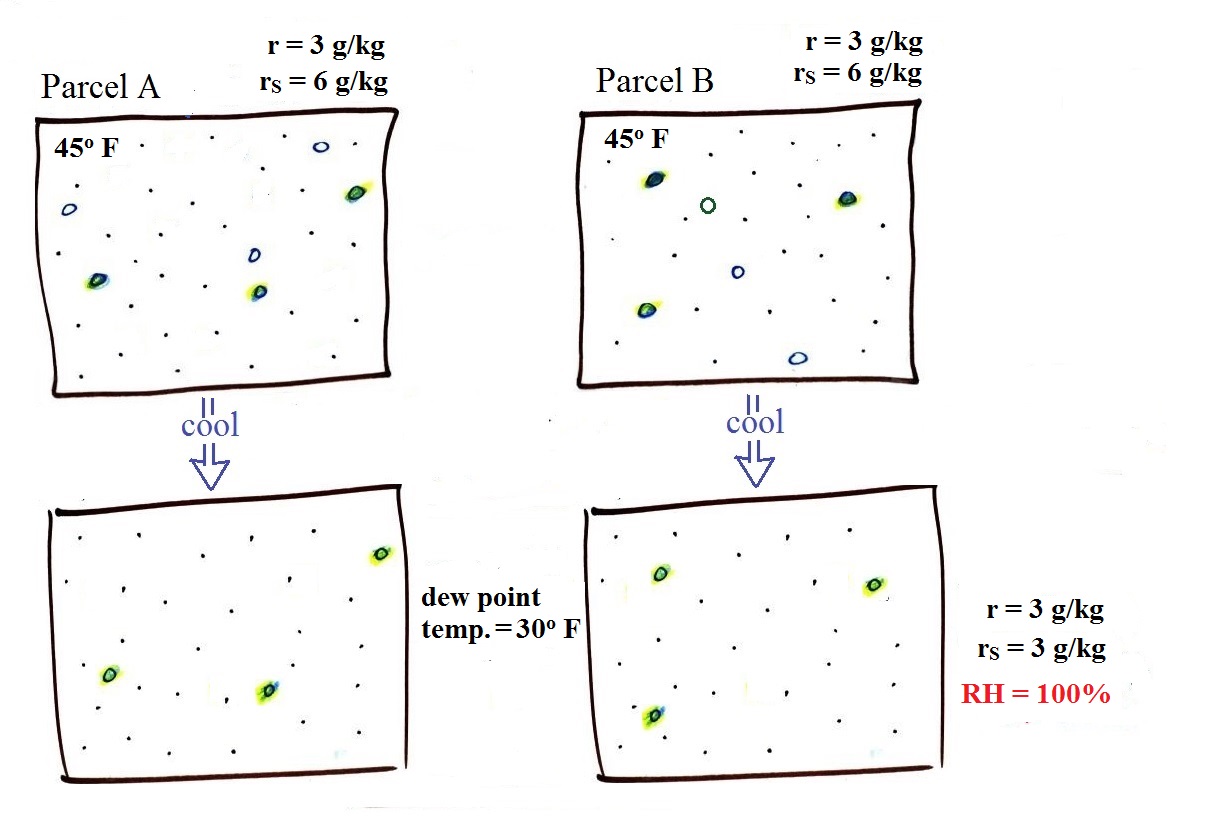
Let's first imagine cooling the 70 F volume
of air (Parcel A) down to 45 F. Once there we see that
Parcel A and Parcel B are identical. They both have the
same mixing ratios, the same saturation mixing ratios, and the
same relative humidities.
If we cool the both the parcels of 45 F air to 30 F we find that
the saturation mixing ratio would decrease to 3
grams/kilogram. Since the air actually contains 3 g/kg,
the RH of the 30 F air would become 100%. The 30 F air
would be saturated, it would be filled to capacity with water
vapor. 30 F is the dew point temperature for Parcel A, 70
F air that contained 3 grams of water vapor per kilogram of dry
air. It is also the dew point temperature for Parcel B,
the 45 F air that also contained 3 grams of water vapor per
kilogram of dry air. Because both
volumes of air had the same amount of water vapor, they both also have the same dew point
temperature.
Now back to the
student/classroom analogy.

The 4 students move into classrooms of smaller and smaller
capacity. The decreasing capacity of the
classrooms is analogous to the decrease in saturation mixing
ratio that occurs when you cool air. Eventually the
students move into a classroom that they just fill to capacity.
This is analogous to cooling the air to the dew point.
Humidity example problem #1
There are 4 humidity variables
(mixing ratio, saturation mixing ratio, relative
humidity, and dew point temperature).
Generally I'll give you values for two of them and
you'll need to figure out values for the other
two.
Here are the starting conditions for this first
problem
Tair = 90 F
|
r = 6 g/kg
|
RH = ?
|
Td = ?
|
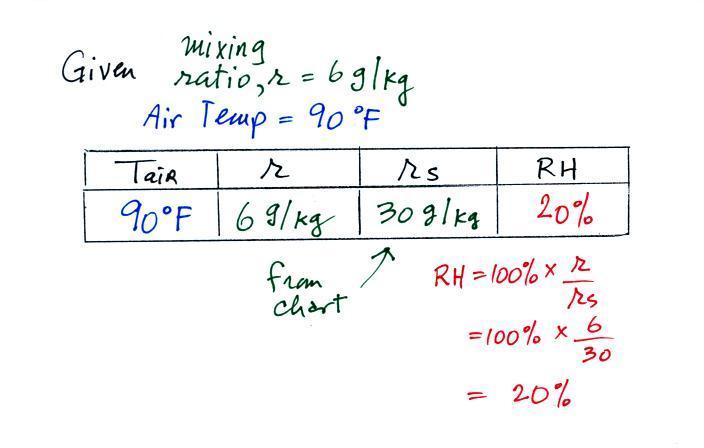
We start by entering the data we were given
Anytime you know the air's temperature
you can look up the saturation mixing ratio value on a chart
(such as the one on p. 86 in the ClassNotes); the saturation
mixing ratio is 30 g/kg for 90 F air. 90 F air could
potentially hold 30 grams of water vapor per kilogram of dry
air (it actually contains 6 grams per kilogram in this
example).
Once you know mixing ratio and saturation mixing ratio
you can calculate the relative humidity (you divide the
mixing ratio by the saturation mixing ratio, 6/30, and
multiply the result by 100%). You ought to be able to
work out the ratio 6/30 in your head (6/30 = 1/5 =
0.2). The RH is 20%.
The numbers we just figured out are shown on the top line
below.
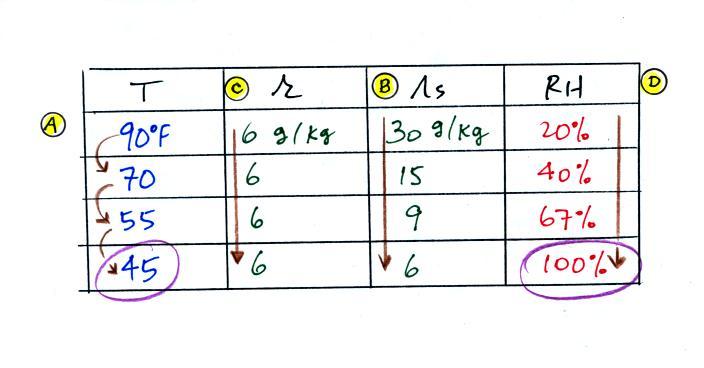
(A) To figure out the dew point, we imagine cooling the
air from 90F to 70F, then to 55F, and finally to 45F.
Note the effect this has on the mixing ratio, the saturation
mixing ratio and the relative humidity.
(B) At each step we looked up the saturation mixing
ratio and entered it on the chart. Note that the
saturation mixing ratio values decrease as the
air is cooling.
(C) The mixing
ratio (r) doesn't change as we cool the air.
The only thing that changes r is adding or removing water
vapor and we aren't doing either. This is probably the
most difficult concept to grasp.
(D) Note how the relative humidity is increasing as we
cool the air. The air still contains the same amount
of water vapor it is just that the air's capacity is
decreasing.
Finally at 45 F the RH becomes 100%. This is the
dew point. The dew point temperature is 45 F
What would happen if we cooled the air below the dew
point temperature?
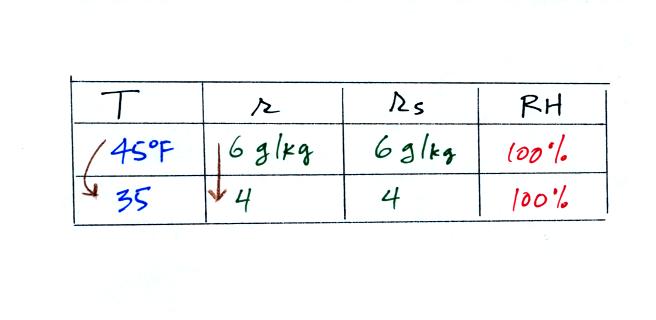
35 F air can't hold the 6 grams of water
vapor that 45 F air can. You can only "fit" 4 grams of
water vapor into the 35 F air. The remaining 2 grams
would condense. If this happened at ground level the
ground would get wet with dew. If it happens above the
ground, the water vapor condenses onto small particles in
the air and forms fog or a cloud. Because water vapor
is being taken out of the air (the water vapor is turning
into water), the mixing ratio will decrease from 6 g/kg to 4
g/kg. As you cool air below the dew point, the RH
stays constant at 100% and the mixing ratio decreases.
This is the situation where cooling the air seems to be
affecting the value of the mixing ratio. But it's
because we're cooling the air below the dew point and water
vapor is condensing. The air is actually losing water
vapor and the mixing ratio (whose job it is to tell you how
much water vapor is in the air) should decrease.
In many ways cooling moist air is liking
squeezing a moist sponge
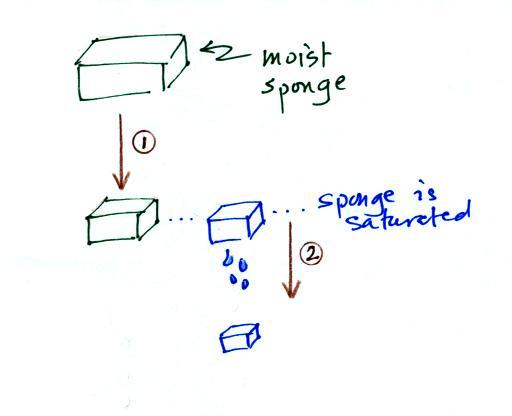
Squeezing the sponge and reducing its
volume is like cooling moist air and reducing the saturation
mixing ratio. At first (Path 1 in the figure) when you
squeeze the sponge nothing happens, no water drips
out. Eventually you get to a point where the sponge is
saturated. This is like reaching the dew point.
If you squeeze the sponge any further (Path 2) water will
begin to drip out of the sponge (water vapor will condense
from the air).