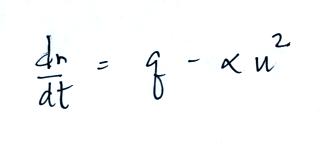
Today we will add two additional
small ion loss
terms. A small ion can attach to an uncharged particle, creating
a charged particle or a so-called "large ion".

Or a small ion of one polarity can attach to a charged particle of the opposite polarity creating an unchared particle (provided the small ion and the particle have equal quantities of charge). These two new terms are included in the small ion balance equation below. The β+o and β+- terms are referred to as "attachment coefficients."
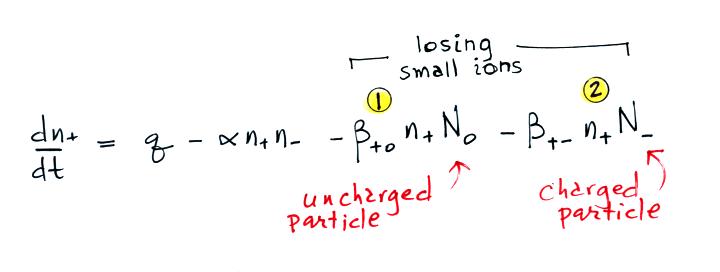

Or a small ion of one polarity can attach to a charged particle of the opposite polarity creating an unchared particle (provided the small ion and the particle have equal quantities of charge). These two new terms are included in the small ion balance equation below. The β+o and β+- terms are referred to as "attachment coefficients."

We often assume that the
concentrations of positive and negative small ions and the
concentrations of positively and negatively charged particles are
equal. Let's also make the following assumptions concerning the
attachment coefficients.
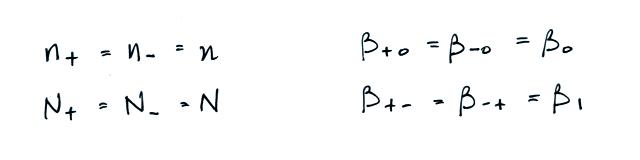
The balance equation then becomes

The bottom equation is just a simplification of the top
equation. A
total particle concentration term, Z, is used rather than keeping track
of the concentrations of charged and uncharged particles.
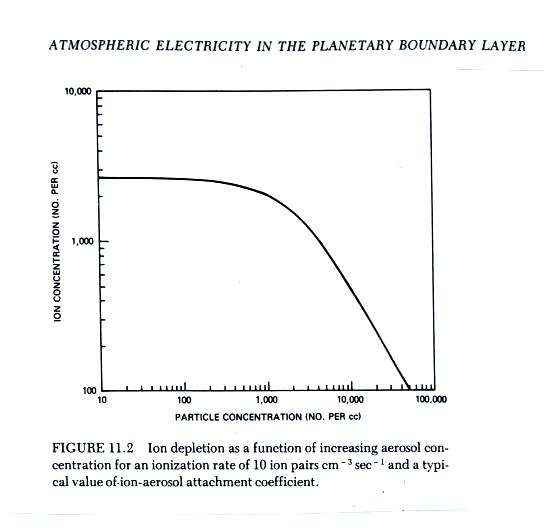
The figure below (from The Earth's Electrical Environment reference) illustrates how ion-particle attachment begins to significantly reduce small ion concentrations beginning at particles concentrations of about 1000 cm-3
In some past editions of this course we have spent close to a full class period looking at how you might derive expressions for the ion-particle attachment coefficients. I'm not sure that's really necessary here. Though I have added supplementary notes that you can look at if you are interested. You can find them here. The first part of these supplementary notes deals with the attachment to uncharged particles, the second part considers attachment to charged particles.
We will spend some time considering what fraction of particles are uncharged and charged. We'll start with a large ion (charged particle) balance equation.
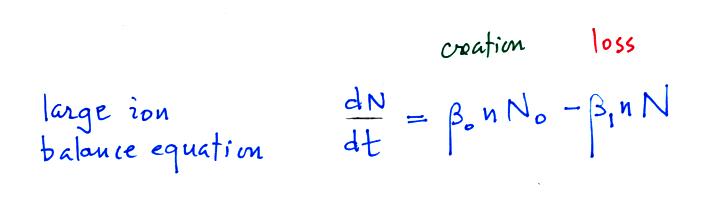
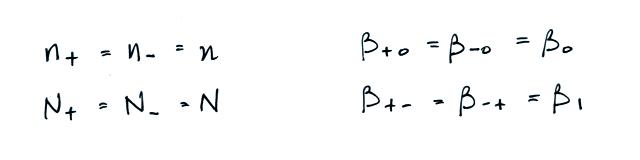

The figure below (from The Earth's Electrical Environment reference) illustrates how ion-particle attachment begins to significantly reduce small ion concentrations beginning at particles concentrations of about 1000 cm-3

In some past editions of this course we have spent close to a full class period looking at how you might derive expressions for the ion-particle attachment coefficients. I'm not sure that's really necessary here. Though I have added supplementary notes that you can look at if you are interested. You can find them here. The first part of these supplementary notes deals with the attachment to uncharged particles, the second part considers attachment to charged particles.
We will spend some time considering what fraction of particles are uncharged and charged. We'll start with a large ion (charged particle) balance equation.
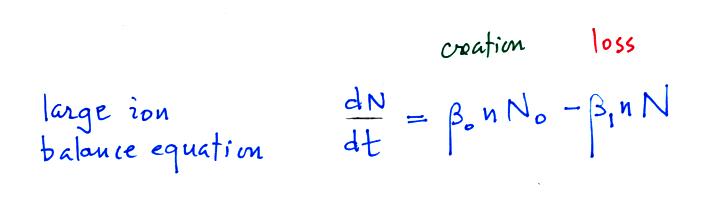
N in this equation can represent
the concentration of either positively or negatively charged
particles. Large ions are
created when a small ion attaches to an uncharged particle. They
are destroyed when a small ion attaches to a charged particle of the
opposite polarity .
Under steady state conditions
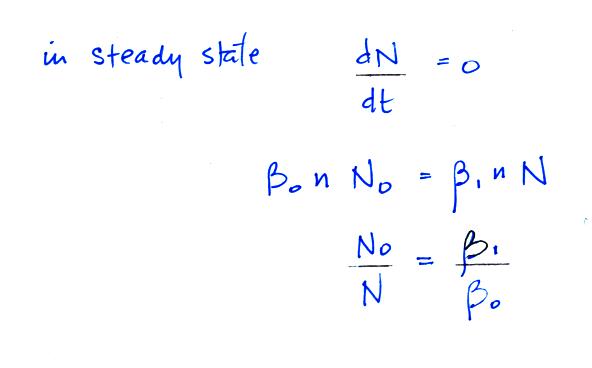
Under steady state conditions

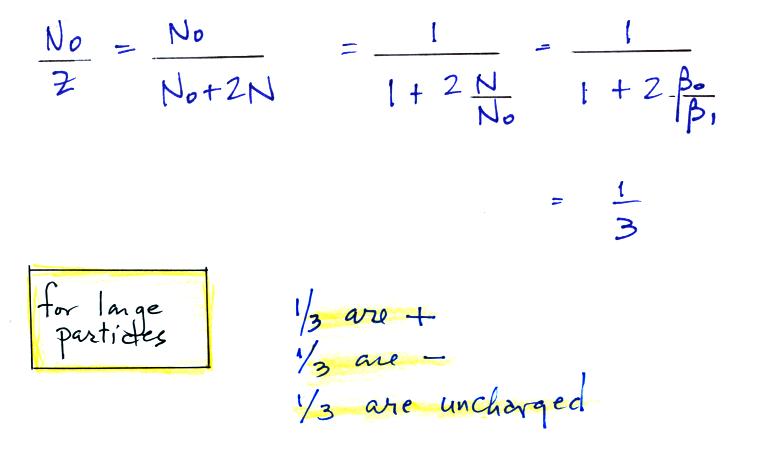
Now we'll look at the fraction of
large and small particles that are uncharged. In the
supplementary
notes we show that β0
= β1 for large
particles. In that case

For large particles you would
expect to find equal numbers of positively charged, negatively charged,
and non-charged particles.
For small particles
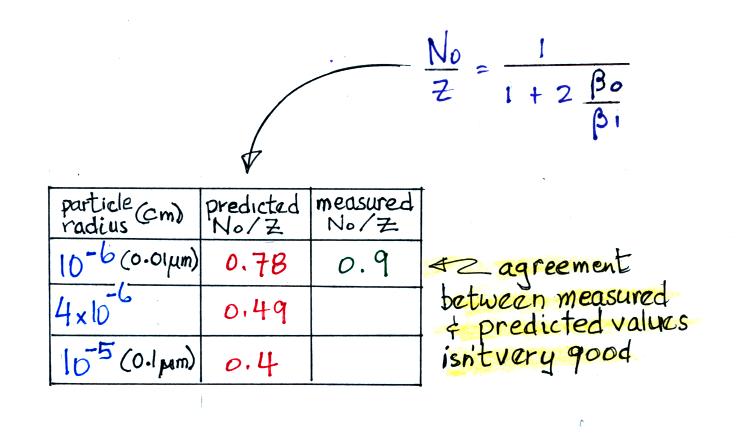
The agreement between predictions and measurements of the uncharged fraction (No/Z) is not very good for small particles. If the table had been extended to larger particles, the predicted No/Z would approach 0.33.
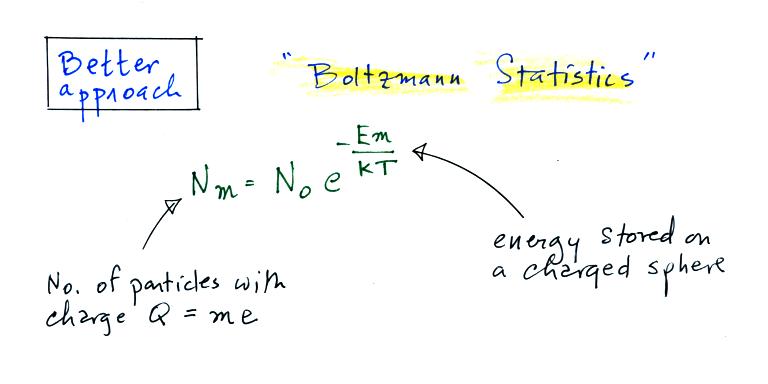
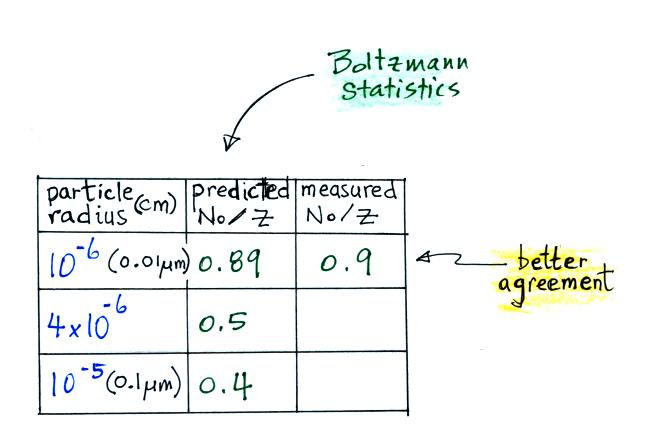
Better agreement is obtained using Boltzmann statistics.
For small particles

The agreement between predictions and measurements of the uncharged fraction (No/Z) is not very good for small particles. If the table had been extended to larger particles, the predicted No/Z would approach 0.33.
Better agreement is obtained using Boltzmann statistics.

A charged particle has a certain
amount of "stored" energy associated with it. Thus we can use the
Boltzmann distribution above to predict the distribution of charged
particles (the particles can carry only integral multiples of an
electronic charge, i.e. Q = me, where m is an integer and e is the
charge on an electron).
The energy stored on a charged sphere is (click here to see the details of the derivation)
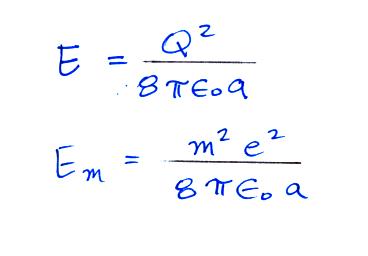
We can insert this expression into the Boltzmann distribution
equation above.

This agrees much better with the measured value. The table shown
earlier is reproduced below. This time Boltzmann statistics are
used to predict the uncharged fraction.
The energy stored on a charged sphere is (click here to see the details of the derivation)
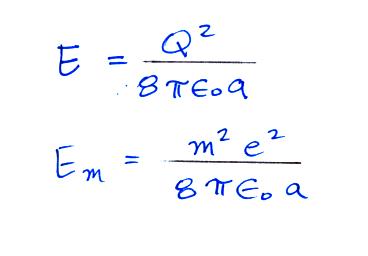

A temperature of 300 K was assumed
in the calculation above. The exponential starts to
become pretty small for particles with radii less that 2.8 x 10-6 cm
(especially if m > 1). So we can see that most small particles
will be uncharged. Those that are charged will mostly just carry
1 electronic charge. For example
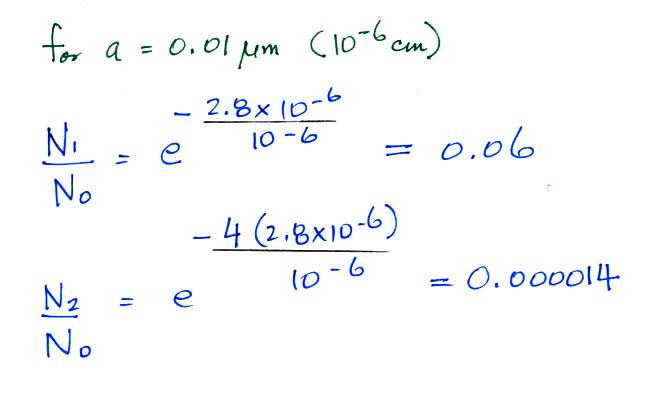
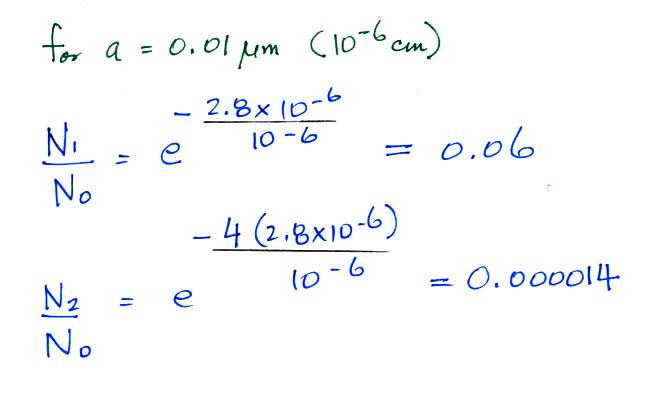
Now we can compare predictions of
the uncharged fraction of particles with measurements. Here are
the details of the predicted value for a particle with radius = 10-6 cm.
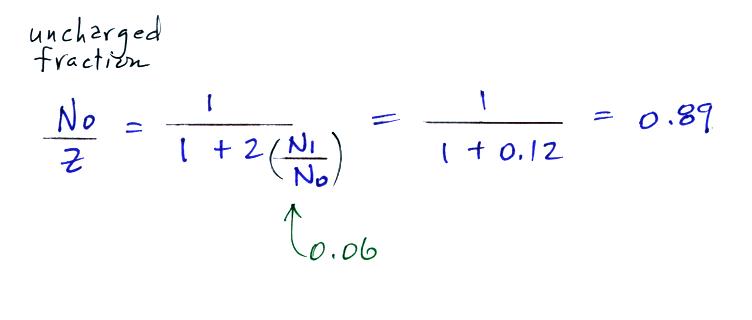
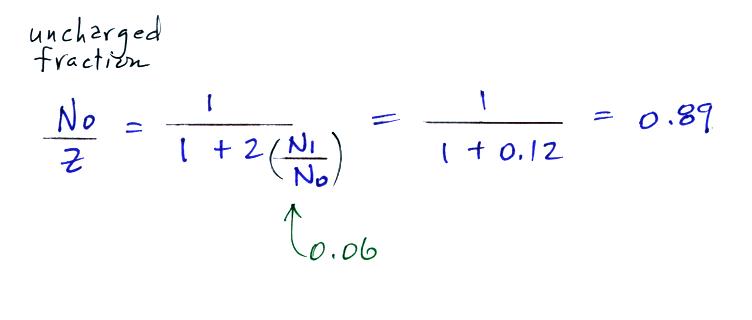

The agreement between measured and
predicted values is much better. Again, if the table
had been extended to larger particles, we would expect the predicted No/Z to approach 0.33.