Friday, Jan. 25, 2019
Dour/Le Pottier Quarter "Avel Gorn"
(4:48), Startijenn "Strak ha pak"
(4:23), "Skeud"
(7:46), "Rond
de Loudeac" (4:37); Plantec "Tennder"
(7:07)
We'll be using page 25,
page 33, page 35, page 36, page 34, and maybe page 26 from the ClassNotes
today.
Quick recap
atmospheric
pressure at any level in the atmosphere
depends on (is determined by)
the weight
of the air overhead
Pressure units (found on page
24a in the ClassNotes packet)
Inches of mercury refers to the
reading on a mercury barometer. This
seems like unusual units for pressure. But mercury
(13.6 grams/cm3)
is denser than steel (about 7.9 grams/cm3). If we could
some how construct a solid 1" x 1" bar of mercury it would
only need to be 30 inches long to equal the weight or the
iron bar or the weight of a tall column of air.
Each of these columns would weigh 14.7 pounds. The
pressure at the base of each would be the same.
A mercury barometer is really just a balance. You
balance the weight of a very tall column of air with the weight
of a much shorter column of (liquid) mercury. You'll find
a more detailed explanation of how mercury barometers work in a
separate Supplementary
Reading section.
You never know where something you learn in ATMO 170 will turn
up. Take pressure units for example.
I once lived and worked in France. Here's a picture of a
car I owned when I was there (the one below is in mint
condition, the one I had was in far worse shape).

It's
a Peugeot
404. I was at
the service station one day and decided to pump up the
tires a little bit. I wanted to put about 30 psi
into the tires but the scale on the compressor only went
up to 4. Not knowing much French it took me 15
minutes, before I realized
the air compressor was marked in "bars" not "psi". Since
14.7 psi is about 1 bar, 30 psi would be about 2 bars (90 psi
needed in my bicycle tires would be about 6 bars).
Changes in atmospheric pressure with altitude
If you remember and understand the statement
atmospheric
pressure at any level in the atmosphere
depends on (is determined by)
the weight
of the air overhead
You can quickly and easily figure out what happens to air
pressure as you move upward in the atmosphere. A
pile of bricks is helpful too. Here's
a picture of 5 bricks stacked on top of each
other.
Each brick weighs 5 pounds, there's a total of 25
pounds of weight. At
the bottom of the pile you would measure a weight of 25
pounds. If you moved up a brick you would measure a
weight of 20 pounds, the weight of the four bricks that
are still above. The pressure would be less.
Weight and pressure will decrease as you move up the pile.
Layers
of air in the atmosphere is not too much
different from a pile of bricks.
Pressure at any level is determined by the
weight of the air still overhead.
Pressure decreases with increasing
altitude because there is less and less
air remaining overhead. The figure
below is an older version of the figure on
page
25 in the ClassNotes.
At sea level altitude the pressure is normally about 1000
mb. That is determined by the weight of all (100%)
of the air in the atmosphere.
Some parts of Tucson are 3000 feet
above sea level (most of central Tucson is a little lower
than that, around 2500 feet). At 3000 ft. about 10%
of the air is below, 90% is still overhead. It is
the weight of the 90% that is still above that determines
the atmospheric pressure in Tucson. If 100% of the
atmosphere produces a pressure of 1000 mb, then 90% will
produce a pressure of 900 mb.
Pressure is typically about 700 mb at
the summit of Mt. Lemmon (9000 ft. altitude) because 70% of the
atmosphere is overhead..
Pressure decreases rapidly with
increasing altitude. We will find that pressure
changes more slowly if you move horizontally.
Pressure changes about 1 mb for every 10 meters of
elevation change. Pressure changes much more slowly
normally if you move horizontally: about 1 mb in 100
km. Still the small horizontal changes are what
cause the wind to blow and what cause storms to form.
Here's an example of
a surface weather map from last Friday. The yellow
lines are pressure contours and are called
isobars. Note that the values are near the 1000 mb
average sea level pressure value that we would
expect at sea level altitude.
The sketch of pressure versus altitude shows a
submarine at a depth of about 30 ft. or so. The
pressure there is determined by the weight of the air plus
the weight of the water overhead. Water is much
denser and much heavier than air. At 30 ft., the
pressure is already twice what it would be at the surface
of the ocean (2000 mb instead of 1000 mb).
I learned about a relatively new sport called free diving
a semester or two ago. Basically divers see how deep they
can go while holding their breath. They must descend and
return to the surface on just a single lungful of air. It is
a very hazardous sport. Here is a link
to an article about a diver that made it to a depth of 236
feet but died upon reaching the
surface. Death was caused by the
high pressure deep under water forcing fluid into the
diver's lungs.
The downward force of air pressure
I am concerned that you might be thinking that a
sea level pressure value of 14.7 psi might not sound like
much. But when you start to multiply
14.7 by all the square inches on your body it turns into a lot
of pounds of force.
The yellow box on the person's chest in the picture is a
brick size, 4" x 8" = 32 square inch, area. If you
multiply 14.7 psi by 32 sq. in. you get 470 pounds! It
would take a stack of 90 to 100 bricks to produce that much
weight. Imagine lying on the beach with 90 bricks
stacked up on your chest. That's what the atmosphere is
doing.
Why isn't the person in the picture above
crushed by the weight of the atmosphere above. The
answer is that the person's body pushes back with the same
amount of force. Air does the same thing.
Air pressure pushes downward, upward and sideways
Air pressure is a force that pushes downward, upward, and
sideways. If you fill a balloon with air and then push
downward on it, you can feel the air in the balloon pushing back
(pushing upward). You'd see the air in the balloon pushing
sideways as well.
We were able to see this by placing a brick
on top of a balloon. The balloon gets squished (pushed out
sideways) but not flattened. It eventually pushes upward
with enough force to support the brick. The squished
balloon is what air at the bottom of the atmosphere looks
like. And it is supporting more than just one brick, it is
supporting a pile 90 to 100 bricks tall.
Another helpful representation
of air in the atmosphere might be a people pyramid.

The people in the figure are like layers of
air in the atmosphere all stacked on top of each other.
If the bottom person in the stack above were standing on a
scale, the scale would measure the total weight of all the
people in the pile. That's analogous to sea level
pressure being determined by the weight of the all the air
above.
The bottom person in the picture above must be
strong enough to support the weight of all the people
above. The bottom layer of the atmosphere pushes upward
with enough pressure to support the weight of the air above.
Here's another pretty amazing example of air pressure
pushing upward.
This is my present day car (a 1980 Toyota
Celica). It sits on 4 tires, which are really nothing more
than balloons. The air pressure in the four tires
pushes upward with enough force to keep the 1000 or 2000 pound
vehicle off the ground. The air pressure also pushes
downward, that's something you'd feel if the car ran over your
foot. The air also pushes sideways with a lot of force;
tires need to be strong to keep from exploding or coming off the
rim.
6. Upward Air Pressure force demonstration
This is a logical point to do a demonstration. A demo
that tries to prove that air pressure really does push upward as
well as downward. Not only that but that the upward force
is fairly strong. The demonstration is summarized on page
35 in the ClassNotes.
It's pretty obvious that if you fill a balloon with a
little water and let go it will drop. And most everyone
in the class knows why.
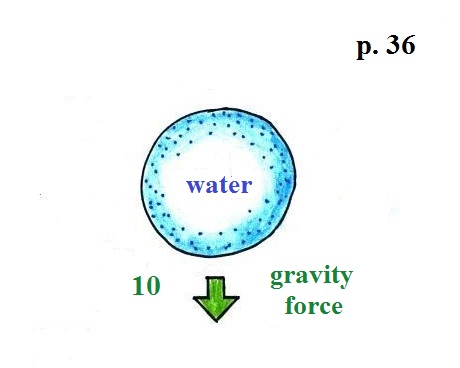
Gravity
exerts a downward force on the balloon. I just
made up a number, 10, to give you some idea of its
strength.
But the picture above isn't quite complete.
The water balloon is surrounded by air that is
pushing upward, downward, and sideways on the balloon.
These pressure forces
are strong but mostly cancel each other out. The
sideways forces do cancel out exactly.
The up and down forces aren't quite equal because pressure
decreases with increasing altitude. The upward
pointing force at the bottom is stronger (15 units) than the
downward force at the top (14 units). They don't
cancel and there is a weak upward
pressure difference force (1 unit
strong). I'm pretty sure that most people don't know
about this pressure difference force.
This picture includes all
the forces (gravity and pressure difference).
The downward gravity force is stronger than the
upward pressure difference force and the balloon
falls.
It seems like we could change things a
little bit and somehow keep the upward and downward
pressure forces from working against each other. That's
what we do in the demonstration.
In
the demonstration a wine glass is filled
with water (about the same amount of water
that you might put in a small water
balloon).
A small plastic lid is used to cover the wine glass (you'll
need to look hard to see the lid in the photo above). The
wine glass is then turned upside and the water does not fall
out.

All the same forces are shown again in the left most
figure. We'll split that into two parts - a water and lid
part and an empty glass part.
The 14 units of pressure force is pushing on the glass now
and not the water. I was holding onto the glass, I'm the
one that balanced out this downward pressure force.
Gravity still pulls downward on the water with the same 10
units of force. But with 15 units, the upward pressure
force is able to overcome the downward pull of gravity. It
can do this because all 15 units are used to overcome gravity
and not to cancel out the downward pointing pressure
force.
The Magdeburg hemispheres
experiment (sideways pressure force)
Air pressure pushes downward with hundreds of pounds of force
on someone lying on the beach.
The pressure of the air in tires pushes
upward with enough force to keep a 1 ton automobile off the
ground.
What about the sideways air pressure
force?
Here's a description of a
demonstration that really needs to be done in Arizona Stadium
at half time during a football game. It involves
Magdeburg hemispheres and two teams of horses (the following
quote and the figure below are from an
article in Wikipedia):
" ... Magdeburg hemispheres are a
pair of large copper hemispheres with mating rims, used to
demonstrate the power of atmospheric pressure. When the rims
were sealed with grease and the air was pumped out, the sphere
contained a vacuum and could not be pulled apart by
teams of horses. The Magdeburg hemispheres were designed by a
German scientist and mayor of Magdeburg, Otto von Guericke in
1656 to demonstrate the air pump which he had invented, and
the concept of atmospheric pressure."

Gaspar Schott's sketch of Otto von
Guericke's Magdeburg hemispheres experiment (from the
Wikipedia article referenced above)
It is the pressure of the air pushing
inward against the outside surfaces of the hemispheres
that keeps them together. The hemispheres appear to
have had pretty large surface area. There would be
15 pounds of force pressing against every square inch (at
sea level) of the hemisphere which could easily have been
several thousand pounds of total force.
Suction cups work the same way
The suction cup has been pressed against smooth surface.
The cup is flexible and can be pulled away from the wall leaving
a small volume between the wall and the cup where there isn't
any air (a vacuum). There's no air pressure pushing
outward, away from the wall, in the space between the wall and
the suction cup. There's just pressure from the air
surrounding the suction cup that is pushing and holding it
against the wall. The name suction cup is a little
misleading. There isn't any suction, rather just air
outside the suction cup pushing and holding it against the wall.
I suspect that if I were to attach the suction cup I had in
class to a white board mounted to a wall and were to ask a
couple of strong people to come down and try to pull it off the
white board they would end up pulling the white board off the
wall. The Facilities Management people wouldn't appreciate
that very much.
Changes in air density with
altitude
(see page
34 in the ClassNotes)
We've spent a lot of time (too much?)
looking at air pressure and how it changes with
altitude. Next we'll consider air density.
How does air density change with increasing
altitude? You know the answer to that question.
You get out of breath more easily at high altitude than at
sea level. Air gets thinner (less dense) at higher
altitude. A lungful of air at high altitude just
doesn't contain as many oxygen molecules as it does at lower
altitude or at sea level.
It would be nice to also understand why air density
decreases with increasing altitude.
The people pyramid reminds you that there is more
weight, more pressure, at the bottom of the atmosphere than
there is higher up.
Layers of air are not solid and rigid like in a stack of
bricks. Layers of air are more like mattresses stacked on
top of each other. Mattresses are
compressible, bricks (and people) aren't. Mattresses are
also reasonably heavy, the mattress at the bottom of the pile
would be squished by the weight of the three mattresses
above. This is shown at right. The mattresses higher
up aren't compressed as much because there is less weight
remaining above. The same is true with layers of air in
the atmosphere.
The statement above is at the top of page 34 in the
ClassNotes.
There's a surprising amount of information in this figure,
we(you) need to spend a minute or two looking for it
1. You can first notice and remember that pressure decreases
with increasing altitude. 1000 mb at the
bottom decreases to 700 mb at the top of the picture. You
should be able to explain why this happens.
2. Each layer of air contains the same amount (mass) of
air. This is a fairly subtle point.
You can tell because the pressure drops by the same amount, 100
mb, as you move upward through each layer. Pressure
depends on weight. So if all the pressure changes are
equal, the weights of each of the layers must be the same.
Each of the layers must contain the same amount (mass) of air
(each layer contains 10% of the air in the atmosphere).
3. The densest air is found at the bottom of the picture.
The bottom layer is compressed the most because it is supporting
the weight of all of the rest of the atmosphere. It is the
thinnest layer in the picture and the layer with the smallest
volume. Since each layer has the same amount of air (same
mass) and the bottom layer has the smallest volume it must have
the highest density. The top layer has the same amount of
air but about twice the volume. It therefore has a lower
density (half the density of the air at sea level).
Density is decreasing with increasing altitude.
That's the main point in this figure.
4. A final point. Pressure decreases 100
mb in a fairly short vertical distance in the bottom layer of
the picture - a rapid rate of decrease with altitude. The
same 100 mb drop takes place in about twice the vertical
distance in the top layer in the picture - a smaller rate of
decrease with altitude. Pressure is decreasing
most rapidly with increasing altitude in the densest air in the
bottom layer. We'll make use of this
concept again at the end of the semester when we try to figure
out why/how hurricanes intensify and get as strong as they do.


















