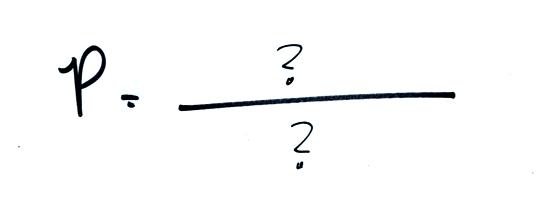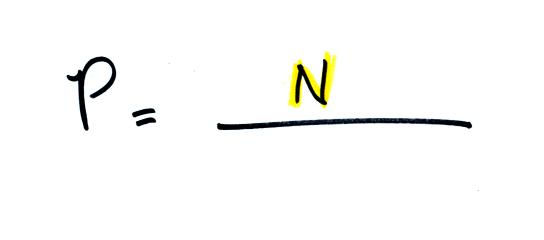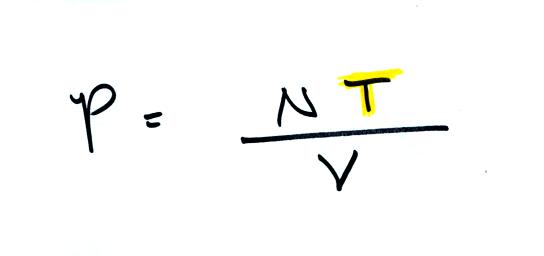Wednesday Feb. 27, 2008
The Experiment #2 reports are due next Monday. Bring your
materials in now and pick up the supplementary information sheet.
The revised Expt. #1 reports are also due next Monday.
1S1P Assignment #2 is now available.
Copies of the worksheets
that accompany this assignment will be distributed in class on Friday.
The worksheets are an optional part of the assignment, they aren't
required.
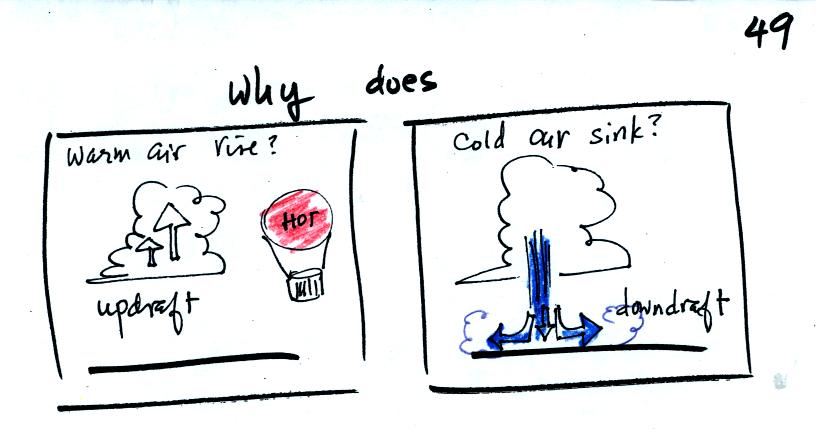
We are going to try to understand why
warm air rises and cold air sinks (the figure above is on p. 49 in the
photocopied Classnotes)..
It is always a good idea to have a picture in mind, a hot air balloon
for example. Cold air pours out of thundestorms in the
summer. When this descending air hits the ground it spreads our
horizontally under the storm. These surface winds can sometimes
reach 100 MPH which makes them stronger than most tornadoes.
Hot air balloons do sometimes fall from the sky; most everyone would
understand that gravity was the force responsible for
bringing down a hot air balloon.
But what causes a hot air balloon to rise? We will see that it is
a pressure difference force. Pressure decreases with increasing
altitude. This creates a force that points upward from high
toward low pressure.
Understanding rising and sinking air is a 3-step
process. The
first step is learning about the ideal gas law.
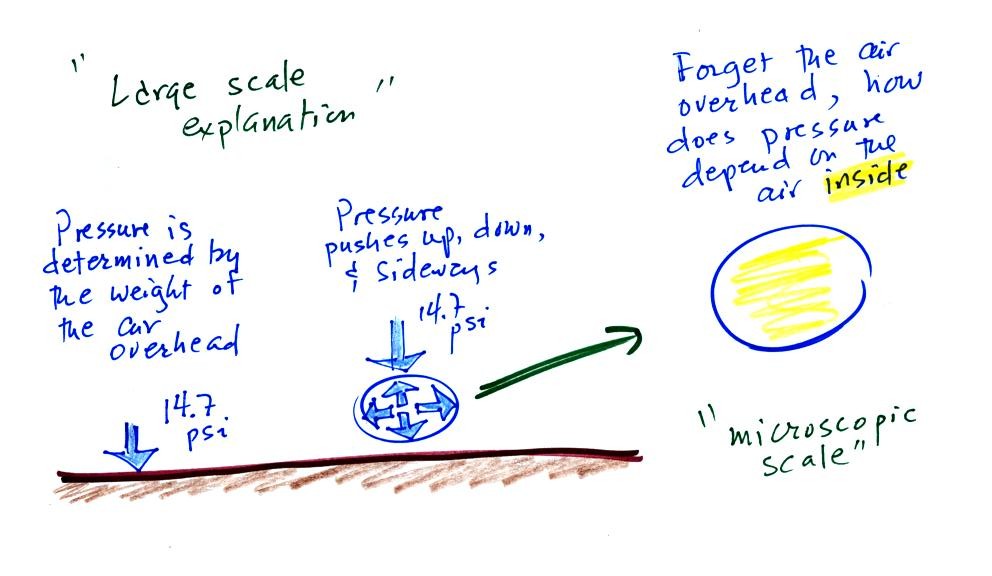
Up to this point in the semester we have been thinking of pressure as
being determined
by the weight of the air overhead. Air pressure pushes down
against the ground at sea level with 14.7 pounds of force per square
inch. If you imagine the weight of the atmosphere pushing down on
a balloon sitting on the ground you realize that the air in the balloon
pushes back with the same force. Air everywhere in the atmosphere
pushes upwards, downwards, and sideways. The ideal gas law
equation will give us an idea of what
determines the strength of the pressure inside the balloon.
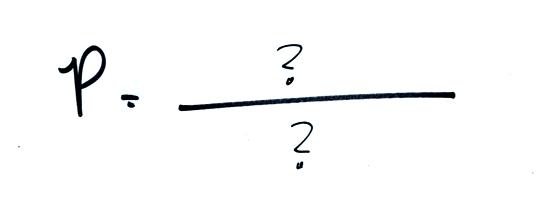
Step
#1 - ideal gas law
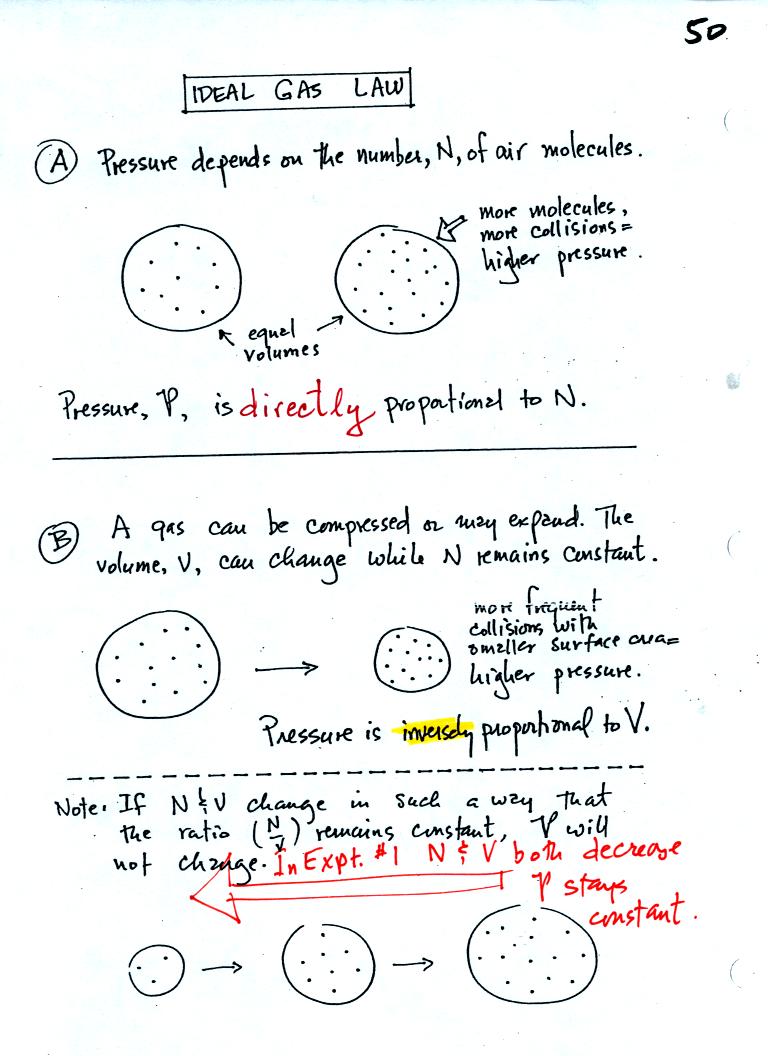
The pressure produced by the air
molecules inside a balloon will
first depend on how many air molecules are there. If there
weren't any air molecules at all there wouldn't be any
pressure. As you add more and more add to something like a
bicycle tire, the
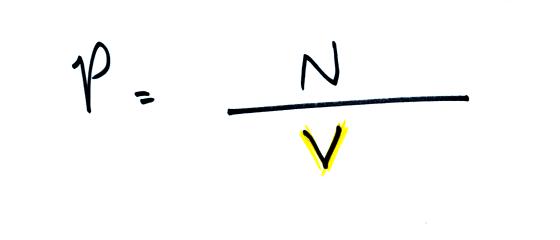
pressure increases. Pressure is directly proportional to N - an
increase in N causes an increase in P. If N doubles, P also
doubles (as long as the other variables in the equation don't change).
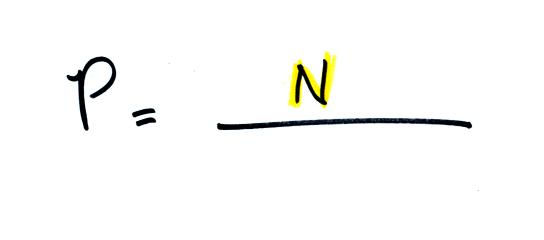
Air pressure inside a balloon
also
depends on the size of the
balloon. Pressure is inversely proportional to volume, V
. If V were to double, P would drop to 1/2 its original value.
Note it
is possible to keep pressure constant by changing N and V
together in just the right kind of way. This is what happens in
Experiment #1 that some of you are working on. Oxygen in a
graduated cylinder reacts with steel wool to form rust. Oxygen is
removed from the air sample which is a decrease in N. As oxygen
is removed, water rises up into the cylinder decreasing the air sample
volume. N and V both decrease in the same relative amounts and
the air sample pressure remains constant.
If you were to remove 20% of the air molecules, V would decrease
to 20% of its original value and pressure would stay constant.
Increasing
the temperature of the gas in a balloon will cause the gas molecules to
move more quickly. They'll collide with the walls of the balloon
more frequently and rebound with greater force. Both will
increase the pressure.
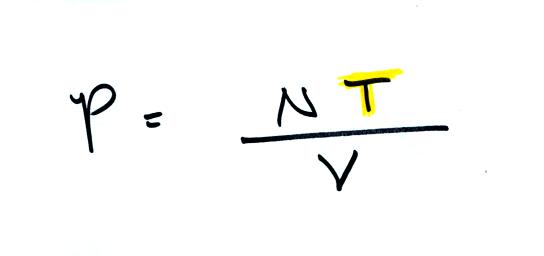
Surprisingly the pressure
does
not depend on the mass of the
molecules. Pressure doesn't depend on the composition of the
gas. Gas molecules with a lot of mass will move slowly, the less
massive molecules will move more quickly. They both will collide
with the walls of the container with the same force.
An attempt was made to demonstrate the effect of temperature on
pressure. An air-filled flask was connected to a manometer.
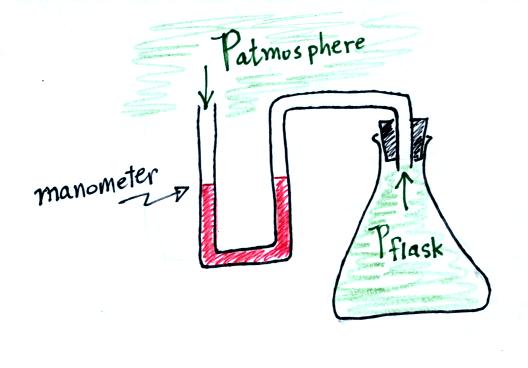
Initially the air in the flask was exactly the same as the air
outside. The levels of the red liquid in the manometer were the
same indicating the Patmosphere and Pflask were
the same. Next we both warmed and cooled the air in the
flask.
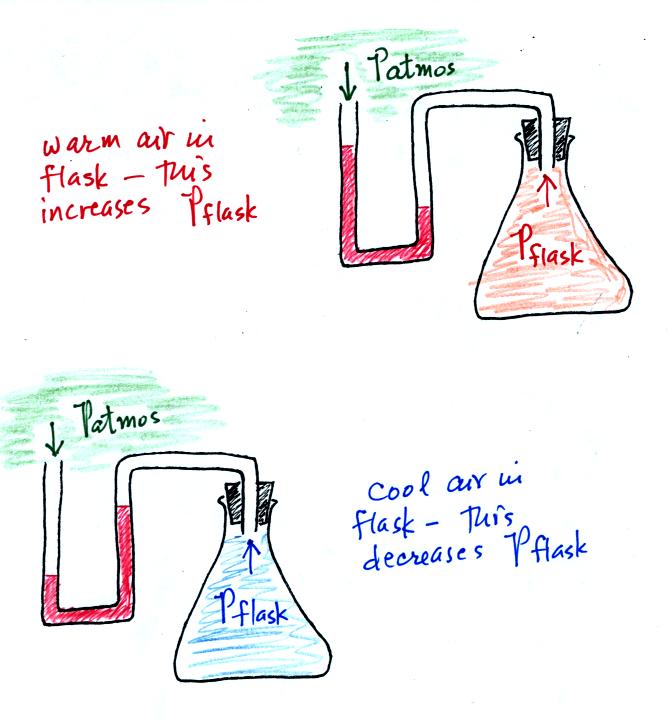
Warming the air in the flask increased the pressure inside the
flask. Cooling the flask reduced the pressure of the air in the
flask. The changing liquid levels revealed these changes in
pressure.
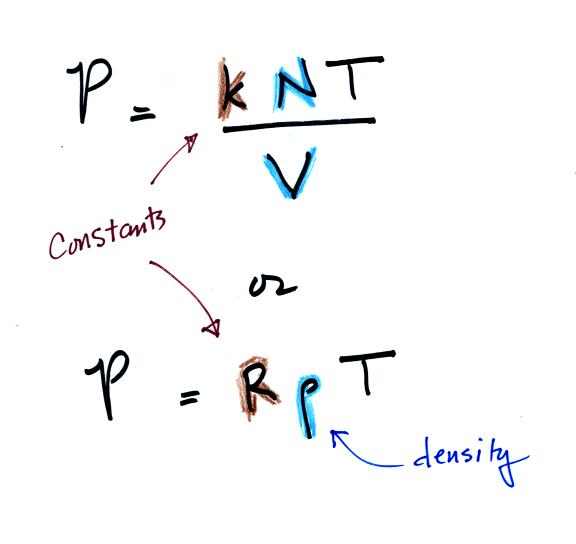
Two ideal gas law equations are
shown: the one we just derived and a slightly different form of the
same equation. You can
ignore the
constants k and R if you are just trying to understand how a change in
one of the variables would affect the pressure. You only need the
constants when you are doing a calculation involving numbers.
Here is an ideal
gas law animation that wasn't shown in class.
You
can vary N, V, or T and see the effect on pressure. Caution the
animation sometimes takes quite a while to load.
Step
#2 - Charles' law
We're still trying to understand why warm air rises and cold air
sinks. We've still got two more steps to go.
We now
turn our attention to Charles' Law, a special situation involving the
ideal gas law. This is Step #2.
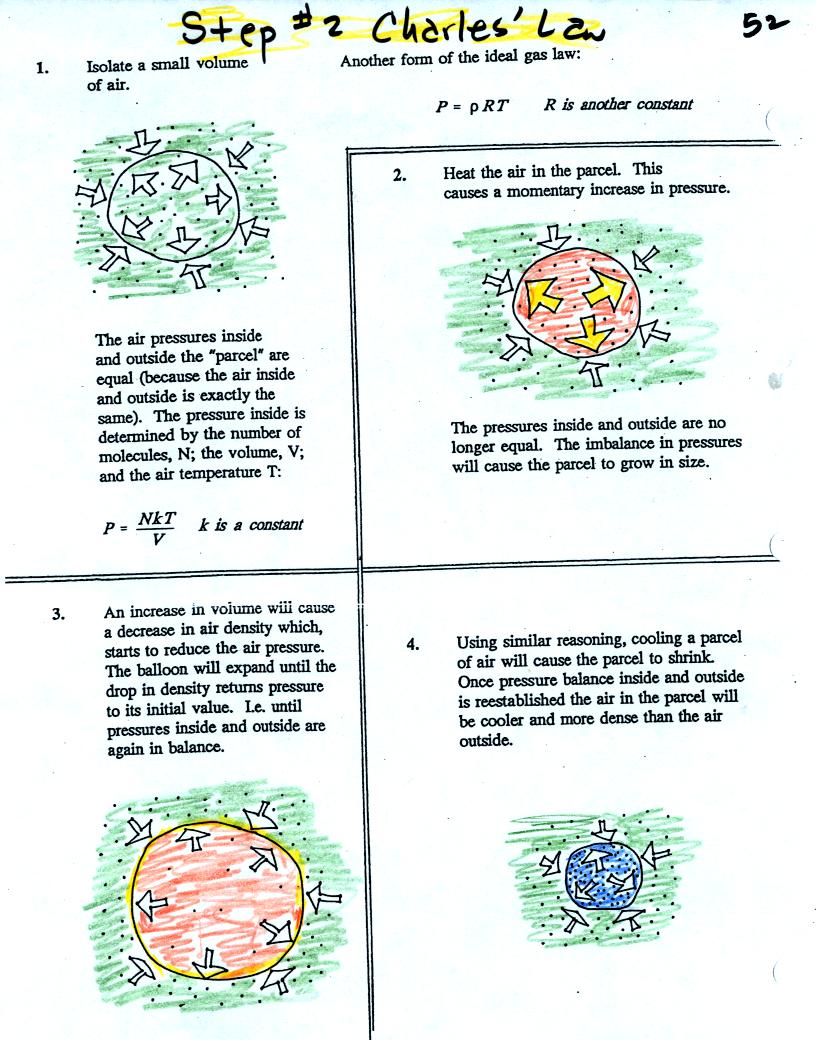
Read through the explanation on p. 52 in the photocopied
Classnotes. If you feel comfortable with that explanation then
you can skip to the Charles Law demonstration further along in these
online notes. Otherwise you can read the following explanation.
Air in the atmosphere behaves like air in a
balloon. A
balloon can grow or shrink in size depending on the pressure of the air
inside.
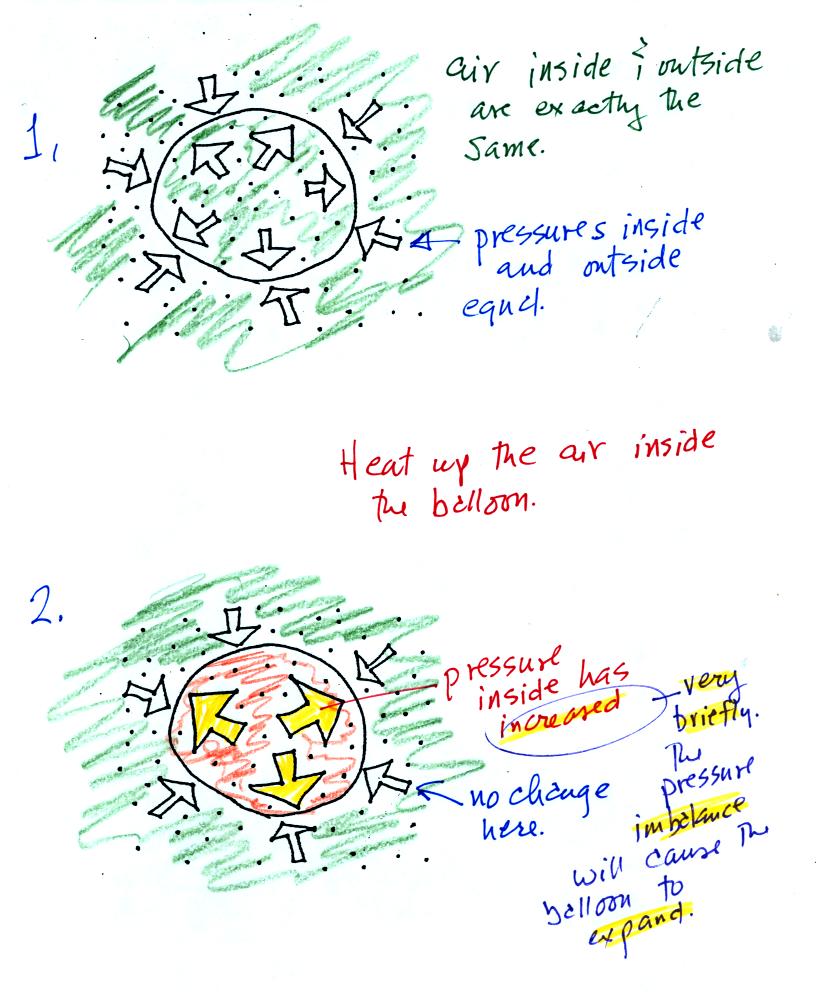
We start in the top figure with air inside a balloon that
is exactly the same as the air outside. The air inside and
outside have been colored green. The arrows show that the
pressure of the air inside pushing outward and the pressure of
the air surrounding the balloon pushing inward are all the same.
Next we warm the air in the balloon (Fig. 2). The ideal gas law
equation
tells us that the pressure of the air
in the balloon will increase. The increase is
momentary though.
Because the pressure inside is now greater than
the pressure outside, the balloon will expand. As volume begins
to increase, the pressure of the air inside the balloon will
decrease.
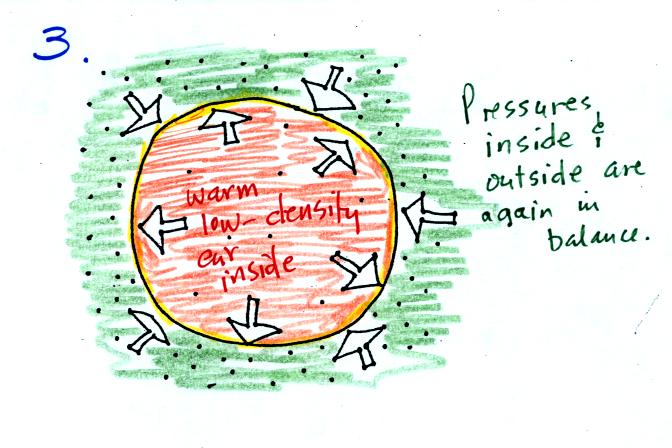
Eventually the balloon will expand just enough that the pressures
inside and
outside are again in balance. You end up with a balloon of warm
low density air that has the same pressure as the air surrounding it
(Fig. 3)
You can use the same reasoning to understand what happens when you cool
the air in a balloon.
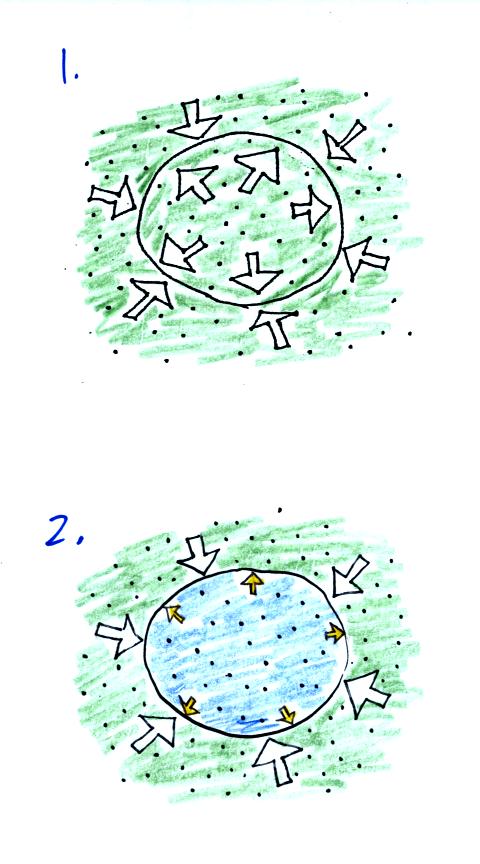
The air inside and outside are the same in Fig. 1. Cooling the
air inside the balloon in Fig. 2 causes a momentary drop in the inside
pressure and creates a pressure imbalance. The larger outside air
pressure compresses the balloon.
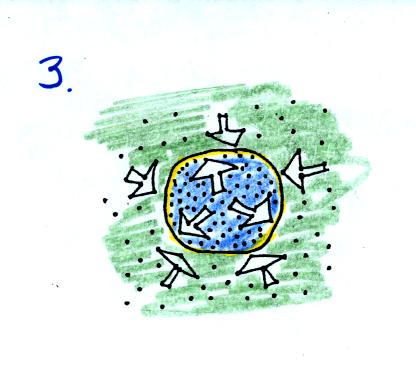
As the balloon volume decreases, pressure inside the balloon
increases. It eventually is able to balance the outside air
pressure. You end up with a balloon filled with cold high
density air.
These two associations:
warm air = low density air and
cold air = high density air
are important and will come up a lot during the remainder of the
semester.
Demonstration
of Charles' Law
Charles Law can be demonstrated by dipping a balloon in
liquid
nitrogen.

The balloon had shrunk down to practically no volume when
pulled from the liquid nitrogen. It was filled with cold high
density air. As
the balloon warmed the balloon expanded and the density of the air
inside
the balloon decreased. The volume and temperature kept changing
in a way that kept pressure constant.
Step
#3 - vertical forces acting on air parcels
Now the final step, we must learn about the vertical
forces that
operate on a parcel of air in the atmosphere.
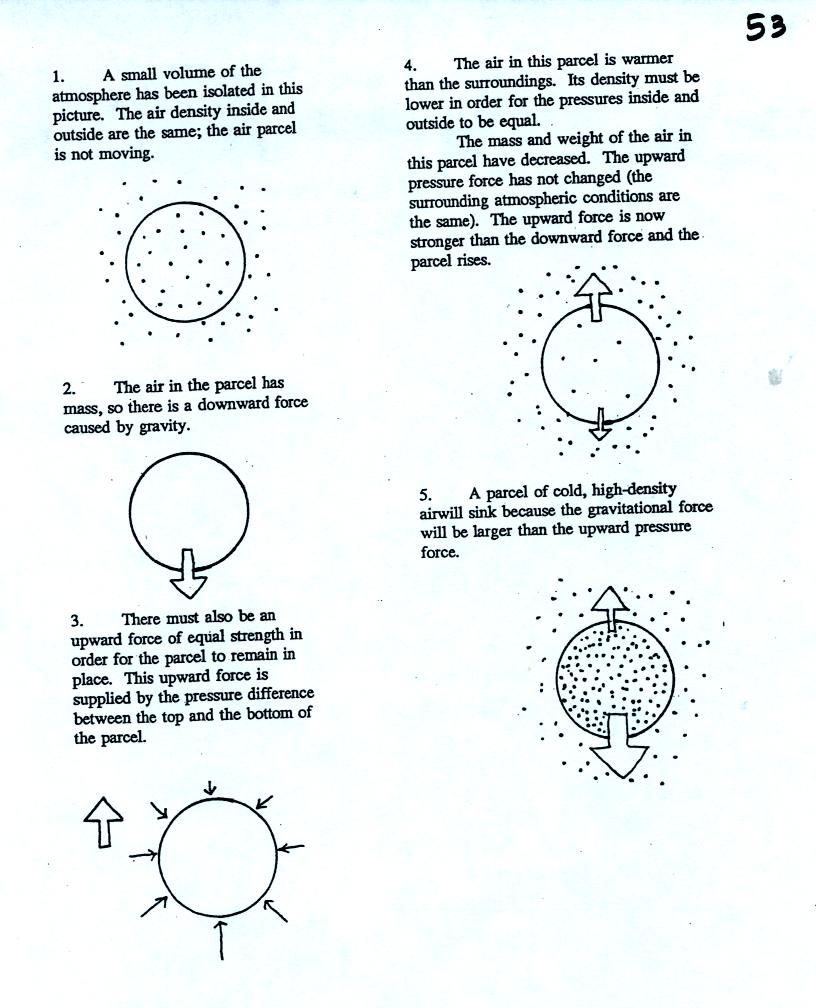
Air has mass and weight When an air parcel has
the same
temperature, pressure, and density as the air around it, the parcel
will remain stationary (top picture below)
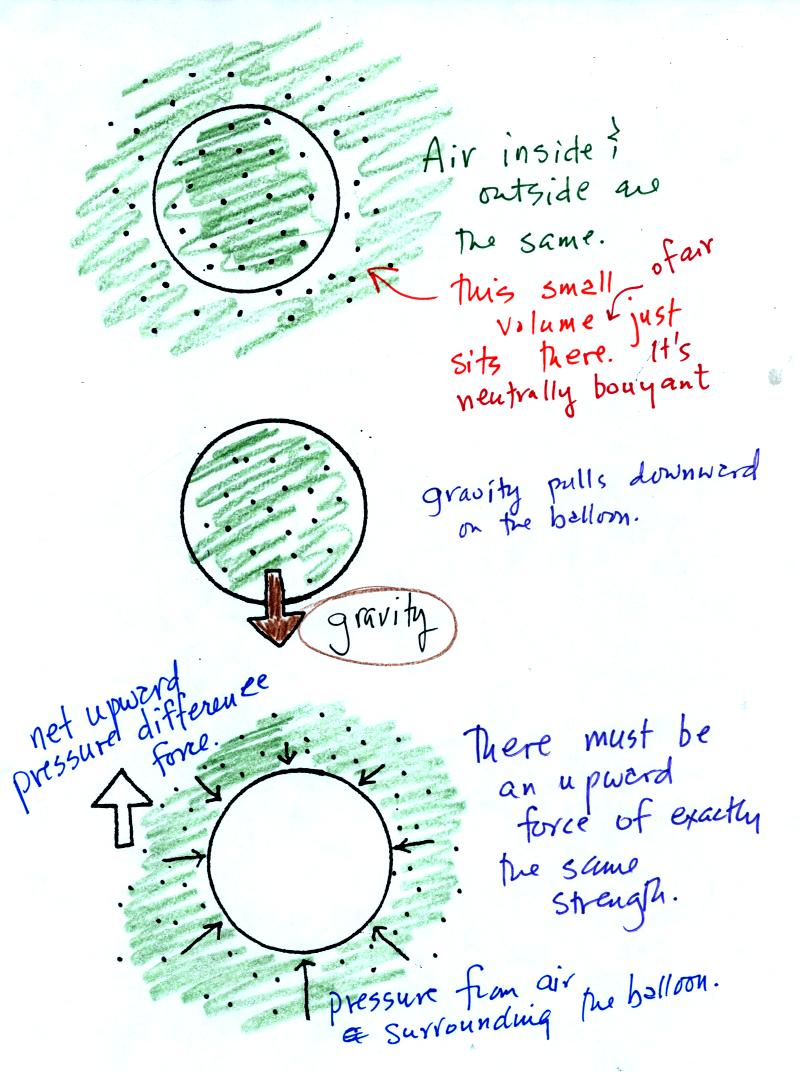
Gravity does pull down on the air in the balloon (middle figure).
With gravity pulling downward on the air,
there must be another upward-pointing force of equal strength.
The upward force (bottom figure) is caused by pressure differences
between the bottom
(higher pressure pushing up) and top of the balloon (slightly lower
pressure pushing down on the balloon).
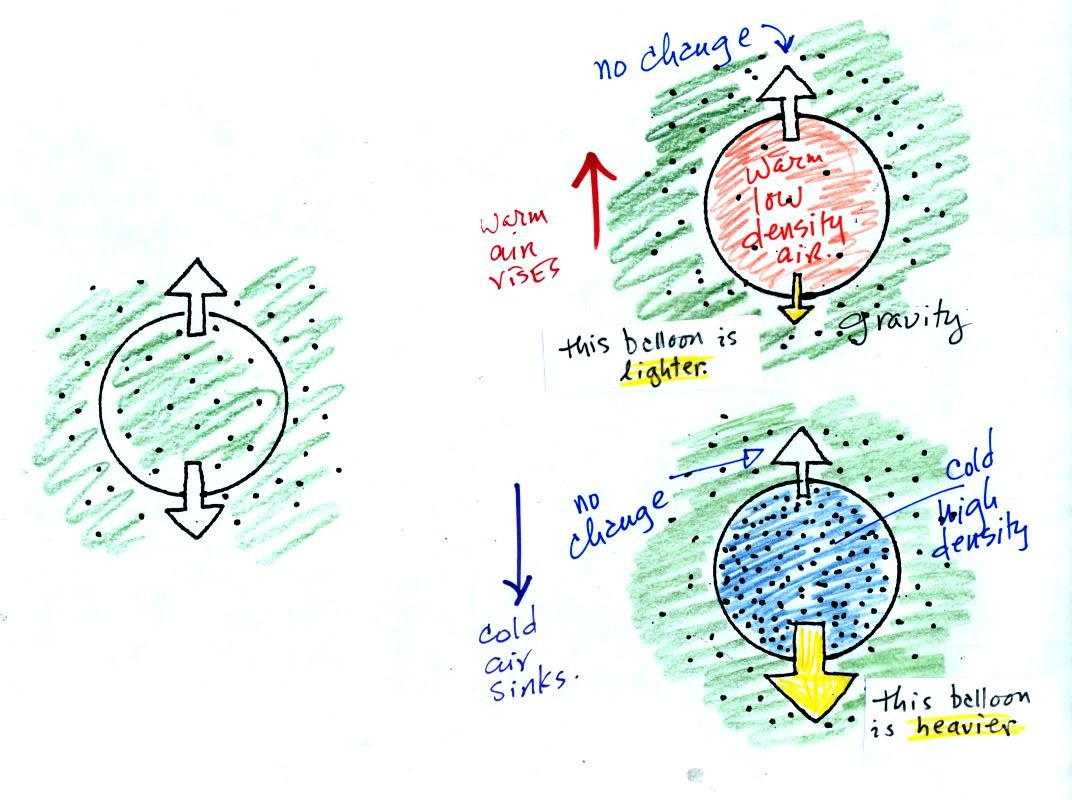
If the balloon is filled with warm, low density air the gravity force
will weaken (there is less air in the balloon so it weighs less). The
upward pressure difference force (which depends on the
surrounding air) will not change. The upward force will be
stronger than the downward force and the balloon will rise.
Conversely if a balloon is filled with cold high density air, the
balloon gets heavier. The upward pressure difference force
doesn't change. The net force is now downward and the balloon
will sink.
Free
convection demonstration
We modified the Charles Law demonstration
(performed
in class last Friday). We used a balloon filled with
helium
instead of air (see bottom of p. 54 in the photocopied Class
Notes). Helium is less dense than air even when the
helium has the same temperature as the surrounding air. A
helium-filled balloon doesn't need to warmed up in order to rise.

We dunked the helium-filled balloon in some liquid nitrogen to cool
it
and to cause the density of the helium to increase. When
removed
from the liquid nitrogen the balloon didn't rise, the gas inside was
denser than the surrounding air (the purple and blue balloons in the
figure above). As the balloon warms and expands
its density decreases. The balloon at some point has the same
density as the air around it (green above) and is neutrally
bouyant. Eventually the balloon becomes less dense that the
surrounding air (yellow) and floats up to the ceiling.