Tuesday Mar. 20, 2012
click here to download today's notes in a more printer friendly format

Three songs from the Punch Brothers before we attempted to restart the class following Spring Break.  You heard "Sometimes" (here's a live version), "Movement and Location" and "Who's Feeling Young Now?".

I was able to catch up on all the grading over Spring Break.  Quiz #2, the Expt. #1 revised reports, and the 1S1P Surface Weather Map Analyses were all returned today together with a computer generated grade summary.

The 1S1P Bonus Assignment "Causes of the Seasons" report and revisions of the Expt. #2 reports are due on Thursday (Mar. 22).

An in-class assignment was handed out today.  If you would like to download the assignment and turn it in at the beginning of class on Thursday you can earn at least partial credit.

A second, take-home, assignment was also handed out.  You'll need to read the online material about the Controls of Temperature.  The assignment is due at the start of class next Tuesday, Mar. 27.

We spent the majority of the class period on an introduction to the next major topic we will be covering: humidity (moisture in the air).  This topic and the terms that we will be learning and using can be confusing.  That's the reason for this introduction.  We will be mainly be interested in 4 variables:


Our first job will be to figure out what their "jobs" are and what can cause them to change value .  I've included both what I wrote down in class as well as the explanations on p. 83 in the photocopied ClassNotes. 



Mixing ratio tells you how much water vapor is actually in the air.  You can think of it as just a number: when the value is large there's more water vapor in the air than when the value is small.  It's really not a difficult concept to grasp.  Mixing ratio has units of grams of water vapor per kilogram of dry air (the amount of water vapor in grams mixed with a kilogram of dry air).  It's basically the same idea as teaspoons of sugar mixed in a cup of tea.
The value of the mixing ratio won't change unless you add water vapor to or remove water vapor from the air.  Warming the air won't change the mixing ratio.  Cooling the air won't change the mixing ratio (unless the air is cooled below its dew point temperature and water vapor starts to condense, but in that case the condensation means water vapor is being removed from the air).  Since the mixing ratio's job is to tell you how much water vapor is in the air, you don't want it to change unless water vapor is actually added to or removed from the air.

Saturation mixing ratio is just an upper limit to how much water vapor can be found in air, the air's capacity for water vapor.  It's a property of air and depends on the air's temperature; warm air can potentially hold more water vapor than cold air.  It doesn't say anything about how much water vapor is actually in the air (that's the mixing ratio's job).    This variable has the same units: grams of water vapor per kilogram of dry air.  Saturation mixing ratio values for different air temperatures are listed and graphed on p. 86 in the photocopied class notes.


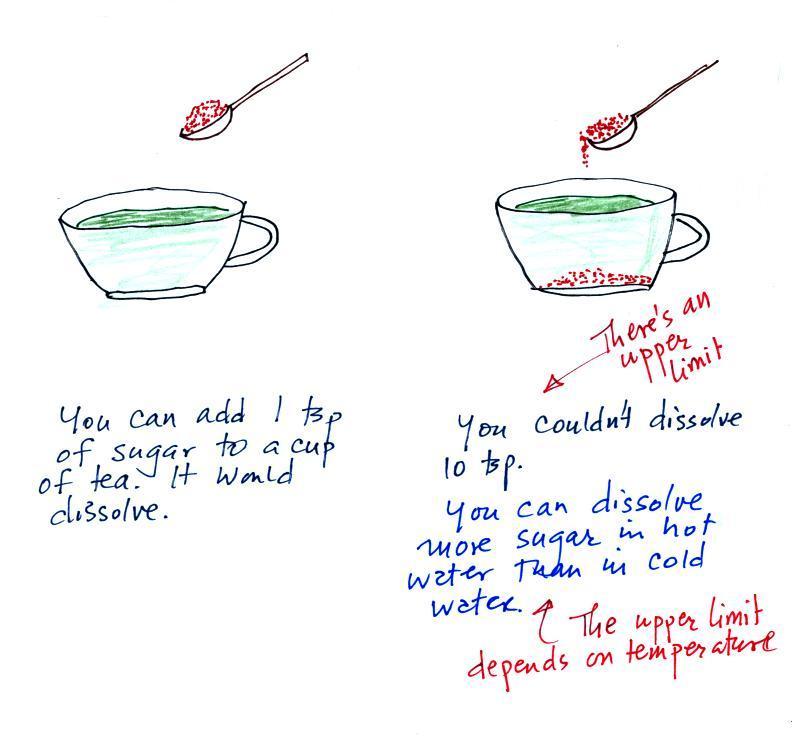
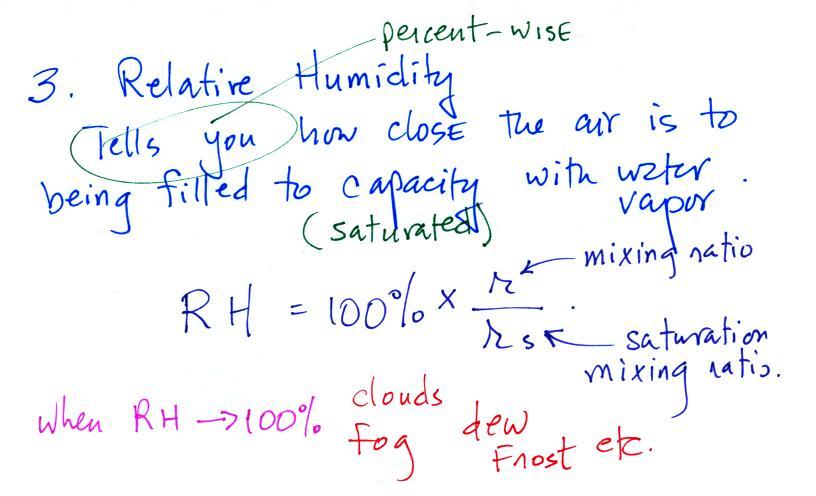
The sugar dissolved in tea analogy is still helpful.  Just as is the case with water vapor in air, there's a limit to how much sugar can be dissolved in a cup of hot water.  You can dissolve more sugar in hot water than in cold water.

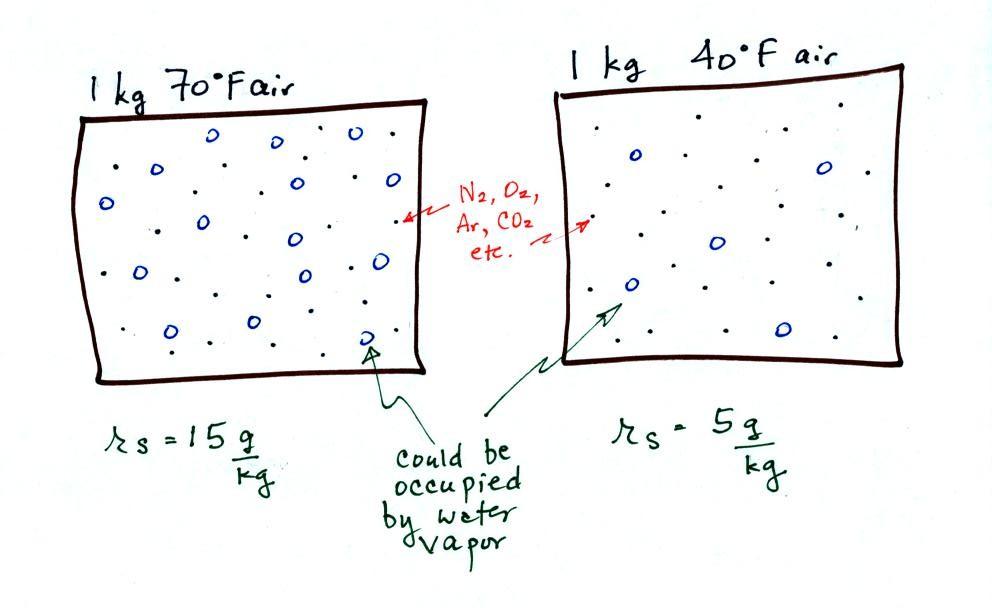
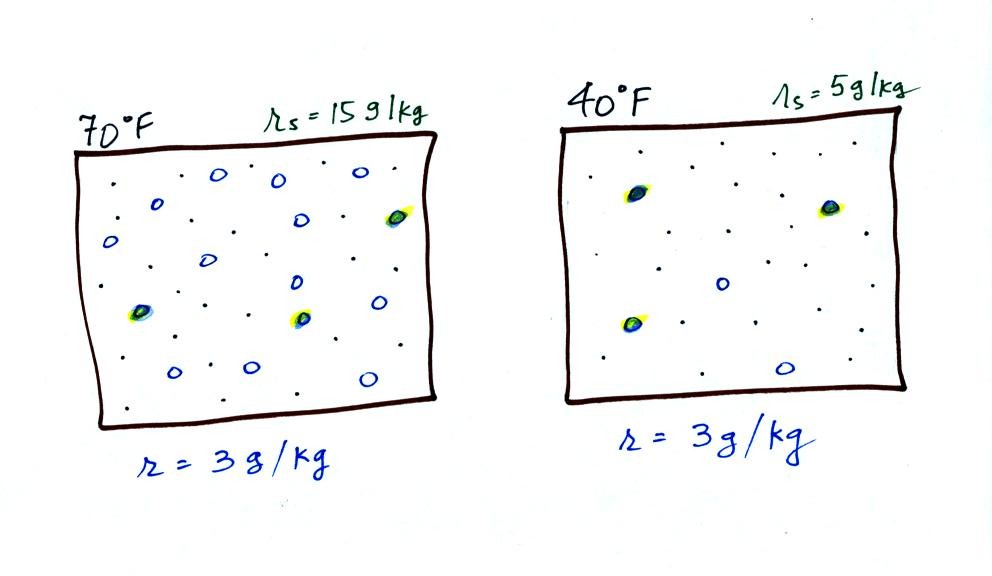
The dependence of saturation mixing ratio on air temperature is illustrated below:


The small specks represent all of the gases in air except for the water vapor.  Each of the open circles represents 1 gram of water vapor that the air could potentially hold.  There are 15 open circles drawn in the 1 kg of 70 F air; each 1 kg of 70 F air could hold up to 15 grams of water vapor.  The 40 F air only has 5 open circles; this cooler air can only hold up to 5 grams of water vapor per kilogram of dry air.  The numbers 15 and 5 came from the table on p. 86.


Now we have gone and actually put some water vapor into the volumes of 70 F and 40 F air (the open circles are colored in).  The same amount, 3 grams of water vapor, has been added to each volume of air.  The mixing ratio, r, is 3 g/kg in both cases.

After looking at the figure above you might start to guess at what relative humidity might mean.


The relative humidity is the variable most people are familiar with.  It tells you how "full" the air is with water vapor, how close it is to being filled to capacity with water vapor.

In the analogy (sketched on the right hand side of p. 83 in the photocopied notes) 4 students wander into Classroom A which has 16 empty seats.  Classroom A is filled to 25% of its capacity.  You can think of 4, the actual number of students, as being analogous to the mixing ratio.  The classroom capacity is analogous to the saturation mixing ratio.  The percentage occupancy is analogous to the relative humidity.

The figure below goes back to the volumes (1 kg each) of 70 F and 40 F air that could potentially hold 15 grams or 5 grams of water vapor.

Both the 70 F and the 40 F air each contain 3 grams of water vapor.  The 70 F air is only filled to 20% of capacity (3 of the 15 open circles is colored in) because this warm air's capacity, the saturation mixing ratio, is large.  The RH in the 40 F is 60% even though it has the same actual amount of water vapor because the 40 F air can't hold as much water vapor and is closer to being saturated. 

Something important to note: RH doesn't really tell you how much water vapor is actually in the air.  The two volumes of air above contain the same amount of water vapor (3 grams per kilogram) but have very different relative humidities.  You could just as easily have two volumes of air with the same relative humidities but different actual amounts of water vapor.


The dew point temperature has two jobs.  First it gives you an idea of the actual amount of water vapor in the air.  In this respect it is just like the mixing ratio.  If the dew point temperature is low the air doesn't contain much water vapor.  If it is high the air contains more water vapor. 

Second the dew point tells you how much you must cool the air in order to cause the RH to increase to 100% (at which point a cloud, or dew or frost, or fog would form).

If we cool the 70 F air or the 40 F air to 30 F we would find that the saturation mixing ratio would decrease to 3 grams/kilogram.  Since the air actually contains 3 g/kg, the RH of the 30 F air would become 100%.  The 30 F air would be saturated, it would be filled to capacity with water vapor.  30 F is the dew point temperature for 70 F air that contains 3 grams of water vapor per kilogram of dry air.  It is also the dew point temperature for 40 F air that contains 3 grams of water vapor per kilogram of dry air.Because both volumes of air had the same amount of water vapor, they both also have the same dew point temperature.
Now back to the student/classroom analogy


The 4 students move into classrooms of smaller and smaller capacity.  The decreasing capacity of the  classrooms is analogous to the decrease in saturation mixing ratio that occurs when you cool air.  Eventually the students move into a classroom that they just fill to capacity. This is analogous to cooling the air to the dew point.
One of the topics we will be covering in a week or so is formation of precipitation and types of precipitation.  Precipitation was falling from the sky yesterday, maybe you saw some of it (the we below should have been were)

Some of what fell was graupel.  That's not quite the same thing as hail (though it's sometimes called soft hail and often is mistaken for hail), snow, or sleet.  I wanted to mention this because that was probably your only chance to see graupel here in Tucson this semester.

Here is some video from KVOA TV4 and a still photograph of graupel from KOLD TV13 in Tucson.

Here's a midterm grade summary example (numbers in the example are class averages)


1.    You should find your two quiz scores here.  The quiz percentage grades are used to compute your overall grade; all the quizzes have the same weight.  There are two more quizzes between now and the last day of classes.

2.    This is the total number of extra credit points you have earned on the Optional Assignments.  You could have earned up to 1.35 pts at this point in the semester.  By the end of the semester that total will be at least 3 pts and maybe a little more.

3.    If you have turned in an experiment or book report and it has been graded you should see the score here.  If there is a 0 here, an average grade of 33 out of 40 has been used by the computer to show the effect of the experiment report on your overall grade.  The Expt. #1 and Expt. #2 reports have been graded.  If you haven't done an experiment or aren't currently working on an experiment you should check with me.

4.    This shows the total number of 1S1P pts you have earned so far (the computer in this case uses a 0 in the calculation if you haven't done any 1S1P reports).  This total doesn't include any reports on current topics that you may have turned in early.  You should try to earn 45 1S1P pts by the end of the semester.  There are several topics currently available that you can write about.

The writing percentage grade is based on both the experiment report grade and the 1S1P pts total.  It takes into account the fact that you couldn't have earned all of the 45 1S1P pts at this point in the semester.

5.    This is the average that needs to be 90.0% or above in order for you to not have to take the final exam.  Note the extra credit points are added on to the average of the quiz and writing scores.

6.    This is the average with the lowest quiz score dropped.  This is the grade that would be used together with your Final Exam score to determine your overall grade.

These mid term grade estimates try to give you an idea of the grade you would receive at the end of the semester if you continue to perform as you have done so far.  It is possible for you to significantly raise your grade between now and the end of the semester.  It is also possible, of course, for your grade to drop. 

Please check your grade summary carefully for errors or omissions.

Here's the final topic of the day




The first thing we need to realize is that warm water will evaporate more rapidly than cool water.  You probably know that already. 

If a cup of iced tea were set next to a cup of hot tea you probably be able to tell which was which by just looking at them.  You wouldn't need to touch or taste the tea or look for ice cubes in the iced tea.

You might notice that one of the cups of tea was steaming (the cup on the right above).  This would be the hot tea.  You're not actually seeing water vapor.  Rather water vapor is evaporating so quickly that it is saturating the air above.  The air isn't able to accomodate that much water vapor and some of it condenses and forms a cloud of steam.  That's what you are able to see.

Now we'll redraw the picture and cover both cups so that water vapor can begin to buildup in the air above the water in both cups. 


Arrows represent the different rates of evaporation.  One arrow is shown evaporating from the cup of cold water.  The warmer water at right is evaporating 3 times more rapidly.  We've arbitrarily assigned rates of evaporation of 10 and 30 to the water in the two cups.

Water vapor will start to buildup in the air above each cup.  And, even though it has just evaporated, some of the water vapor will condense and rejoin the water at the bottom of each cup.  Let's just assume that 1% of the water vapor molecules will condense. 

The water vapor concentration in each glass will increase until it reaches a point where

water evaporation rate = water vapor condensation rate

for the cup of cold water
10 = 0.01 x water vapor concentration
The 0.01 is 1% expressed in decimal form.  Solving this equation gives you a water vapor concentration of 1000.  The air is saturated when you reach this point and the RH = 100%.  Because the rates of condensation and evaporation are equal there won't be any further change in the water vapor concentration.  If you tried to add additional water vapor to the covered glass, the excess would condense and you'd end up back at the original concentration.


The saturation water vapor concentration in the air in the warm cup would be 3000.  And again the relative humidity would be 100%.

The fact that the rates of evaporation and condensation are equal when air is saturated (RH = 100%) is something we'll be using later when we study the formation of precipitation.  Here's a picture of how that would look inside a cloud.

The air inside the cloud is saturated.  The rate of evaporation from the cloud droplet (2 green arrows) is balanced by an equal rate of condensation (2 orange arrows).  The RH = 100%.  The cloud droplet won't grow any bigger or get any smaller.

Here's something to test your understanding of this material.
What information can you add to this picture?  Is the water in one of the glasses warmer than the other?  Is there more water vapor in the air in one of the glasses than in the other?  Is the relative humidity in each glass more than 100%, less than 100% or is it equal to 100%.  The rates of evaporation and condensation aren't equal in either glass, so the pictures will change with time.  What will the glasses look once they have reached equilibrium?  Think about this for a while and then click here for the answers and some explanation.