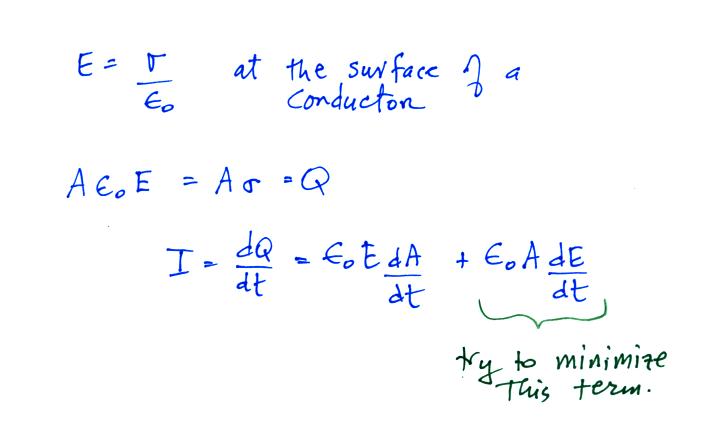Lecture 4 - Review of electrostatics pt. 2

We'll insert this into the expression for electric field.

It is often much simpler to determine the electrostatic potential because it is a scalar quantity. The electric field can then be determined by taking the gradient of the potential.

The expression above is valid for a point charge. More general expressions for cases where multiple charges are present or when charge is distributed over a volume or on a surface are shown below.

Essentially you can break a more complex charge distribution into smaller pieces and then either sum over a collection of multiple discrete charges, or integrate over volume or surface distributions of charge to determine the electrostatic potential.

There may be situations where E is known. We can determine the potential as shown above.

Let's assume a point charge and substitute in an expression for E into the left integral above.

We'll set this equal to the earlier expression

We get our earlier result (provided we assume that Φ(r = ∞) is zero)

We can write the electric field as the gradient of the electrostatic potential and then substitute that into Gauss' Law.

We obtain Poisson's Equation. Laplace's equation applies in situtations where the volume space charge density is zero. We'll be using Laplace's equation in our next lecture. Here is a handout with vector differential operators (Laplacian, curl, gradient and divergence) in cartesian, cylindrical, and spherical coordinate systems.

Now some applications of what we have been learning. In this and the next class we'll looking at a couple of instruments used to measure thunderstorm and lightning electric fields.

The first is an electric field mill used to measure static and slowly time varying electric fields. Referring to the figure below at left (from Uman's 1987 The Lightning Discharge book). The sensors (referred to as studs in the figure) are covered by a rotating grounded plate. The rotating plate is notched or slotted so that the sensors are periodically exposed to and covered (shielded) from the ambient electric field. A photograph of the field mill shown in class is shown below at right (signal and power cables are connected at the bottom of the mill).

The two photographs below are closeups of the top of the field mill

The stator plates are exposed to the E field at left and covered in the photograph at right.

The next figure shows currents flowing into and out of the sensor plate in response to an incident E field.

The sensor plate is covered at Point 1. At Point 2 the sensor is uncovered and we assume the ambient field points upward (toward negative charge in the lower part of a thunderstorm perhaps). Positive charge flows up to the sensor plate. The current flows from the sensor in Point 3 because the sensor has been covered and shielded from the E field. Points 4 and 5 are similar except the polarity of the E field has been changed.

Note the current signals at Points 2 & 5 are the same even though the field polarities are reversed. You must keep track of when the sensor is covered and uncovered in order to determine the polarity of the incident E field.

It is a relatively simple matter to relate the amplitude of the signal current to the intensity of the incident E field.

We use the expression derived a few days ago relating the E field at the surface of a conductor and the surface charge density (sigma in the equations above). A is the area of the sensor.

If you integrate the current (connect the sensor through a capacitor to ground) you obtain an output voltage that is proportional to E.

Next we looked at some typical E field records obtained with an electric field mill. The data come from the Kennedy Space Center field mill network.

The first record is interesting because it shows the transition from fair to foul or stormy weather electric fields (a change in polarity and in field strength).

At the very beginning of the record fields are about 200 V/m. The field crosses zero at about 20:39:00 GMT and increase in amplitude, eventually reaching about -2500 V/m. The abrupt transitions are caused by lightning. In our next lecture we will expand the time scale and look at the field variations that occur in an individual discharge. Later in the course we'll come back to field records like this and show what we start to learn something about the locations of charge and amounts of charge involved in lightning discharges by analyzing these electric field changes.

Note that the vertical axis is labeled potential gradient rather than electric field. This brings up a confusing situation regarding electric field polarities that you should be aware of; something that might cause some confusion if you ever read through some of the atmospheric electricity literature.

The figure above at left correctly show the E field pointing downward toward negative charge on the earth's surface during fair weather. The E field reverses direction under a thunderstorm. The main negative charge center in the cloud causes positive charge to build up in the ground under the storm. The E field points upward.

A physicist would consider the fair weather field to be negative polarity because it points downward and would call the stormy weather field positive. Someone from the atmospheric electricity community might refer to the fair weather field as positive and would call the foul weather field negative. This is a source of confusion.

Nowadays atmospheric electricians either just simply use the physics convention or refer to the potential gradient rather than the electric field. In fair weather a negative E field (physics convention) and a positive potential gradient are consistent.