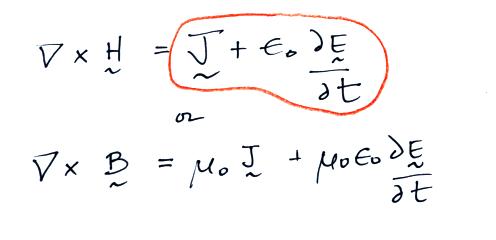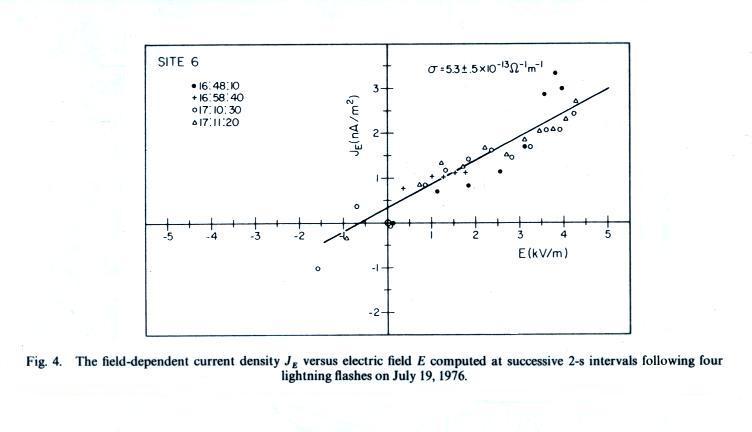
Estimates of conductivity ranged
from 2 to 6 x 10-13 mhos/m.
Actual measurements of conductivity
ranged from 0.4 to 1.8 x 10-14 mhos/m. The
agreement is not very
good. This idea didn't quite pan out. The researchers that
conducted the experiment concluded the estimates of conductivity "will
be
extremely sensitive to small time variations in the local Maxwell
current density and must be modified to include these terms".