The main
goal of today's class is to understand why warm air rises and cold air
sinks.

Hot air balloons rise (they also
sink), so does the relatively
warm air in a thunderstorm (its warmer than the air around
it). Conversely cold air sinks. The surface winds
caused by a thunderstorm downdraft (as shown above) can reach speeds of
100 MPH and are a serious weather hazard.
A full understanding of this is a 3-step process (the following is from the bottom part of p. 49 in the photocopied ClassNotes)
A full understanding of this is a 3-step process (the following is from the bottom part of p. 49 in the photocopied ClassNotes)

We will first learn about the ideal
gas law.
That is an equation that tells you which/how properties of the air
inside a
balloon work to determine the air's pressure. Then we will look
at Charles' Law, a special situation involving the ideal gas law (air
temperature and density change together in a way that keeps the
pressure
inside a balloon constant).
Then we'll learn about the vertical forces that act on air (an upward
and a downward force)
The figure above makes an important point: the air molecules in a balloon "filled with air" really take up very little space. A balloon filled with air is really mostly empty space. It is the collisions of the air molecules with the inside walls of the balloon that keep it inflated.
The figure above makes an important point: the air molecules in a balloon "filled with air" really take up very little space. A balloon filled with air is really mostly empty space. It is the collisions of the air molecules with the inside walls of the balloon that keep it inflated.

Up to this point in the semester we
have been thinking of pressure as
being determined
by the weight of the air overhead. Air pressure pushes down
against the ground at sea level with 14.7 pounds of force per square
inch. If you imagine the weight of the atmosphere pushing down on
a balloon sitting on the ground you realize that the air in the balloon
pushes back with the same force. Air everywhere in the atmosphere
pushes upwards, downwards, and sideways.
The ideal gas law equation is another way of thinking about air pressure, sort of a microscope . We ignore the atmosphere and concentrate on just the air inside the balloon. We are going to "derive" an equation. Pressure (P) will be on the left hand side. Properties of the air inside the balloon will be found on the right side of the equation.
The ideal gas law equation is another way of thinking about air pressure, sort of a microscope . We ignore the atmosphere and concentrate on just the air inside the balloon. We are going to "derive" an equation. Pressure (P) will be on the left hand side. Properties of the air inside the balloon will be found on the right side of the equation.


In A
the pressure produced by
the air
molecules inside a balloon will
first depend on how many air molecules are there, N. If there
weren't any air molecules at all there wouldn't be any
pressure. As you add more and more add to something like a
bicycle tire, the
pressure increases. Pressure is directly proportional to N - an
increase in N causes an increase in P. If N doubles, P also
doubles (as long as the other variables in the equation don't change).
In B
air pressure inside a balloon also depends on the size of the balloon. Pressure is inversely proportional to volume, V . If V were to double, P would drop to 1/2 its original value.
Note
it is possible to keep pressure constant by changing N and V together in just the right kind of way. This is what happens in Experiment #1 that some students are working on. Oxygen in a graduated cylinder reacts with steel wool to form rust. Oxygen is removed from the air sample which is a decrease in N. As oxygen is removed, water rises up into the cylinder decreasing the air sample volume. N and V both decrease in the same relative amounts and the air sample pressure remains constant. If you were to remove 20% of the air molecules, V would decrease to 20% of its original value and pressure would stay constant.
In B
air pressure inside a balloon also depends on the size of the balloon. Pressure is inversely proportional to volume, V . If V were to double, P would drop to 1/2 its original value.
Note
it is possible to keep pressure constant by changing N and V together in just the right kind of way. This is what happens in Experiment #1 that some students are working on. Oxygen in a graduated cylinder reacts with steel wool to form rust. Oxygen is removed from the air sample which is a decrease in N. As oxygen is removed, water rises up into the cylinder decreasing the air sample volume. N and V both decrease in the same relative amounts and the air sample pressure remains constant. If you were to remove 20% of the air molecules, V would decrease to 20% of its original value and pressure would stay constant.

Part C: Increasing
the temperature of the gas in a balloon will cause the gas molecules to
move more quickly. They'll collide with the walls of the balloon
more frequently and rebound with greater force. Both will
increase the pressure. You shouldn't throw a can of spray paint
into a fire because the temperature will cause the pressure inside the
can to increase and the can could explode. We'll demonstrate the
effect of temperature on pressure in class on Friday.
Surprisingly, as explained in Part D, the pressure does not depend on the mass of the molecules. Pressure doesn't depend on the composition of the gas. Gas molecules with a lot of mass will move slowly, the less massive molecules will move more quickly. They both will collide with the walls of the container with the same force.
The figure below (which replaces the bottom of p. 51 in the photocopied ClassNotes) shows two forms of the ideal gas law. The top equation is the one we just derived and the bottom is a second slightly different version. You can ignore the constants k and R if you are just trying to understand how a change in one of the variables would affect the pressure. You only need the constants when you are doing a calculation involving numbers (which we won't be doing).
Surprisingly, as explained in Part D, the pressure does not depend on the mass of the molecules. Pressure doesn't depend on the composition of the gas. Gas molecules with a lot of mass will move slowly, the less massive molecules will move more quickly. They both will collide with the walls of the container with the same force.
The figure below (which replaces the bottom of p. 51 in the photocopied ClassNotes) shows two forms of the ideal gas law. The top equation is the one we just derived and the bottom is a second slightly different version. You can ignore the constants k and R if you are just trying to understand how a change in one of the variables would affect the pressure. You only need the constants when you are doing a calculation involving numbers (which we won't be doing).

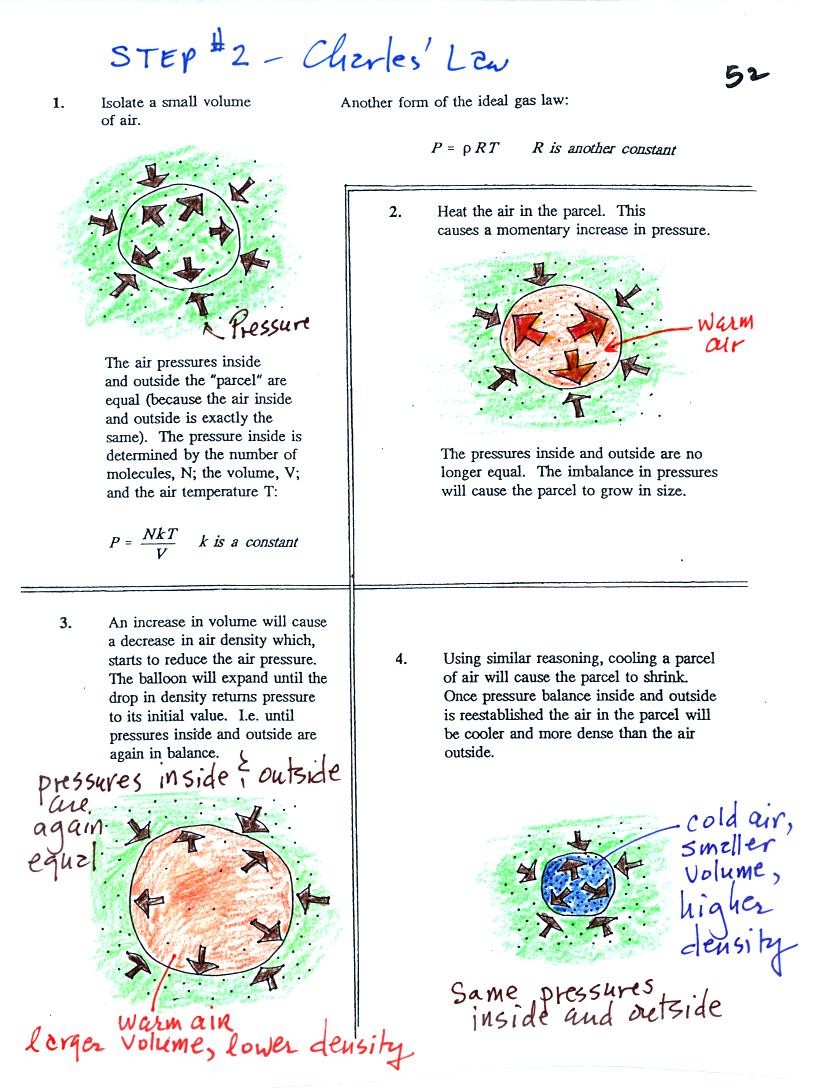
Read through the explanation on p.
52 in the photocopied
Classnotes. In the atmosphere a parcel (balloon) of air will
always try to keep its pressure the same as the pressure of the
surrounding air. If they aren't equal the parcel will either
expand or shrink until they are again equal.
If you warm air it will expand and density will decrease until the pressure inside and outside the parcel are equal.
If you cool air the parcel will shrink and the density will increase until the pressures balance.
These two associations:
If you warm air it will expand and density will decrease until the pressure inside and outside the parcel are equal.
If you cool air the parcel will shrink and the density will increase until the pressures balance.
These two associations:
(i)
warm air = low
density air
(ii) cold air = high density air
(ii) cold air = high density air
are important and will come up a
lot during the remainder of the
semester.
Click here
if you
would like a little
more detailed, more step-by-step,
explanation of Charles Law. Otherwise continue on and read a
summary of the Charles'
Law demonstration that we did in class.
Charles Law can be demonstrated by dipping a balloon in liquid nitrogen. You'll find an explanation on the top of p. 54 in the photocopied ClassNotes.

The balloon had shrunk down to
practically zero volume when
pulled from the liquid nitrogen. It was filled with cold high
density air. As
the balloon warmed the balloon expanded and the density of the air
inside
the balloon decreased. The volume and temperature kept changing
in a way that kept pressure constant. Eventually the balloon ends
up back at room temperature (unless it pops).
Now
finally on to step #3. It's found on p. 53 in the photocopied
ClassNotes.

Basically what it comes down to is
this - there are two forces
acting on a parcel (balloon) of air:
Gravity pulls downward. The strength of the gravity force depends on the mass of the air inside the balloon.
There is an upward pointing pressure difference force. This is caused by the air surrounding the balloon.
When the air inside a parcel is exactly the same as the air outside, the two forces have equal strengths and cancel out. The parcel is neutrally bouyant and doesn't rise or sink.
If you replace the air inside the balloon with warm low density air, it won't weigh as much. The gravity force is weaker. The upward pressure difference force doesn't change and ends up stronger than the gravity force. The balloon will rise.
Conversely if the air inside is cold high density air, it weighs more. Gravity is stronger than the upward pressure difference force and the balloon sinks.
Gravity pulls downward. The strength of the gravity force depends on the mass of the air inside the balloon.
There is an upward pointing pressure difference force. This is caused by the air surrounding the balloon.
When the air inside a parcel is exactly the same as the air outside, the two forces have equal strengths and cancel out. The parcel is neutrally bouyant and doesn't rise or sink.
If you replace the air inside the balloon with warm low density air, it won't weigh as much. The gravity force is weaker. The upward pressure difference force doesn't change and ends up stronger than the gravity force. The balloon will rise.
Conversely if the air inside is cold high density air, it weighs more. Gravity is stronger than the upward pressure difference force and the balloon sinks.
We used
balloons filled with hydrogen instead of air (see bottom of p. 54 in
the photocopied Class
Notes). Hydrogen is less dense than air even when the
hydrogen has the same temperature as the surrounding air. A
hydrogen-filled balloon doesn't need to warmed up in order to rise.

We dunked the hydrogen-filled balloon
in some liquid nitrogen to cool
it
and to cause the density of the hydrogen to increase. When
removed
from the liquid nitrogen the balloon didn't rise, the gas inside was
denser than the surrounding air (the purple and blue balloons in the
figure above). As the balloon warms and expands
its density decreases. The balloon at some point has the same
density as the air around it (green above) and is neutrally
bouyant. Eventually the balloon becomes less dense that the
surrounding air (yellow) and floats up to the ceiling.
Here's an example of where something like this happens in the atmosphere (this figure wasn't shown in class)
Here's an example of where something like this happens in the atmosphere (this figure wasn't shown in class)

At (1) sunlight reaching the ground is absorbed and warms the
ground. This in turns warms air in contact with the ground
(2). Once this air becomes warm and its density is low enough,
small "blobs" of air separate from the air layer at the ground and
begin
to rise (3). Rising air expands and cools (we've haven't covered
this yet). If it cools enough (to the dew point) a cloud will
become visible as shown at Point 4. This whole process is called
free convection; many of our summer
thunderstorms start this way.
Here's some extra material that we didn't have time for in class, something that I promised to add to today's notes, We'll come back to this briefly on Friday. I have a really colorful demonstration that goes along with this topic.
We've just seen how the relative strengths of the downward graviational force and the upward pressure difference force determine whether a parcel of air will rise or sink. Archimedes Law is another way of trying to understand the situation.

A gallon of water weighs about 8 pounds (lbs).
If you submerge a 1 gallon jug of water in a swimming pool, the jug becomes, for all intents and purposes, weightless. Archimedes' Law (see figure below, from p. 53a in the photocopied ClassNotes) explains why this is true.

The upward bouyant force is really just another name for the
pressure difference force covered earlier today (higher pressure
pushing
up on the bottle and low pressure at the top pushing down, resulting in
a net upward force). A 1 gallon bottle will displace 1 gallon of
pool water. One
gallon of pool
water weighs 8 pounds. The upward bouyant force will be 8 pounds,
the same as the downward force on the jug due to gravity. The two
forces are equal and opposite.
Now we imagine pouring out all the water and filling the 1 gallon jug with air. Air is about 1000 times less dense than water;compared to water, the jug will weigh practically nothing.

If you submerge the jug in a pool it will displace 1 gallon of
water
and experience an 8 pound upward bouyant force again. Since there
is no downward force the jug will float.
One gallon of sand (which is about 1.5 times denser than water) jug will weigh 12 pounds.

The jug of sand will sink because the downward force is greater
than
the upward force.
You can sum all of this up by saying anything that is less dense than water will float in water, anything that is more dense than water will float in water.
The same reasoning applies to air in the atmosphere.

Air that is less dense (warmer) than the air around it will
rise.
Air that is more dense (colder) than the air around it will sink.
Here's a little more information about Archimedes.
I might also has said something about adding a couple of pages of notes about Newton's Law of Universal Gravitation. It sounded like there might be some extra credit involved somehow, so here are the notes (from pps 27 & 28 in the photocopied ClassNotes).
Newton's Law of Universal Gravitation is an equation that allows you to calculate the gravitational attraction between two objects. Don't worry about this topic. I'm including it just so that you can see that with a little thought you can appreciate and understand why certain variables appear in Newton's Law and why they appear in either the numerator (direct proportionality) or in the denominator (inverse proportionality).

The gravitational attraction between two objects (M and m in the figures) depends first of all on the distance separating the objects. The gravitational force becomes weaker the further away the two objects are from each other. In the bottom picture above and the top figure below we see that the attractive force also depends on the masses of the two objects.

Here's some extra material that we didn't have time for in class, something that I promised to add to today's notes, We'll come back to this briefly on Friday. I have a really colorful demonstration that goes along with this topic.
We've just seen how the relative strengths of the downward graviational force and the upward pressure difference force determine whether a parcel of air will rise or sink. Archimedes Law is another way of trying to understand the situation.

If you submerge a 1 gallon jug of water in a swimming pool, the jug becomes, for all intents and purposes, weightless. Archimedes' Law (see figure below, from p. 53a in the photocopied ClassNotes) explains why this is true.

Now we imagine pouring out all the water and filling the 1 gallon jug with air. Air is about 1000 times less dense than water;compared to water, the jug will weigh practically nothing.

One gallon of sand (which is about 1.5 times denser than water) jug will weigh 12 pounds.

You can sum all of this up by saying anything that is less dense than water will float in water, anything that is more dense than water will float in water.
The same reasoning applies to air in the atmosphere.

Here's a little more information about Archimedes.
I might also has said something about adding a couple of pages of notes about Newton's Law of Universal Gravitation. It sounded like there might be some extra credit involved somehow, so here are the notes (from pps 27 & 28 in the photocopied ClassNotes).
Newton's Law of Universal Gravitation is an equation that allows you to calculate the gravitational attraction between two objects. Don't worry about this topic. I'm including it just so that you can see that with a little thought you can appreciate and understand why certain variables appear in Newton's Law and why they appear in either the numerator (direct proportionality) or in the denominator (inverse proportionality).

The gravitational attraction between two objects (M and m in the figures) depends first of all on the distance separating the objects. The gravitational force becomes weaker the further away the two objects are from each other. In the bottom picture above and the top figure below we see that the attractive force also depends on the masses of the two objects.
